Giải hệ phương trình\(\left\{{}\begin{matrix}x-\sqrt{3y+1}=2\\\sqrt{3y+1}+4=3\sqrt{\left(x-2y\right)\left(y+1\right)}\end{matrix}\right.\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
2 tháng 2 2021
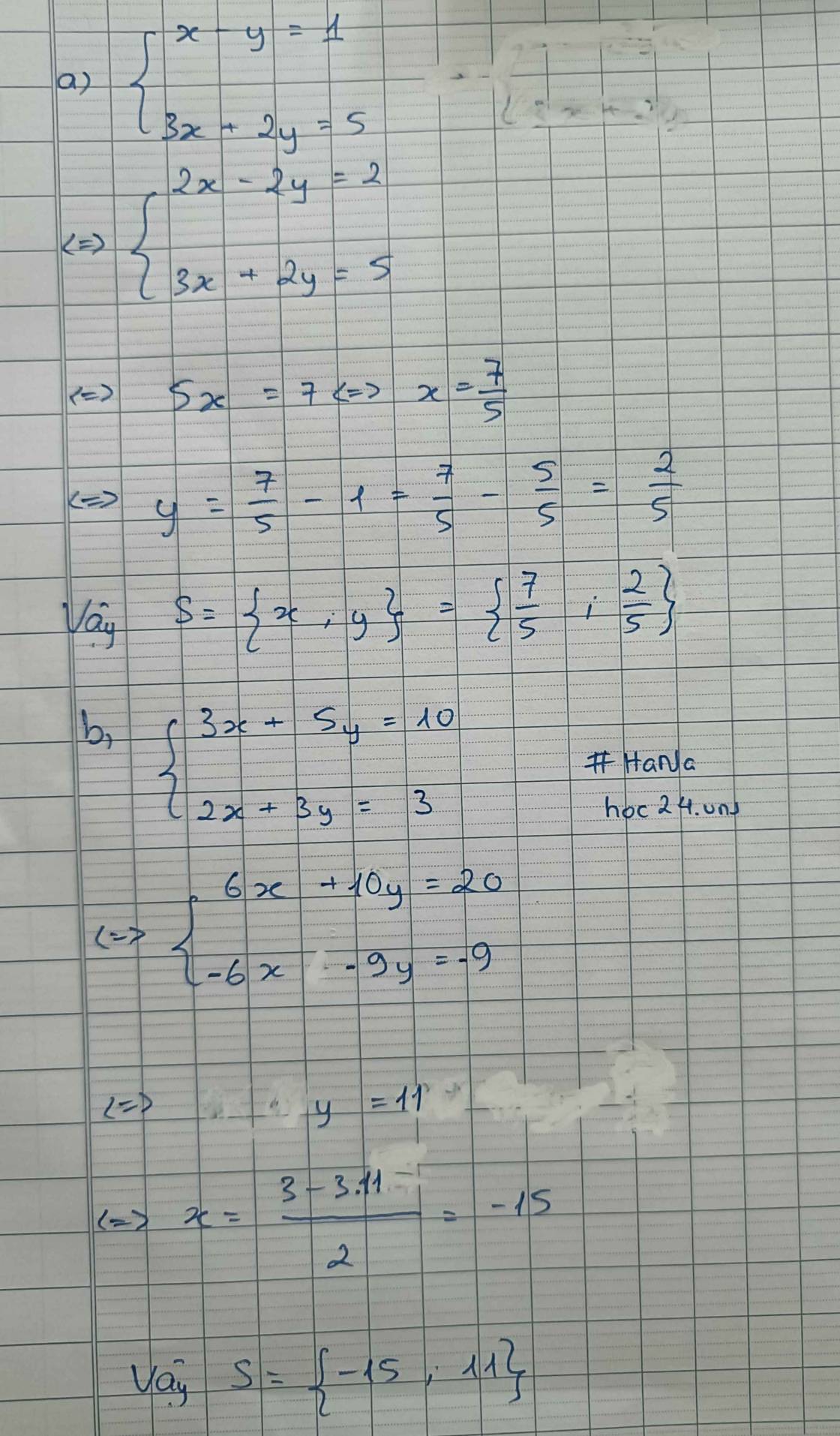
\(\left\{{}\begin{matrix}\dfrac{x+2}{y-1}=\dfrac{x-4}{y+2}\\\dfrac{2x+3}{y-1}=\dfrac{4x+1}{2y+1}\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}\left(x+2\right)\left(y+2\right)=\left(y-1\right)\left(x-\text{4}\right)\\\left(2x+3\right)\left(2y+1\right)=\left(y-1\right)\left(4x+1\right)\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}xy+2x+2y+4=xy-4y-x+4\\4xy+2x+6y+3=4xy-4x+y-1\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}3x+6y=0\\6x+5y=-4\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}x=-\dfrac{8}{7}\\y=\dfrac{4}{7}\end{matrix}\right.\)(TM)
2 tháng 2 2021
\(\left\{{}\begin{matrix}5\left(x-y\right)-3\left(2x+3y\right)=12\\3\left(x+2y\right)-4\left(x+2y\right)=5\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}5x-5y-6x-9y=12\\3x+6y-4x-8y=5\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}-x-14y=12\\-x-2y=5\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}x=-\dfrac{26}{3}\\y=-\dfrac{7}{12}\end{matrix}\right.\)
Vậy HPT có nghiệm (x;y) = (\(-\dfrac{26}{3};-\dfrac{7}{12}\))
25 tháng 4 2017
a. ĐK: \(x\ge1;y\ge1\)
Đặt \(\sqrt{x-1}=a\left(a\ge0\right)\) và \(\sqrt{y-1}=b\left(b\ge0\right)\)
Khí đó hệ phương trình trở thành:
\(\left\{{}\begin{matrix}2a-b=1\\a+b=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}b=2a-1\\a+2a-1=2\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}b=2.1-1\\a=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}b=1\\a=1\end{matrix}\right.\)(tm)
* a = 1 \(\Leftrightarrow\sqrt{x-1}=1\Leftrightarrow x-1=1\Leftrightarrow x=2\)(tmđk)
* b = 1 \(\sqrt{y-1}=1\Leftrightarrow y-1=1\Leftrightarrow y=2\) (tmđk)
Vậy nghiệm của hệ phương trình là (2;2)
25 tháng 4 2017
b. Đặt \(\left(x-1\right)^2=a\) ( a \(\ge\) 0)
Khi đó hệ phương trình đã cho trở thành :
\(\left\{{}\begin{matrix}a-2y=2\\3a+3y=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=2+2y\\3\left(2+2y\right)+3y=1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}a=2+2.\left(-\dfrac{5}{9}\right)\\y=-\dfrac{5}{9}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}a=\dfrac{8}{9}\\y=-\dfrac{5}{9}\end{matrix}\right.\)(tmđk)
* a = \(\dfrac{8}{9}\Leftrightarrow\) \(\left(x-1\right)^2=\dfrac{8}{9}=\left(\pm\dfrac{2\sqrt{2}}{3}\right)^2\) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{2\sqrt{2}}{3}+1\\x=-\dfrac{2\sqrt{2}}{3}+1\end{matrix}\right.\)
Vậy nghiệm của hệ phương trình là \(\left(\dfrac{2\sqrt{2}}{3};-\dfrac{5}{9}\right);\left(\dfrac{-2\sqrt{2}}{3};-\dfrac{5}{9}\right)\)
26 tháng 1
a: ĐKXĐ: y<=1/2
\(\left\{{}\begin{matrix}3\left(x-1\right)-\sqrt{1-2y}=1\\\left(x-1\right)+2\sqrt{1-2y}=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}6\left(x-1\right)-2\sqrt{1-2y}=2\\\left(x-1\right)+2\sqrt{1-2y}=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}7\left(x-1\right)=7\\\left(x-1\right)+2\sqrt{1-2y}=5\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x-1=1\\2\sqrt{1-2y}=5-1=4\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=2\\\sqrt{1-2y}=2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=2\\1-2y=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\y=-\dfrac{3}{2}\left(nhận\right)\end{matrix}\right.\)
b:
ĐKXĐ: \(x\in R\)
\(\left\{{}\begin{matrix}\sqrt{x^2-2x+1}-3y=7\\2\left|x-1\right|-8y=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\sqrt{\left(x-1\right)^2}-3y=7\\2\left|x-1\right|-8y=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\left|x-1\right|-3y=7\\2\left|x-1\right|-8y=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2\left|x-1\right|-6y=14\\2\left|x-1\right|-8y=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}2y=13\\\left|x-1\right|-3y=7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=\dfrac{13}{2}\\\left|x-1\right|=3y+7=3\cdot\dfrac{13}{2}+7=\dfrac{39}{2}+7=\dfrac{53}{2}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=\dfrac{13}{2}\\x-1\in\left\{\dfrac{53}{2};-\dfrac{53}{2}\right\}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=\dfrac{13}{2}\\x\in\left\{\dfrac{55}{2};-\dfrac{51}{2}\right\}\end{matrix}\right.\)
c: ĐKXĐ: y>=4
\(\left\{{}\begin{matrix}2\left(x^2-x\right)+\sqrt{y-4}=0\\3\left(x^2-x\right)-2\sqrt{y-4}=-7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}4\left(x^2-x\right)+2\sqrt{y-4}=0\\3\left(x^2-x\right)-2\sqrt{y-4}=-7\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}7\left(x^2-x\right)=-7\\2\left(x^2-x\right)+\sqrt{y-4}=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x^2-x=-1\\\sqrt{y-4}=2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x^2-x+1=0\\y-4=4\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}=0\left(vôlý\right)\\y=8\end{matrix}\right.\)
=>\(\left(x,y\right)\in\varnothing\)
29 tháng 3 2019
Điều kiện: $\left\{\begin{matrix} 2y(x+1)\geq 0\\x\geq -3 \\y\geq 1 \\ x^2+x+2y-4\geq 0 \end{matrix}\right.$
$\Leftrightarrow \left\{\begin{matrix} x\geq -1\\ y\geq 1\\x^2+x+2y-4\geq 0 \end{matrix}\right.$
$(1)\Leftrightarrow 2(x+3y+1)\sqrt{2xy+2y}=6xy+8y^2+6y$
$\Leftrightarrow [(x+3y+1)-\sqrt{2xy+2y}]^2-(x+y+1)^2=0$
$\Leftrightarrow (x+3y+1-\sqrt{2xy+2y}-x-y-1)(x+3y+1-\sqrt{2xy+2y}+x+y+1)=0$
$\Leftrightarrow \begin{bmatrix} 2y=\sqrt{2xy+2y} (A)\\ 2x+4y+2=\sqrt{2xy+2y} (B) \end{bmatrix}$
+) iải (A):
(A)<=> $4y^2=2xy+2y$
<=> $\begin{bmatrix} y=0 (loại vì y \geq 1)\\ 2y=x+1 \end{bmatrix}$
thế $2y=x+1$ vào (2) => nhân liên hợp 2 căn được pt: $x-3+\sqrt{x^2+2x-3}=\sqrt{x+3}+\sqrt{x-1}$ => bình phương => rút gọn được pt sau:
$(\sqrt{x^2+2x-3}+x-4)^2=9$ => giải được 2 nghiệm
+) giải (B):
(B) <=> $(\sqrt{2y}-\sqrt{x-1})^2+3(x+2y+1)=0$
Vì $\left\{\begin{matrix} x\geq -1\\ y\geq 1 \end{matrix}\right.$ => pt vô nghiệm