Nếu r(x^2 - 1) - (x^2 - s) = 5x +6 thì r - s = ?
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HQ
Hà Quang Minh
Giáo viên
17 tháng 9 2023
a)
\(\begin{array}{l}R(x) + S(x) = - 8{x^4} + 6{x^3} + 2{x^2} - 5x + 1 + {x^4} - 8{x^3} + 2x + 3\\ = ( - 8 + 1){x^4} + (6 - 8){x^3} + 2{x^2} + ( - 5 + 2)x + (1 + 3)\\ = - 7{x^4} - 2{x^3} + 2x - 3x + 4\end{array}\)
b)
\(\begin{array}{l}R(x) - S(x) = - 8{x^4} + 6{x^3} + 2{x^2} - 5x + 1 - ({x^4} - 8{x^3} + 2x + 3)\\ = - 8{x^4} + 6{x^3} + 2{x^2} - 5x + 1 - {x^4} + 8{x^3} - 2x - 3\\ = ( - 8 - 1){x^4} + (6 + 8){x^3} + 2{x^2} + ( - 5 - 2)x + (1 - 3)\\ = - 9{x^4} + 14{x^3} + 2x - 7x - 2\end{array}\)
TT
1
17 tháng 12 2017
\(S=\dfrac{3x+6}{x^2-4x+4}-\dfrac{5x-16}{x^2+4x+4}\)
\(S=\dfrac{3x+6}{\left(x-2\right)^2}-\dfrac{5x-16}{\left(x+2\right)^2}\)
\(S=\dfrac{3x+6}{\left(x-2\right)^2}-\dfrac{5x-16}{-\left(x-2\right)^2}\)
\(S=\dfrac{3x+6}{\left(x-2\right)^2}-\dfrac{-(5x-16)}{\left(x-2\right)^2}\)
\(S=\dfrac{3x+6}{\left(x-2\right)^2}-\dfrac{-5x+16}{\left(x-2\right)^2}\)
\(S=\dfrac{3x+6-\left(-5x\right)+16}{\left(x-2\right)^2}\)
\(S=\dfrac{3x-\left(-5x\right)+6+16}{\left(x-2\right)^2}\)
\(S=\dfrac{8x+22}{\left(x-2\right)^2}\)
4 tháng 11 2017
Bài 1.
a) Do hai phân thức bằng nhau , ta có :
( x +2)P( x2 - 22) = ( x - 1)Q( x -2)
=( x + 2)P( x - 2)( x + 2) = ( x - 1)Q( x - 2)
Suy ra : P = x - 1 ; Q = ( x + 2)2
b) Do hai phân thức bằng nhau , ta có :
( x + 2)P(x2 - 2x + 1) = ( x - 2)Q( x2 - 1)
= ( x + 2)P( x - 1)2 = ( x - 2)Q( x - 1)( x + 1)
Suy ra : P = ( x - 2)( x + 1) = x2 - x - 2
Q = ( x + 2)( x - 1) = x2 + x + 2
4 tháng 11 2017
Bài 2. a) Do : \(\dfrac{P}{Q}=\dfrac{R}{S}=>PS=QR\)
Xét : ( P + Q)S= PS + QS = QR + QS = Q( R + S)
-> \(\dfrac{P+Q}{Q}=\dfrac{R+S}{S}\)
b) Do : \(\dfrac{P}{Q}=\dfrac{R}{S}=>PS=QR\)
Xét : ( S - R)P = PS - PR = QR - PR = R( Q - P)
-> \(\dfrac{R-S}{R}=\dfrac{Q-P}{P}\)
- > \(\dfrac{R}{R-S}=\dfrac{P}{Q-P}\)
AW
26 tháng 12 2017
a)S=\(\left(\dfrac{x}{x^2-36}-\dfrac{x-6}{x^2+6x}\right):\dfrac{2x-6}{x^2+6x}+\dfrac{x}{6-x}\)
=\(\left(\dfrac{x}{\left(x-6\right)\left(x+6\right)}-\dfrac{x-6}{x\left(x+6\right)}\right):\dfrac{2x-6}{x\left(x+6\right)}+\dfrac{x}{6-x}\)
\(\left(\dfrac{x^2}{x\left(x-6\right)\left(x+6\right)}-\dfrac{\left(x-6\right)^2}{x\left(x-6\right)\left(x+6\right)}\right):\dfrac{2x-6}{x\left(x+6\right)}+\dfrac{x}{6-x}\)
=\(\dfrac{x^2-\left(x-6\right)^2}{x\left(x-6\right)\left(x+6\right)}:\dfrac{2\left(x-3\right)}{x\left(x+6\right)}+\dfrac{x}{6-x}\)
=\(\dfrac{6\left(2x-6\right)x\left(x+6\right)}{x\left(x-6\right)\left(x+6\right)\left(2x-6\right)}+\dfrac{x}{6-x}\)
=\(\dfrac{6}{x-6}+\dfrac{x}{6-x}\)
=\(\dfrac{6}{x-6}-\dfrac{x}{x-6}=\dfrac{6-x}{x-6}=-1\)
b ) S khi rút gọn=-1 => mọi giá trị của x đều thỏa mãn S=-1
CM
19 tháng 5 2019
Đường tròn : C): (x-1)2+ (y-3) 2 = R2 có tâm I( 1;3) bán kính R.
Để đường thẳng d tiếp xúc với đường tròn (C) khi
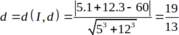
Chọn B.
TH
0
r(x2-1)-(x2-s)=5x+6
=>rx2-r-x2+s=5x+6
=>x2(r-1)-r+s
- Áp dụng phương pháp hệ số bất định ta có:
-r+s=6 =>r-s=-6