Tìm x biết (9/4) :(1/3x - 1/2) = 9/2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
17 tháng 7 2021
(1-3x2)-(x-2)(9x+1)=(3x-4)(3x+4)-9(x+3)2
⇒1-3x2-(9x2+x-18x-2)=9x2-16-9(x2+6x+9)
⇒1-3x2-(9x2-17x-2)= -56x-97
⇒1-3x2-9x2+17x+2=-56x-97
⇒3-12x2+17x=-56x-97
⇒3-12x2+17x+56x+97=0
⇒-12x2+73x+100=0
⇒-(12x2-73x-100)=0
22 tháng 9 2021
a: Ta có: \(\left(x+2\right)\left(x^2-2x+4\right)-x\left(x^2+2\right)=15\)
\(\Leftrightarrow x^3+8-x^3-2x=15\)
\(\Leftrightarrow2x=-7\)
hay \(x=-\dfrac{7}{2}\)
b: Ta có: \(\left(x-2\right)^3-\left(x-4\right)\left(x^2+4x+16\right)+6\left(x+1\right)^2=49\)
\(\Leftrightarrow x^3-6x^2+12x-8-x^3+64+6\left(x+1\right)^2=49\)
\(\Leftrightarrow-6x^2+12x+56+6x^2+12x+6=49\)
\(\Leftrightarrow24x=-13\)
hay \(x=-\dfrac{13}{24}\)
2 tháng 7 2023
1: =>x^2+4x-21=0
=>(x+7)(x-3)=0
=>x=3 hoặc x=-7
2: =>(2x-5-4)(2x-5+4)=0
=>(2x-9)(2x-1)=0
=>x=9/2 hoặc x=1/2
3: =>x^3-9x^2+27x-27-x^3+27+9(x^2+2x+1)=15
=>-9x^2+27x+9x^2+18x+9=15
=>18x=15-9-27=-21
=>x=-7/6
6: =>4x^2+4x+1-4x^2-16x-16=9
=>-12x-15=9
=>-12x=24
=>x=-2
7: =>x^2+6x+9-x^2-4x+32=1
=>2x+41=1
=>2x=-40
=>x=-20
30 tháng 6 2021
Ta có:(x-3)(x2+3x+9)-x(x2-4)=1
=> x3-27-x3+4x=1
=>4x=28=>x=7
XO
6 tháng 8 2020
(3x - 4)(x - 2) = 3x(x - 9) - 3
=> 3x2 - 10x + 8 = 3x2 - 27x - 3
=> 27x - 10x = -3 - 8
=> 17x = -11
=> x = -11/17
6 tháng 8 2020
\(\left(3x-4\right)\left(x-2\right)=3x\left(x-9\right)-3\)
\(\Leftrightarrow3x^2-6x-4x+8=3x^2-27x-3\)
\(\Leftrightarrow3x^2-10x+8=3x^2-27x-3\)
\(\Leftrightarrow17x+11=0\)
\(\Leftrightarrow17x=-11\)
\(\Leftrightarrow x=\frac{-11}{17}\)
TT
16 tháng 11 2023
a) 1/4(x-3)+2=1/5
1/4.(x-3) = 1/5-2
1/4.(x-3) = -9/5
x-3 = (-9/5):1/4
x-3 = -36/5
x = -36/5+3
x= -21/5
30 tháng 12 2021
a x = \(\dfrac{-1}{12}\)
b x = \(\dfrac{-4}{3}\)
c x = \(\dfrac{-1}{6}\)
d x = \(\dfrac{-1}{4}\)
30 tháng 12 2021
\(\left(4x+1\right)^2=\dfrac{4}{9}\)
\(\left(4x+1\right)=\perp\left(\dfrac{2}{3}\right)^2\)
\(\text{Vậy }4x+1=\dfrac{2}{3}\)
\(4x\) \(=\dfrac{2}{3}+\left(-1\right)=\dfrac{-1}{3}\)
\(x\) \(=\left(\dfrac{-1}{3}\right).\dfrac{1}{4}=\dfrac{-1}{12}\)
\(\text{hoặc }4x+1=\dfrac{-2}{3}\)
\(4x\) \(=\left(\dfrac{-2}{3}\right)+\left(-1\right)=\dfrac{-5}{3}\)
\(x\) \(=\left(\dfrac{-5}{3}\right).\dfrac{1}{4}=\dfrac{-5}{12}\)
\(\Rightarrow x\in\left\{\dfrac{-1}{12};\dfrac{-5}{12}\right\}\)
\(\left(3x-1\right)^2=25\)
\(\left(3x-1\right)^2=\perp\left(5\right)^2\)
\(\text{Vậy }3x-1=5\)
\(3x\) \(=5+1=6\)
\(x\) \(=6:3=2\)
\(\text{hoặc }3x-1=-5\)
\(3x\) \(=\left(-5\right)+1=-4\)
\(x\) \(=\left(-4\right):3=\dfrac{-4}{3}\)
\(\Rightarrow x\in\left\{2;\dfrac{-4}{3}\right\}\)
\(\left(x-\dfrac{1}{3}\right)^2=\dfrac{1}{4}\)
\(\left(x-\dfrac{1}{3}\right)^2=\perp\left(\dfrac{1}{2}\right)^2\)
\(\text{Vậy }x-\dfrac{1}{3}=\dfrac{1}{2}\)
\(x\) \(=\dfrac{1}{2}+\dfrac{1}{3}=\dfrac{5}{6}\)
\(\text{hoặc }x-\dfrac{1}{3}=\dfrac{-1}{2}\)
\(x\) \(=\left(\dfrac{-1}{2}\right)+\dfrac{1}{3}=\dfrac{-1}{6}\)
\(\Rightarrow x\in\left\{\dfrac{5}{6};\dfrac{-1}{6}\right\}\)
\(\left(4x-3\right)^2=16\)
\(\left(4x-3\right)=\perp\left(4\right)^2\)
\(\text{Vậy }4x-3=4\)
\(4x\) \(=4+3=7\)
\(x\) \(=7:4=\dfrac{7}{4}\)
\(\text{hoặc }4x-3=-4\)
\(4x\) \(=\left(-4\right)+3=-1\)
\(x\) \(=\left(-1\right):4=\dfrac{-1}{4}\)
\(\Rightarrow x\in\left\{\dfrac{7}{4};\dfrac{-1}{4}\right\}\)
31 tháng 7 2023
\(4.3^x+3^{x+1}=63\)
\(\Rightarrow4.3^x+3.3^x=63\)
\(\Rightarrow7.3^x=63\Rightarrow3^x=9=3^2\Rightarrow x=2\)
\(9.\left(\dfrac{2}{3}\right)^{x+2}-\left(\dfrac{2}{3}\right)^x=\dfrac{4}{3}\)
\(\Rightarrow9.\left(\dfrac{2}{3}\right)^2\left(\dfrac{2}{3}\right)^x-\left(\dfrac{2}{3}\right)^x=\dfrac{4}{3}\)
\(\Rightarrow9.\dfrac{4}{9}^{ }.\left(\dfrac{2}{3}\right)^x-\left(\dfrac{2}{3}\right)^x=\dfrac{4}{3}\)
\(\Rightarrow\left(\dfrac{2}{3}\right)^x.\left(4-1\right)=\dfrac{4}{3}\)
\(\Rightarrow\left(\dfrac{2}{3}\right)^x.\dfrac{1}{3}=\dfrac{4}{3}\Rightarrow\left(\dfrac{2}{3}\right)^x=4\)
mà \(0< \left(\dfrac{2}{3}\right)^x< 1;4>0;x>0\)
\(\Rightarrow x\in\varnothing\)
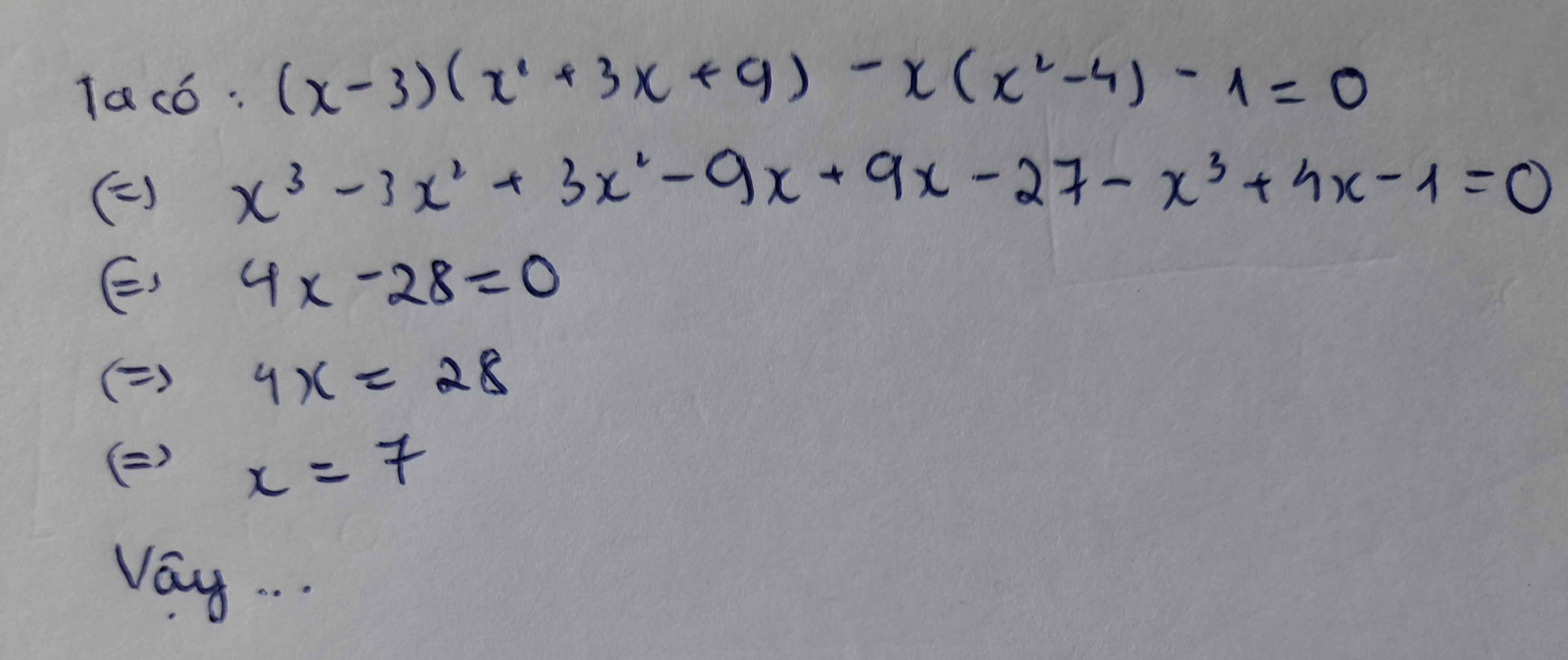
(9/4):(1/3x-1/2)=9/2
=> (1/3x-1/2)=9/4:9/2=9/4.2/9
=> (1/3x-1/2)=1/2
=> 1/3x =1/2+1/2
=> 1/3x =1
=> x =1:1/3=1.3
=> x =3