làm giúp mình và giải thích với ạ mình cảm ơn nhìu ><
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a)\(\frac{1}{n\left(n+1\right)\left(n+2\right)}=\frac{1}{2}.\frac{1}{n+1}.\left(\frac{1}{n}-\frac{1}{n+2}\right)\)=\(\frac{1}{2}.\frac{1}{n\left(n+1\right)}-\frac{1}{2}.\frac{1}{\left(n+1\right)\left(n+2\right)}\)= \(\frac{1}{2}\left(\frac{1}{n}-\frac{1}{n+1}\right)-\frac{1}{2}\left(\frac{1}{n+1}-\frac{1}{n+2}\right)\)
=> a = \(\frac{1}{2}\left(\frac{1}{1}-\frac{1}{2}\right)-\frac{1}{2}\left(\frac{1}{2}-\frac{1}{3}\right)\)+\(\frac{1}{2}\left(\frac{1}{2}-\frac{1}{3}\right)-\frac{1}{2}\left(\frac{1}{3}-\frac{1}{4}\right)\)+....+\(\frac{1}{2}\left(\frac{1}{2018}-\frac{1}{2019}\right)-\frac{1}{2}\left(\frac{1}{2019}-\frac{1}{2020}\right)\)=\(\frac{1}{2}\left(1-\frac{1}{2}\right)-\frac{1}{2}\left(\frac{1}{2019}-\frac{1}{2020}\right)\)=\(\frac{1}{4}\left(1-\frac{1}{2019.1010}\right)\)=\(\frac{2019.1010-1}{2.2019.2020}\)
b) tương tự \(\frac{1}{n\left(n+1\right)\left(n+2\right)\left(n+3\right)}=\left(\frac{1}{n}-\frac{1}{n+1}\right)\left(\frac{1}{n+2}-\frac{1}{n+3}\right)\)=\(\frac{1}{2}\left(\frac{1}{n}-\frac{1}{n+2}\right)-\left(\frac{1}{n+1}-\frac{1}{n+2}\right)\)-\(\frac{1}{3}\left(\frac{1}{n}-\frac{1}{n+3}\right)+\frac{1}{2}\left(\frac{1}{n+1}-\frac{1}{n+3}\right)\)=\(\frac{1}{6}\left(\frac{1}{n}-\frac{1}{n+1}\right)-\frac{1}{3}\left(\frac{1}{n+1}-\frac{1}{n+2}\right)\)+\(\frac{1}{6}\left(\frac{1}{n+2}-\frac{1}{n+3}\right)\)= M-P+N
Với n từ 1 đến 2017 thì
M= \(\frac{1}{6}\left(\frac{1}{1}-\frac{1}{2}\right)+\frac{1}{6}\left(\frac{1}{2}-\frac{1}{3}\right)+...\)+\(\frac{1}{6}\left(\frac{1}{2017}-\frac{1}{2018}\right)\)=\(\frac{1}{6}\left(1-\frac{1}{2018}\right)=\frac{2017}{6.2018}\)
N= \(\frac{1}{6}\left(\frac{1}{3}-\frac{1}{4}\right)+\frac{1}{6}\left(\frac{1}{4}-\frac{1}{5}\right)+...+\)\(\frac{1}{6}\left(\frac{1}{2019}-\frac{1}{2020}\right)=\)\(\frac{1}{6}\left(\frac{1}{3}-\frac{1}{2020}\right)=\frac{2017}{6.3.2020}\)
P= \(\frac{1}{3}\left(\frac{1}{2}-\frac{1}{3}\right)+\frac{1}{3}\left(\frac{1}{3}-\frac{1}{4}\right)+...+\)\(\frac{1}{3}\left(\frac{1}{2018}-\frac{1}{2019}\right)\)= \(\frac{1}{3}\left(\frac{1}{2}-\frac{1}{2019}\right)=\frac{2017}{3.2.2019}\)
M+N-P = \(\frac{2017}{6}\left(\frac{1}{2018}+\frac{1}{3.2020}-\frac{1}{2019}\right)\)=\(\frac{2017}{6}.\left(\frac{1}{2018.2019}+\frac{1}{3.2020}\right)\)
= \(\frac{2017\left(1010+1009.673\right)}{3.2018.2019.2020}\)

12.
\(y=\sqrt{2}sin\left(2x+\dfrac{\pi}{4}\right)\le\sqrt[]{2}\)
\(\Rightarrow M=\sqrt{2}\)
13.
Pt có nghiệm khi:
\(5^2+m^2\ge\left(m+1\right)^2\)
\(\Leftrightarrow2m\le24\)
\(\Rightarrow m\le12\)
14.
\(\Leftrightarrow\left[{}\begin{matrix}cosx=1\\cosx=-\dfrac{5}{3}\left(loại\right)\end{matrix}\right.\)
\(\Leftrightarrow x=k2\pi\)
15.
\(\Leftrightarrow\left[{}\begin{matrix}tanx=-1\\tanx=3\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=-\dfrac{\pi}{4}+k\pi\\x=arctan\left(3\right)+k\pi\end{matrix}\right.\)
Đáp án A
16.
\(\dfrac{\sqrt{3}}{2}sinx-\dfrac{1}{2}cosx=\dfrac{1}{2}\)
\(\Leftrightarrow sin\left(x-\dfrac{\pi}{6}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{6}=\dfrac{\pi}{6}+k2\pi\\x-\dfrac{\pi}{6}=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{3}+k2\pi\\x=\pi+k2\pi\end{matrix}\right.\)
\(\left[{}\begin{matrix}2\pi\le\dfrac{\pi}{3}+k2\pi\le2018\pi\\2\pi\le\pi+k2\pi\le2018\pi\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}1\le k\le1008\\1\le k\le1008\end{matrix}\right.\)
Có \(1008+1008=2016\) nghiệm

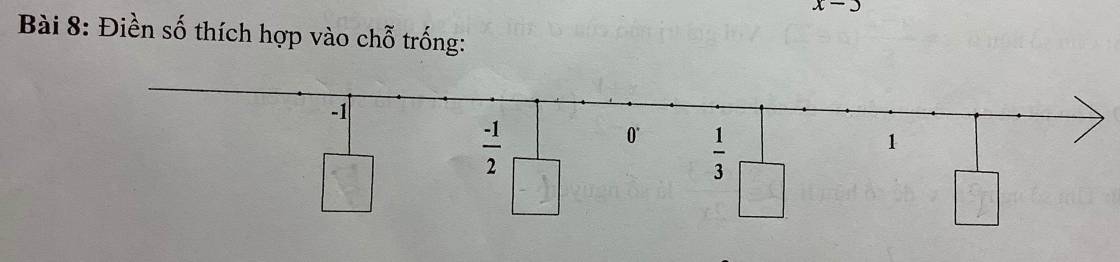
Các số được điền vào các ô theo thứ tự từ trái sang phải là:
-1; - \(\dfrac{1}{3}\); \(\dfrac{2}{3}\); \(\dfrac{4}{3}\)

Ta có
\(\frac{1}{2}=\frac{1\times3\times5}{2\times3\times5}=\frac{15}{30}\)
\(\frac{1}{3}=\frac{1\times2\times5}{3\times2\times5}=\frac{10}{30}\)
\(\frac{2}{5}=\frac{2\times2\times3}{5\times2\times3}=\frac{12}{30}\)
Hok tốt !!!!!!!!!!!!!!!!!!!

\(3x+2.\left(x-3\right)-x=2010\)
\(\Leftrightarrow3x+2x-6-x=2010\)
\(\Leftrightarrow4x-6=2010\)
\(\Leftrightarrow4x=2016\)
\(\Leftrightarrow x=504\)
Vậy...
\(3\times+2\left(\times-3\right)-\times=2010\)
\(\Rightarrow2\times+2\times-6=2010\)
\(\Rightarrow4\times-6=2010\)
\(\Rightarrow4\times=2016\)
\(\Rightarrow\times=504\)






mng ới ;-;
Bài 1 :
\(\Delta'=m^2-2\left(m-2018\right)=m^2-2m+2018=\left(m-1\right)^2+2017>0\forall m\)
Vậy pt luôn có 2 nghiệm phân biệt