Giúp mình câu c bài 2 với ạ 😣😓😓😓
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Phương trình hoành độ giao điểm (d) và (P):
\(x^2-2x-3=ax-a-3\)
\(\Leftrightarrow x^2-\left(a+2\right)x+a=0\)
\(\Delta=\left(a+2\right)^2-4a=a^2+4>0;\forall a\Rightarrow\) (d) luôn cắt (P) tại 2 điểm pb
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_A+x_B=a+2\\x_Ax_B=a\end{matrix}\right.\)
Mặt khác do A, B thuộc (d) nên: \(\left\{{}\begin{matrix}y_A=ax_A-a-3\\y_B=ax_B-a-3\end{matrix}\right.\)
\(y_A+y_B=0\)
\(\Leftrightarrow a\left(x_A+x_B\right)-2a-6=0\)
\(\Leftrightarrow a\left(a+2\right)-2a-6=0\)
\(\Leftrightarrow a^2-6=0\)
\(\Leftrightarrow a=\pm\sqrt{6}\)

C3: Hệ bpt trở thành: \(\left\{{}\begin{matrix}x\ge1-m\\mx\ge2-m\end{matrix}\right.\)
a, Để hệ phương trình vô nghiệm thì \(m=0\)
b, Để hệ có nghiệm duy nhất thì \(\left\{{}\begin{matrix}m\ne0\\\dfrac{m-2}{m}=1-m\end{matrix}\right.\)
\(\Leftrightarrow\) \(\left\{{}\begin{matrix}m\ne0\\m=\pm\sqrt{2}\end{matrix}\right.\) \(\Leftrightarrow\) \(m=\pm\sqrt{2}\)
c, \(x\in\left[-1;2\right]\) \(\Leftrightarrow\) \(-1\le x\le2\)
Để mọi \(x\in\left[-1;2\right]\) là nghiệm của hệ bpt trên thì
\(\left\{{}\begin{matrix}-1\le1-m\le2\\-1\le\dfrac{2-m}{m}\le2\end{matrix}\right.\) với \(m\ne0\)
\(\Leftrightarrow\) \(\left\{{}\begin{matrix}2\ge m\ge-1\\m\ge\dfrac{2}{3}\end{matrix}\right.\) \(\left(m\ne0\right)\)
\(\Leftrightarrow\) \(2\ge m\ge\dfrac{2}{3}\)
Vậy \(m\in\left[\dfrac{2}{3};2\right]\) thì mọi \(x\in\left[-1;2\right]\) là nghiệm của hệ bpt
Chúc bn học tốt!

Câu 16.
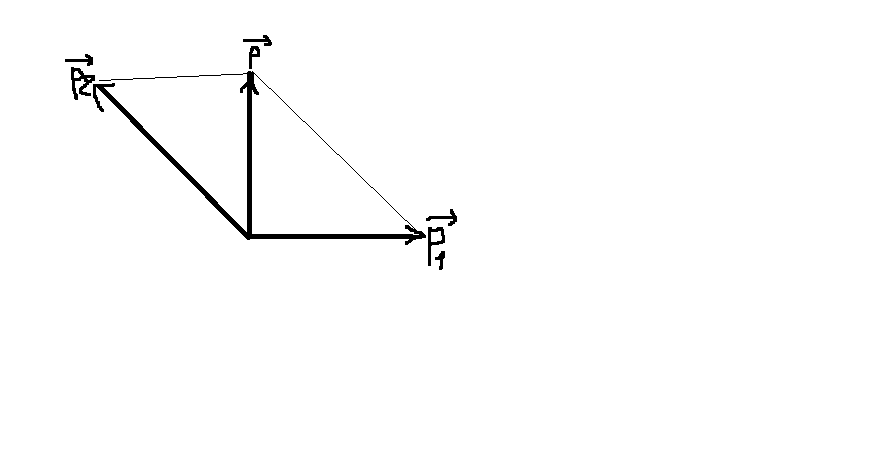
Hình vẽ tương đối thôi nha!!!
Bảo toàn động lương ta có:
\(\overrightarrow{p}=\overrightarrow{p_1}+\overrightarrow{p_2}\)
\(\Rightarrow p^2=p_2^2-p_1^2\)\(\Rightarrow p_2=\sqrt{p^2+p_1^2}\)
\(\Rightarrow m_2\cdot v_2=\sqrt{\left(m_1+m_2\right)\cdot v+m_1\cdot v_1}\)
\(\Rightarrow0,3\cdot v_2=\sqrt{[\left(0,5+0,3\right)\cdot3]^2+(0,5\cdot4)^2}=3,124\)
\(\Rightarrow v_2=10,41\)m/s

27.
Bán kính mặt cầu ngoại tiếp tứ diện vuông được tính bằng:
\(R=\sqrt{\dfrac{OA^2+OB^2+OC^2}{4}}=\sqrt{\dfrac{1^2+2^2+3^2}{4}}=\dfrac{\sqrt{14}}{2}\)
28.
Từ giả thiết suy ra \(A\left(2;2;2\right)\)
Gọi điểm thuộc mặt Oxz có tọa độ dạng \(D\left(x;0;z\right)\)
\(\Rightarrow\overrightarrow{AD}=\left(x-2;-2;z-2\right)\)
\(\overrightarrow{BD}=\left(x+2;-2;z\right)\) ; \(\overrightarrow{CD}=\left(x-4;-1;z+1\right)\)
D cách đều A, B, C \(\Rightarrow\left\{{}\begin{matrix}AD=BD\\AD=CD\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\left(x-2\right)^2+4+\left(z-2\right)^2=\left(x+2\right)^2+4+z^2\\\left(x-2\right)^2+4+\left(z-2\right)^2=\left(x-4\right)^2+1+\left(z+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x+z=1\\2x-3z=3\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}z=-\dfrac{1}{2}\\x=\dfrac{3}{4}\end{matrix}\right.\)
\(\Rightarrow P\left(\dfrac{3}{4};0;-\dfrac{1}{2}\right)\)
29.
Do tâm I mặt cầu thuộc Oz nên tọa độ có dạng: \(I\left(0;0;z\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AI}=\left(-3;1;z-2\right)\\\overrightarrow{BI}=\left(-1;-1;z+2\right)\end{matrix}\right.\)
Mặt cầu qua A, B nên \(AI=BI\)
\(\Leftrightarrow3^2+1^2+\left(z-2\right)^2=1^2+1^2+\left(z+2\right)^2\)
\(\Leftrightarrow8z=8\Rightarrow z=1\)
\(\Rightarrow I\left(0;0;1\right)\Rightarrow R=IB=\sqrt{1^2+1^1+3^2}=\sqrt{11}\)
Phương trình mặt cầu:
\(x^2+y^2+\left(z-1\right)^2=11\)


1. Many young men prefer scuba - diving to water polo beacause it is .....
⇒ Đáp án: A. adventure
2. .... players are not allowed to interfere with the opponent's movements unless the player is holding ball
⇒ Đáp án: C. defender
3. Curits's water ballet club ... both national and international publicity
⇒ Đáp án: A. attract
4. Scuba - diving can be ... because you can ... be attracked by sharks
⇒ Đáp án: C. Dangerous / Easily
5. After livng school, many of us only read for .....
⇒ Đáp án: D. pleasure
6. Many enjoys reading ..., adventure, and whatever else can either buy or borrow
⇒ Đáp án: A. romance
7. Read the book ... and you can find the information you need
⇒ Đáp án: D. carefully
8. Increasing trade in ivory products is a threat to the ... of elephants
⇒ Đáp án: D. survival
9. Chemical wastes from factories are ... that casue serious damage to species habitats
⇒ Đáp án: B. pollutants
10. The elephants are ... for their tusks
⇒ Đáp án: C. poaching


\(\dfrac{x-3}{3x-5}< \dfrac{3x-5}{x-3}.\left(x\ne3;x\ne\dfrac{5}{3}\right).\)
\(\Leftrightarrow\dfrac{x-3}{3x-5}-\dfrac{3x-5}{x-3}< 0.\Leftrightarrow\dfrac{\left(x-3\right)^2-\left(3x-5\right)^2}{\left(3x-5\right)\left(x-3\right)}< 0.\)
\(\Leftrightarrow\dfrac{x^2-6x+9-\left(9x^2-30x+25\right)}{\left(3x-5\right)\left(x-3\right)}< 0.\) \(\Leftrightarrow\dfrac{x^2-6x+9-9x^2+30x-25}{\left(3x-5\right)\left(x-3\right)}< 0.\)
\(\Leftrightarrow\dfrac{-8x^2+24x-16}{\left(3x-5\right)\left(x-3\right)}< 0.\Leftrightarrow\dfrac{8x^2-24x+16}{\left(3x-5\right)\left(x-3\right)}>0.\)
\(\Leftrightarrow\dfrac{8\left(x^2-3x+2\right)}{\left(3x-5\right)\left(x-3\right)}>0.\Leftrightarrow\dfrac{\left(x-2\right)\left(x-1\right)}{\left(3x-5\right)\left(x-3\right)}>0.\)
Đặt \(\dfrac{\left(x-2\right)\left(x-1\right)}{\left(3x-5\right)\left(x-3\right)}=f\left(x\right).\)
Lập bảng xét dấu:
| x | \(-\infty\) 1 \(\dfrac{5}{3}\) 2 3 \(+\infty\) |
| x - 2 | - | - | - 0 + | + |
| x - 1 | - 0 + | + | + | + |
| 3x - 5 | - | - 0 + | + | + |
| x - 3 | - | - | - | - 0 + |
| f (x) | + 0 - || + 0 - || + |
Vậy \(\dfrac{\left(x-2\right)\left(x-1\right)}{\left(3x-5\right)\left(x-3\right)}=f\left(x\right)>0.\) \(\Leftrightarrow x\in\left(-\infty;1\right)\cup\left(\dfrac{5}{3};2\right)\cup\left(3;+\infty\right).\)
a)
\(\left\{{}\begin{matrix}2x^2+7x-4\ge x^2-4\\\dfrac{2x-1}{x^2+x-2}< \dfrac{2x-5}{x^2+x-2}\end{matrix}\right.\)
<=>\(\left\{{}\begin{matrix}x^2+7\ge0\\\dfrac{2x-5-2x+1}{x^2+x-2}>0\end{matrix}\right.\)
<=>\(\left\{{}\begin{matrix}x\left(x+7\right)\ge0\\\dfrac{-4}{x^2+x-2}>0\end{matrix}\right.\)
=> \(\left\{{}\begin{matrix}x\left(x+7\right)\ge0\\\left(x-1\right)\left(x+2\right)< 0\end{matrix}\right.\)
ta có x+2>x-1
=>x-1<0 và x+2 >0 để thỏa điều kiện =>x<1 và x>-2(hay -2<x<1)(1)
vì -2<x<1 nên x+7>0
=>x\(\ge\)0 để thỏa điều kiện(2)
từ (1) và (2) =>0\(\le\)x<1
b)
\(\left\{{}\begin{matrix}\left(x-3\right)\left(\sqrt{2}-x\right)>0\\4x-3< 2\left(x+3\right)\end{matrix}\right.\)
<=>\(\left\{{}\begin{matrix}\left(x-3\right)\left(\sqrt{2}-x\right)>0\\2x-9< 0\end{matrix}\right.\)
<=>\(\left\{{}\begin{matrix}\left(x-3\right)\left(\sqrt{2}-x\right)>0\\x< \dfrac{9}{2}\end{matrix}\right.\)
có 2 TH xảy ra để thỏa điều kiện
TH1 (x-3)<0 và (\(\sqrt{2}\)-x)<0=>\(\sqrt{2}\)<x<3(nhận)
TH2 (x-3)>0 và (\(\sqrt{2}\)-x)>0=>3<x<\(\sqrt{2}\)(loại)
em nghĩ như nào làm như v thôi có gì sai chị xem và sửa hộ em nhá ![]()