Giải phương trình: (x2 + 5x)2 - 2(x2 + 5x) = 24
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
PT
1
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 3 2020
Bài 1
a/ \(x\left(x^2+1\right)+2\left(x^2+1\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x^2+1\right)=0\Rightarrow x=-2\)
b/
\(\Leftrightarrow x^3-6x^2+9x+5x^2-30x+45=0\)
\(\Leftrightarrow x\left(x-3\right)^2+5\left(x-3\right)^2=0\)
\(\Leftrightarrow\left(x+5\right)\left(x-3\right)^2=0\)
\(\Rightarrow\left[{}\begin{matrix}x=-5\\x=3\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 3 2020
1.
c/ \(\Leftrightarrow x^3+2x^2+2x+x^2+2x+2=0\)
\(\Leftrightarrow x\left(x^2+2x+2\right)+x^2+2x+2=0\)
\(\Leftrightarrow\left(x+1\right)\left(x^2+2x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x^2+2x+2=0\left(vn\right)\end{matrix}\right.\)
d/
\(\Leftrightarrow x^4+x^3-2x^2-x^3-x^2+2x+4x^2+4x-8=0\)
\(\Leftrightarrow x^2\left(x^2+x-2\right)-x\left(x^2+x-2\right)+4\left(x^2+x-2\right)=0\)
\(\Leftrightarrow\left(x^2-x+4\right)\left(x^2+x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-x+4=0\left(vn\right)\\x^2+x-2=0\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
23 tháng 2 2022
Bài 1:
a: \(\Leftrightarrow x^2-5x+6< =0\)
=>(x-2)(x-3)<=0
=>2<=x<=3
b: \(\Leftrightarrow\left(x-6\right)^2< =0\)
=>x=6
c: \(\Leftrightarrow x^2-2x+1>=0\)
\(\Leftrightarrow\left(x-1\right)^2>=0\)
hay \(x\in R\)
TC
14 tháng 4 2022
â) thay m = 6 và phương trình ta đc
\(x^2-5x+6=0\)
\(\Leftrightarrow\left(x-2\right).\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\x-3=0\end{matrix}\right.=>\left[{}\begin{matrix}x=2\\x=3\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 4 2022
b.
Phương trình có 2 nghiệm khi: \(\Delta=25-4m\ge0\Rightarrow m\le\dfrac{25}{4}\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=5\\x_1x_2=m\end{matrix}\right.\)
Pt có 2 nghiệm dương khi \(m>0\)
\(x_1\sqrt{x_2}+x_2\sqrt{x_1}=6\)
\(\Leftrightarrow x_1^2x_2+x_2^2x_1+2x_1x_2\sqrt{x_1x_2}=36\)
\(\Leftrightarrow x_1x_2\left(x_1+x_2\right)+2x_1x_2\sqrt{x_1x_2}=36\)
\(\Leftrightarrow5m+2m\sqrt{m}=36\)
Đặt \(\sqrt{m}=t>0\Rightarrow2t^3+5t^2-36=0\)
\(\Leftrightarrow\left(t-2\right)\left(2t^2+9t+18\right)=0\)
\(\Leftrightarrow t=2\Rightarrow\sqrt{m}=2\)
\(\Rightarrow m=4\)
5 tháng 3 2022
1.\(\left(x+2\right)\left(2x-3\right)=x^2-4\)
\(\Leftrightarrow\left(x+2\right)\left(2x-3\right)-\left(x+2\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(2x-3-x+2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\)
2.\(x^2+3x+2=0\)
\(\Leftrightarrow x^2+x+2x+2=0\)
\(\Leftrightarrow x\left(x+1\right)+2\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-2\end{matrix}\right.\)
3.\(2x^2+5x+3=0\)
\(\Leftrightarrow2x^2+2x+3x+3=0\)
\(\Leftrightarrow2x\left(x+1\right)+3\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(2x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-\dfrac{3}{2}\end{matrix}\right.\)
4.\(x^3+x^2-12x=0\)
\(\Leftrightarrow x\left(x^2+x-12\right)=0\)
\(\Leftrightarrow x\left(x+4\right)\left(x-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-4\\x=3\end{matrix}\right.\)
5 tháng 3 2022
a: \(\Leftrightarrow\left(x+2\right)\left(2x-3\right)-\left(x+2\right)\left(x-2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(2x-3-x+2\right)=0\)
=>(x+2)(x-1)=0
=>x=-2 hoặc x=1
b: =>(x+1)(x+2)=0
=>x=-1 hoặc x=-2
c: =>(2x+3)(x+1)=0
=>x=-1 hoặc x=-3/2
d: =>x(x+4)(x-3)=0
hay \(x\in\left\{0;-4;3\right\}\)
4 tháng 1 2021
\(ĐKXĐ:x\ge2\)
Phương trình đã cho \(\Leftrightarrow x^2-5x-2\sqrt{x-2}+8=0\)
\(\Leftrightarrow\left(x^2-6x+9\right)+\left(x-2-2\sqrt{x-2}+1\right)=0\)
\(\Leftrightarrow\left(x-3\right)^2+\left(\sqrt{x-2}-1\right)^2=0\)
Dấu "=" xảy ra \(\Leftrightarrow\left\{{}\begin{matrix}\left(x-3\right)^2=0\\\left(\sqrt{x-2}-1\right)^2=0\end{matrix}\right.\) \(\Leftrightarrow x=3\) ( Thỏa mãn )
Vậy pt đã cho có nghiệm duy nhất \(x=3\)
HX
2
Y
3 tháng 3 2023
\(x^2+2\left(2+\sqrt{x-1}\right)=5x\)
\(\Leftrightarrow x^2+4+2\sqrt{x-1}-5x=0\)
\(\Leftrightarrow x^2-5x+2\sqrt{x-1}+4=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)
CM
19 tháng 11 2017
Đáp án B
Phương trình x 2 - 5 x + 2 = 0 có hai nghiệm x 1 ; x 2
Theo hệ thức Vi-ét ta có:
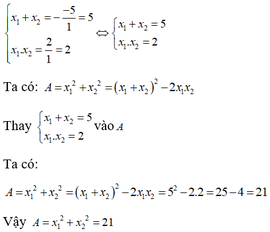
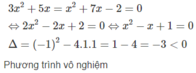
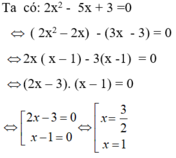
Đặt \(x^2+5x=t\)
\(\Leftrightarrow t^2-2t-24=0\Leftrightarrow\left(t-6\right)\left(t+4\right)=0\Leftrightarrow t=6;t=-4\)
hay \(x^2+5x-6=0\Leftrightarrow\left(x-1\right)\left(x+6\right)=0\Leftrightarrow x=1;x=-6\)
\(x^2+5x+4=0\Leftrightarrow\left(x+1\right)\left(x+4\right)=0\Leftrightarrow x=-1;x=-4\)
Vậy tập nghiệm phương trình là S = { \(\pm\)1 ; -6 ; -4 }
Ta có: \(\left(x^2+5x\right)^2-2.\left(x^2+5x\right)-24=0\)
\(\Leftrightarrow\left(x^2+5x\right)^2-6.\left(x^2+5x\right)+4.\left(x^2+5x\right)-24=0\)
\(\Leftrightarrow\left(x^2+5x\right).\left(x^2+5x-6\right)+4.\left(x^2+5x-6\right)=0\)
\(\Leftrightarrow\left(x^2+5x+4\right).\left(x^2+5x-6\right)=0\)
\(\Leftrightarrow\left(x^2+x+4x+4\right).\left(x^2-x+6x-6\right)=0\)
\(\Leftrightarrow\left(x+1\right).\left(x+4\right).\left(x-1\right).\left(x+6\right)=0\)
Suy ra: \(x=-1\)hoặc \(x=-4\)hoặc \(x=1\)hoặc \(x=-6\)
Vậy .........