Tìm GTNN của: \(B=\dfrac{x+1}{x}\) với \(0< x\le\dfrac{1}{4}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Ta có: \(\dfrac{a+b}{2}\ge\sqrt{ab}\)
\(\Leftrightarrow a+b\ge2\sqrt{ab}\)
\(\Leftrightarrow a-2\sqrt{ab}+b\ge0\)
\(\Leftrightarrow\left(\sqrt{a}-\sqrt{b}\right)^2\ge0\)(luôn đúng)

*Chứng minh bất đẳng thức
Ta có: \(\forall a,b\ge0\) thì \(\left(\sqrt{a}-\sqrt{b}\right)^2\ge0\)
\(\Leftrightarrow a+b-2\sqrt{ab}\ge0\) \(\Leftrightarrow a+b\ge2\sqrt{ab}\) \(\Leftrightarrow\dfrac{a+b}{2}\ge\sqrt{ab}\) (đpcm)
Ta có: \(\left(\sqrt{a}-\sqrt{b}\right)^2\ge0\forall a,b>0\)
\(\Leftrightarrow a-2\sqrt{ab}+b\ge0\forall a,b>0\)
\(\Leftrightarrow a+b\ge2\sqrt{ab}\forall a,b>0\)
\(\Leftrightarrow\dfrac{a+b}{2}\ge\sqrt{ab}\forall a,b>0\)(đpcm)

Áp dụng BĐT Cauchy schwarz dưới dạng en-gel ta có :
\(B=\dfrac{4}{x}+\dfrac{9}{1-x}\ge\dfrac{\left(2+3\right)^2}{x+1-x}=25\)
Dấu \("="\)xảy ra khi \(\dfrac{2}{x}=\dfrac{3}{1-x}\Leftrightarrow x=\dfrac{2}{5}\)

1.
\(f\left(x\right)=\dfrac{4}{x}+\dfrac{x-1+1}{1-x}=\dfrac{2^2}{x}+\dfrac{1}{1-x}-1\ge\dfrac{\left(2+1\right)^2}{x+1-x}-1=8\)
\(f\left(x\right)_{min}=8\) khi \(x=\dfrac{2}{3}\)
2.
\(f\left(x\right)=\dfrac{1}{x}+\dfrac{1}{1-x}\ge\dfrac{4}{x+1-x}=4\)
\(f\left(x\right)_{min}=4\) khi \(x=\dfrac{1}{2}\)
f(x)=4x+x−1+11−x=22x+11−x−1≥(2+1)2x+1−x−1=8f(x)=4x+x−1+11−x=22x+11−x−1≥(2+1)2x+1−x−1=8
f(x)min=8f(x)min=8 khi x=23x=23
2.
f(x)=1x+11−x≥4x+1−x=4f(x)=1x+11−x≥4x+1−x=4
f(x)min=4f(x)min=4 khi x=12

\(P=\dfrac{4}{x}+1+\dfrac{9}{1-x}=\dfrac{4}{x}+25x+25\left(1-x\right)+\dfrac{9}{1-x}-24\)
\(\Rightarrow P\ge2\sqrt{\dfrac{4}{x}.25x}+2\sqrt{25\left(1-x\right).\dfrac{9}{1-x}}-24\)
\(\Rightarrow P\ge20+30-24=26\)
\(\Rightarrow P_{min}=26\)
Dấu "=" xảy ra khi \(\left\{{}\begin{matrix}\dfrac{4}{x}=25x\\25\left(1-x\right)=\dfrac{9}{1-x}\end{matrix}\right.\) \(\Rightarrow x=\dfrac{2}{5}\)

Lời giải:
$A=(x-y)+\frac{4}{x-y}+y+\frac{1}{y}$
Áp dụng BĐT Cô-si:
$(x-y)+\frac{4}{x-y}\geq 2\sqrt{(x-y).\frac{4}{x-y}}=4$
$y+\frac{1}{y}\geq 2$
$\Rightarrow A\geq 4+2=6$
Vậy $A_{\min}=6$ khi $(x,y)=(3,1)$

1) Áp dụng bđt Cauchy cho 3 số dương ta có
\(\dfrac{1}{x}+\dfrac{1}{x}+\dfrac{1}{x}+x^3\ge4\sqrt[4]{\dfrac{1}{x}.\dfrac{1}{x}.\dfrac{1}{x}.x^3}=4\) (1)
\(\dfrac{3}{y^2}+y^2\ge2\sqrt{\dfrac{3}{y^2}.y^2}=2\sqrt{3}\) (2)
\(\dfrac{3}{z^3}+z=\dfrac{3}{z^3}+\dfrac{z}{3}+\dfrac{z}{3}+\dfrac{z}{3}\ge4\sqrt[4]{\dfrac{3}{z^3}.\dfrac{z}{3}.\dfrac{z}{3}.\dfrac{z}{3}}=4\sqrt{3}\) (3)
Cộng (1);(2);(3) theo vế ta được
\(\left(\dfrac{3}{x}+\dfrac{3}{y^2}+\dfrac{3}{z^3}\right)+\left(x^3+y^2+z\right)\ge4+2\sqrt{3}+4\sqrt{3}\)
\(\Leftrightarrow3\left(\dfrac{1}{x}+\dfrac{1}{y^2}+\dfrac{1}{z^3}\right)\ge3+4\sqrt{3}\)
\(\Leftrightarrow P\ge\dfrac{3+4\sqrt{3}}{3}\)
Dấu "=" xảy ra <=> \(\left\{{}\begin{matrix}\dfrac{1}{x}=x^3\\\dfrac{3}{y^2}=y^2\\\dfrac{3}{z^3}=\dfrac{z}{3}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=\sqrt[4]{3}\\z=\sqrt{3}\end{matrix}\right.\) (thỏa mãn giả thiết ban đầu)
2) Ta có \(4\sqrt{ab}=2.\sqrt{a}.2\sqrt{b}\le a+4b\)
Dấu"=" khi a = 4b
nên \(\dfrac{8}{7a+4b+4\sqrt{ab}}\ge\dfrac{8}{7a+4b+a+4b}=\dfrac{1}{a+b}\)
Khi đó \(P\ge\dfrac{1}{a+b}-\dfrac{1}{\sqrt{a+b}}+\sqrt{a+b}\)
Đặt \(\sqrt{a+b}=t>0\) ta được
\(P\ge\dfrac{1}{t^2}-\dfrac{1}{t}+t=\left(\dfrac{1}{t^2}-\dfrac{2}{t}+1\right)+\dfrac{1}{t}+t-1\)
\(=\left(\dfrac{1}{t}-1\right)^2+\dfrac{1}{t}+t-1\)
Có \(\dfrac{1}{t}+t\ge2\sqrt{\dfrac{1}{t}.t}=2\) (BĐT Cauchy cho 2 số dương)
nên \(P=\left(\dfrac{1}{t}-1\right)^2+\dfrac{1}{t}+t-1\ge\left(\dfrac{1}{t}-1\right)^2+1\ge1\)
Dấu "=" xảy ra <=> \(\left\{{}\begin{matrix}\dfrac{1}{t}-1=0\\t=\dfrac{1}{t}\end{matrix}\right.\Leftrightarrow t=1\)(tm)
khi đó a + b = 1
mà a = 4b nên \(a=\dfrac{4}{5};b=\dfrac{1}{5}\)
Vậy MinP = 1 khi \(a=\dfrac{4}{5};b=\dfrac{1}{5}\)

\(c,P=\dfrac{x^2-x^2+8xy-16y^2}{x^2+4y^2}=\dfrac{8\left(\dfrac{x}{y}\right)-16}{\left(\dfrac{x}{y}\right)^2+4}\)
Đặt \(\dfrac{x}{y}=t\)
\(\Leftrightarrow P=\dfrac{8t-16}{t^2+4}\Leftrightarrow Pt^2+4P=8t-16\\ \Leftrightarrow Pt^2-8t+4P+16=0\)
Với \(P=0\Leftrightarrow t=2\)
Với \(P\ne0\Leftrightarrow\Delta'=16-P\left(4P+16\right)\ge0\)
\(\Leftrightarrow-P^2-4P+4\ge0\Leftrightarrow-2-2\sqrt{2}\le P\le-2+2\sqrt{2}\)
Vậy \(P_{max}=-2+2\sqrt{2}\Leftrightarrow t=\dfrac{4}{P}=\dfrac{4}{-2+2\sqrt{2}}=2+\sqrt{2}\)
\(\Leftrightarrow\dfrac{x}{y}=2+2\sqrt{2}\)
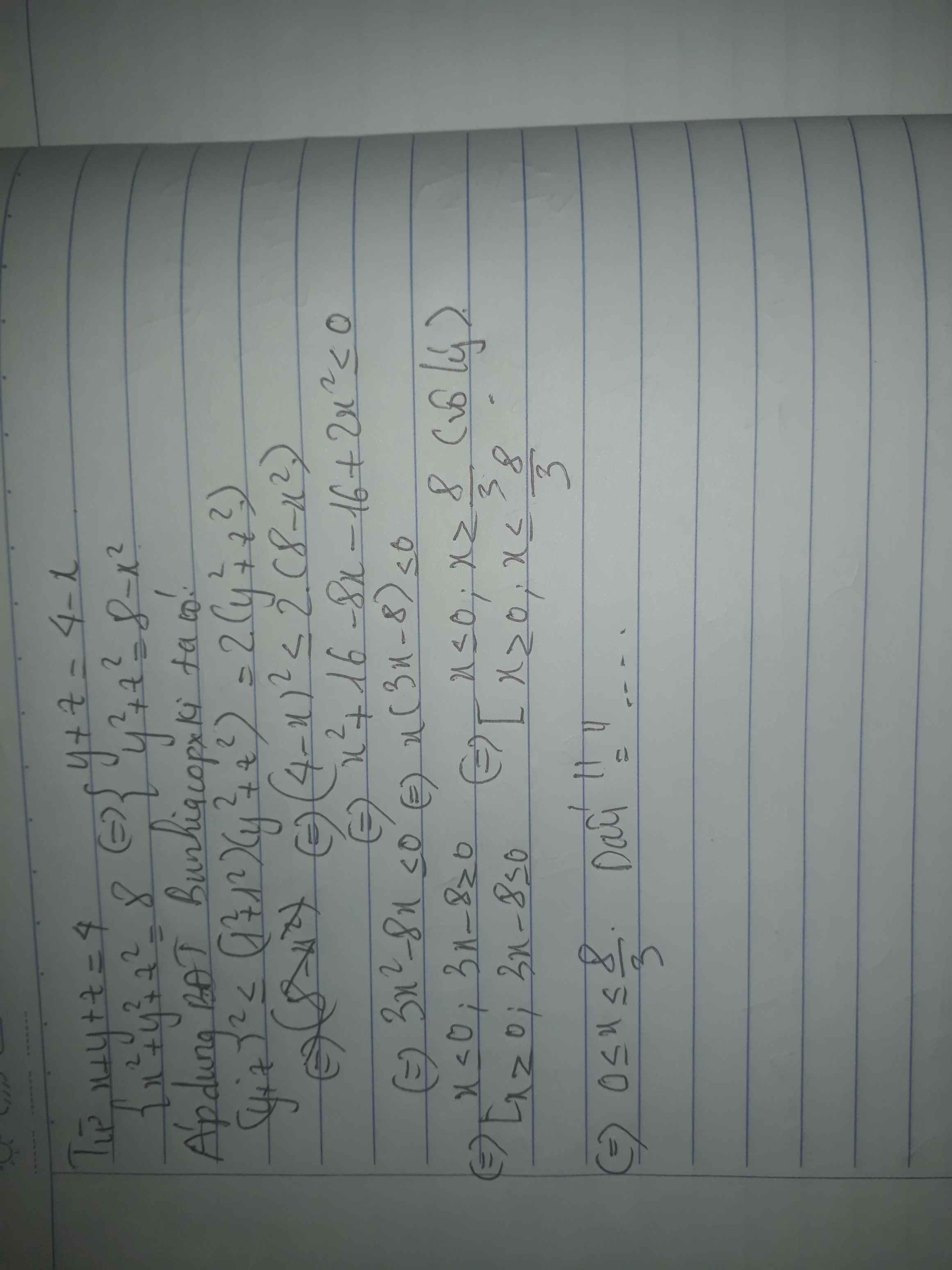
Ta có: x \(\le\) \(\dfrac{1}{4}\)
\(\Rightarrow\) \(\dfrac{1}{x}\ge4\)
Lại có: B = \(\dfrac{x+1}{x}=1+\dfrac{1}{x}\)
\(\Rightarrow\) 1 + \(\dfrac{1}{x}\) \(\ge\) 1 + 4 = 5
hay B \(\ge\) 5
Dấu "=" xảy ra khi và chỉ khi x = \(\dfrac{1}{4}\)
Chúc bn học tốt!