Giúp mình giải câu 3 với ạ.thank
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
NT
Ngô Thành Chung
9 tháng 1 2021
Đúng(1)
NT
Ngô Thành Chung
9 tháng 1 2021
Đúng(0)
Những câu hỏi liên quan
BG
27 tháng 8 2021
1. By the time, I decided to go on a diet, I had put on ten kilos.
2. They waxed the car after they had washed it.
3. Before Lisa sat on the sofa to watch TV, she had made a sandwich
4. As soon as we packed our suitcases, we left for the airport
5. When the lecture started, I entered the lecture hall
6. This is the first time I have tasted Chinese food
7. He has never read a science-fiction book before
8. Như câu 2
9. While Mark was parking his car, he noticed the wing-mirror was broken
10. Ad Judy was walking down the street, she saw an accident
11. I'm sorry I can't take to you now as I'm about to work
12. The Council intends to close the old swimming pool
13. They are planning to have a big wedding in the summer
14. The economy is on the point of collapsing
15. My grandfather is about to go far, far far far far far far far away : (
16. You are due to arrive at 7.30 in the evening
DD
0
28 tháng 3 2022
xin lỗi, mình không giúp được, bạn tự làm đi nhé:)
mình đang thắc mắc là không có giám thị hay sao mà bạn chụp được hẳn cái đề thi như này cũng chịu
Trời ơi!
27 tháng 10 2021
Bài 4:
b: Xét ΔAHB vuông tại H có HM là đường cao
nên \(AM\cdot AB=AH^2\left(1\right)\)
Xét ΔAHC vuông tại H có HN là đường cao
nên \(AN\cdot AC=AH^2\left(2\right)\)
Từ (1) và (2) suy ra \(AM\cdot AB=AN\cdot AC\)
27 tháng 10 2021
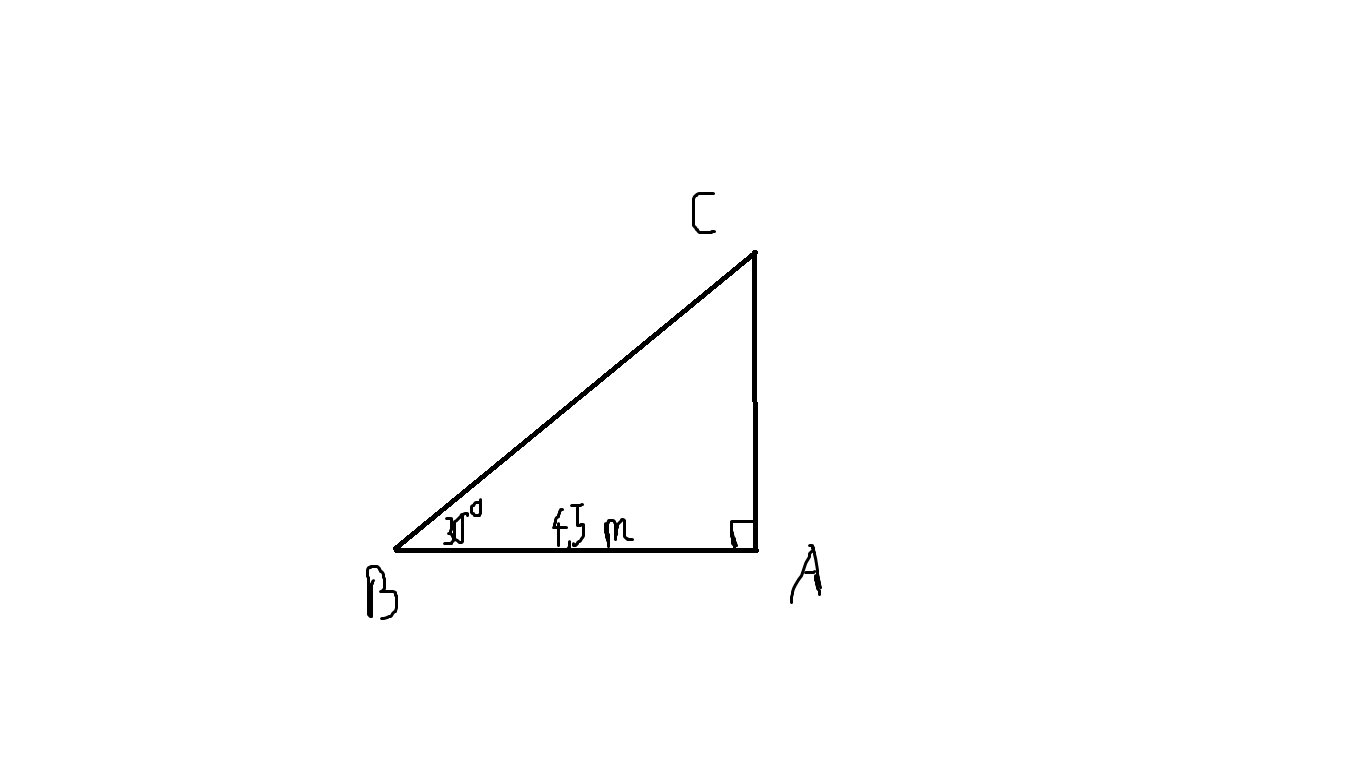
\(TanB=\dfrac{AC}{AB}\Rightarrow Tan30^o=\dfrac{AC}{4,5}\Rightarrow AC=Tan30^o.4,5=\dfrac{3\sqrt{3}}{2}\left(m\right)\)
\(CosB=\dfrac{AB}{BC}\Rightarrow Cos30^o=\dfrac{4,5}{BC}\Rightarrow BC=Cos30^o.4,5=\dfrac{9\sqrt{3}}{4}\)
Chiều cao ban đầu của cây tre là: \(\dfrac{3\sqrt{3}}{2}+\dfrac{9\sqrt{3}}{4}=\dfrac{15\sqrt{3}}{4}\approx6,5\left(m\right)\)
8 tháng 4 2022
Câu 4:
Thay x=2 và y=-1 vào hệ, ta được:
\(\left\{{}\begin{matrix}2a-b=4\\2b+2=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}b=-2\\a=1\end{matrix}\right.\)
Giúp mình giải các câu hỏi trên trừ 2 câu khoanh tròn vì mình đã làm r giúp mik với :3 cảm ơn nhiều ( lưu ý : giải chi tiết vì mình đang bắt đầu học )