a. 2cos2x – 3cosx + 1 = 0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
11 tháng 3 2019
2cos2x – 3cosx + 1 = 0 (Phương trình bậc hai với ẩn cos x).
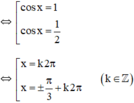
Vậy phương trình có tập nghiệm
8cos(x-pi/3)^3-3cosx-3căn3sinx+2cos2x=0
TK
0
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 7 2020
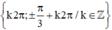
a/ ĐKXĐ: \(cosx\ne-\frac{1}{2}\)
\(\Leftrightarrow2cosx-1=6cosx+3\)
\(\Leftrightarrow4cosx=-4\Rightarrow cosx=-1\)
\(\Rightarrow x=\pi+k2\pi\)
b/
\(\Leftrightarrow cosx\left(2cos2x-1\right)-3cosx=0\)
\(\Leftrightarrow cosx\left(2cos2x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cos2x=2\left(vn\right)\end{matrix}\right.\)
\(\Rightarrow x=\frac{\pi}{2}+k\pi\)
c/
\(\Leftrightarrow2sin2x.cos2x=0\)
\(\Leftrightarrow sin4x=0\)
\(\Rightarrow4x=k\pi\Rightarrow x=\frac{k\pi}{4}\)
NV
Nguyễn Việt Lâm
Giáo viên
15 tháng 7 2020
Với \(x\in\left(-\frac{\pi}{4};\frac{\pi}{2}\right)\Rightarrow cosx>0\Rightarrow3cosx+1>0\)
Do đó pt tương đương:
\(2cos2x-1=0\Rightarrow cos2x=\frac{1}{2}\)
\(\Rightarrow\left[{}\begin{matrix}2x=\frac{\pi}{3}+k2\pi\\2x=-\frac{\pi}{3}+k2\pi\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{6}+k\pi\\x=-\frac{\pi}{6}+k\pi\end{matrix}\right.\)
Pt có 2 nghiệm thuộc khoảng đã cho là \(x=\left\{-\frac{\pi}{6};\frac{\pi}{6}\right\}\)
HP
27 tháng 9 2021
a, \(sin^2x-4sinx+3=0\)
\(\Leftrightarrow\left(sinx-1\right)\left(sinx-3\right)=0\)
\(\Leftrightarrow sinx=1\)
\(\Leftrightarrow x=\dfrac{\pi}{2}+k2\pi\)
HP
27 tháng 9 2021
b, \(2cos^2-cosx-1=0\)
\(\Leftrightarrow\left(cosx-1\right)\left(2cosx+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=1\\cosx=-\dfrac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=\pm\dfrac{2\pi}{3}+k2\pi\end{matrix}\right.\)
NV
Nguyễn Việt Lâm
Giáo viên
9 tháng 10 2020
4.
\(\Leftrightarrow2sinx.cosx-\left(1-2sin^2x\right)+3sinx-cosx-1=0\)
\(\Leftrightarrow cosx\left(2sinx-1\right)+2sin^2x+3sinx-2=0\)
\(\Leftrightarrow cosx\left(2sinx-1\right)+\left(2sinx-1\right)\left(sinx+2\right)=0\)
\(\Leftrightarrow\left(2sinx-1\right)\left(sinx+cosx+2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2sinx-1=0\\sinx+cosx=-2\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\frac{1}{2}\\sin\left(x+\frac{\pi}{4}\right)=-\sqrt{2}< -1\left(l\right)\end{matrix}\right.\)
\(\Leftrightarrow...\)
NV
Nguyễn Việt Lâm
Giáo viên
9 tháng 10 2020
2.
ĐKXĐ: ...
\(\Leftrightarrow cot\left(\frac{\pi}{4}-x\right)=-\frac{1}{\sqrt{3}}\)
\(\Leftrightarrow\frac{\pi}{4}-x=-\frac{\pi}{3}+k\pi\)
\(\Leftrightarrow x=\frac{7\pi}{12}+k\pi\)
3.
\(\Leftrightarrow cos\frac{x}{4}sinx+sin\frac{x}{4}.cosx-3\left(sin^2x+cos^2x\right)+cosx=0\)
\(\Leftrightarrow sin\left(x+\frac{x}{4}\right)=-cosx\)
\(\Leftrightarrow sin\frac{5x}{4}=sin\left(x-\frac{\pi}{2}\right)\)
\(\Leftrightarrow\left[{}\begin{matrix}\frac{5x}{4}=x-\frac{\pi}{2}+k2\pi\\\frac{5x}{4}=\frac{3\pi}{2}-x+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow...\)
2cos^2(x) - 3cosx + 1 = 0
<=> (cosx - 1)(2cosx - 1) = 0
TH1: cosx = 1 <=> x = k.2pi (k ∈ Z)
TH2: 2cosx = 1 <=> cosx = 1/2 <=> x = pi/3 + k.2pi (k ∈ Z)
cảm ơn nhé