Giá trị của a để hàm số y = f ( x ) = x - 1 - 1 x 2 - 3 x + 2 k h i x ≠ 2 2 a + 1 6 k h i x = 2 liên tục tại x = 2
A. 2
B. 1 2
C. 3
D. 1
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

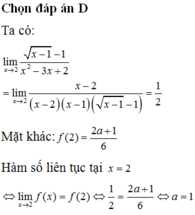

\(f\left(x\right)=\left|x-2015\right|+\left|x+2016\right|\)
a) Ta có: \(\left|x\right|=\orbr{\begin{cases}x=\frac{1}{2}\\x=-\frac{1}{2}\end{cases}}\)
+) Với \(x=\frac{1}{2}\):
\(f\left(\frac{1}{2}\right)=\left|\frac{1}{2}-2015\right|+\left|\frac{1}{2}+2016\right|=2\)
+) Với \(x=-\frac{1}{2}\)
\(f\left(-\frac{1}{2}\right)=\left|-\frac{1}{2}-2015\right|+\left|-\frac{1}{2}+2016\right|=0\)
c) Áp dụng BĐT |x| + |y| \(\ge\)|x + y|, ta được:
\(f\left(x\right)=\left|x-2015\right|+\left|x+2016\right|=\left|2015-x\right|+\left|x+2016\right|\)
\(\ge\left|\left(2015-x\right)+\left(x+2016\right)\right|=\left|4031\right|=4031\)
(Dấu "="\(\Leftrightarrow\left(2015-x\right)\left(x+2016\right)\ge0\)
TH1: \(\hept{\begin{cases}2015-x\ge0\\x+2016\ge0\end{cases}}\Leftrightarrow-2016\le x\le2015\)
TH2: \(\hept{\begin{cases}2015-x\le0\\x+2016\le0\end{cases}}\Leftrightarrow\hept{\begin{cases}x\ge2015\\x\le-2016\end{cases}}\left(L\right)\))
Vậy \(f\left(x\right)_{min}=4031\Leftrightarrow-2016\le x\le2015\)

\(a,f\left(-1\right)=\left(-5\right)\left(-1\right)-3=5-3=2\\ f\left(\dfrac{2}{3}\right)=-5.\dfrac{2}{3}-3=\dfrac{-10}{3}-3=-\dfrac{19}{3}\)
\(b,y=-8\Rightarrow-8=-5x-3\Rightarrow-5=-5x\Rightarrow x=1\\ y=6\Rightarrow6=-5x-3\Rightarrow9=-5x\Rightarrow x=-\dfrac{9}{5}\)
a: f(-1)=5-3=2
f(2/3)=-10/3-3=-19/3
b: y=-8
=>-5x-3=-8
=>-5x=-5
hay x=1
y=6
=>-5x-3=6
=>-5x=9
hay x=-9/5

a: f(-3)=10
f(0)=-8
f(1)=-6
f(2)=0
b: f(x)=0
=>(x-2)(x+2)=0
=>x=2 hoặc x=-2

Câu 1:
a)
| \(y=f\left(x\right)=2x^2\) | -5 | -3 | 0 | 3 | 5 |
| f(x) | 50 | 18 | 0 | 18 | 50 |
b) Ta có: f(x)=8
\(\Leftrightarrow2x^2=8\)
\(\Leftrightarrow x^2=4\)
hay \(x\in\left\{2;-2\right\}\)
Vậy: Để f(x)=8 thì \(x\in\left\{2;-2\right\}\)
Ta có: \(f\left(x\right)=6-4\sqrt{2}\)
\(\Leftrightarrow2x^2=6-4\sqrt{2}\)
\(\Leftrightarrow x^2=3-2\sqrt{2}\)
\(\Leftrightarrow x=\sqrt{3-2\sqrt{2}}\)
hay \(x=\sqrt{2}-1\)
Vậy: Để \(f\left(x\right)=6-4\sqrt{2}\) thì \(x=\sqrt{2}-1\)