Tính giá trị của biểu thức , biết x là nghiệm của phương trình
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

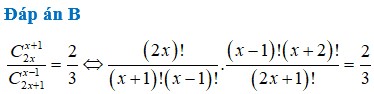
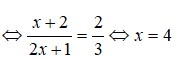

ta có :
\(\sqrt{2}a^2+a-1=0\Leftrightarrow\sqrt{2}a^2=1-a\) nên ta có \(a\le1\)
\(\Rightarrow2a^4=a^2-2a+1\)Vậy \(C=\frac{2a-3}{\sqrt{2\left(a^2-4a+4\right)}+2a^2}=\frac{2a-3}{2a^2+\sqrt{2}\left(2-a\right)}=\frac{2a-3}{\sqrt{2}\left(\sqrt{2}a^2-a+2\right)}\)
\(=\frac{2a-3}{\sqrt{2}\left(1-a-a+2\right)}=\frac{2a-3}{\sqrt{2}\left(3-2a\right)}=-\frac{1}{\sqrt{2}}\)

a là nghiệm nên \(\sqrt{2}a^2+a-1=0\Rightarrow\sqrt{2}a^2=1-a\)
\(\Rightarrow2a^4=\left(1-a\right)^2=a^2-2a+1\)
\(\Rightarrow2a^4-2a+3=a^2-4a+4=\left(a-2\right)^2\)
Mặt khác \(1-a=\sqrt{2}a^2>0\Rightarrow a< 1\)
\(\Rightarrow\sqrt{2\left(2a^4-2a+3\right)}+2a^2=\sqrt{2\left(a-2\right)^2}+2a^2=\sqrt{2}\left(2-a\right)+2a^2\)
\(=\sqrt{2}\left(\sqrt{2}a^2-a+2\right)=\sqrt{2}\left(1-a-a+2\right)=\sqrt{2}\left(3-2a\right)\)
\(\Rightarrow C=\dfrac{2a-3}{\sqrt{2}\left(3-2a\right)}=-\dfrac{\sqrt{2}}{2}\)

\(x^2-4x-6=0\)
\(\text{Δ}=\left(-4\right)^2-4\cdot1\cdot\left(-6\right)=16+24=40>0\)
=>Phương trình này có hai nghiệm phân biệt
Theo vi-et, ta có:
\(x_1+x_2=\dfrac{-b}{a}=\dfrac{-\left(-4\right)}{1}=4;x_1\cdot x_2=\dfrac{c}{a}=\dfrac{-6}{1}=-6\)
\(A=x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2\)
\(=4^2-2\cdot\left(-6\right)=16+12=28\)
\(B=\dfrac{1}{x_1}+\dfrac{1}{x_2}=\dfrac{x_1+x_2}{x_1\cdot x_2}=\dfrac{4}{-6}=-\dfrac{2}{3}\)
\(C=x_1^3+x_2^3\)
\(=\left(x_1+x_2\right)^3-3\cdot x_1\cdot x_2\cdot\left(x_1+x_2\right)\)
\(=4^3-3\cdot4\cdot\left(-6\right)=64+72=136\)
\(D=\left|x_1-x_2\right|\)
\(=\sqrt{\left(x_1-x_2\right)^2}\)
\(=\sqrt{\left(x_1+x_2\right)^2-4x_1x_2}\)
\(=\sqrt{4^2-4\cdot\left(-6\right)}=\sqrt{16+24}=\sqrt{40}=2\sqrt{10}\)