Tìm x, biết: − 9 2 < x < − 4
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
MH
2
KV
6 tháng 11 2023
a) x × 7/9 = 2/3 + 2 1/2
x × 7/9 = 2/3 + 5/2
x × 7/9 = 19/6
x = 19/6 : 7/9
x = 57/14
b) 5/7 + x : 9/4 = 4/3
x : 9/4 = 4/3 - 5/7
x : 9/4 = 13/21
x = 13/21 × 9/4
x = 39/28
6 tháng 11 2023
Một tốp thợ có 120 người dự địnhnlamf trong 50 ngày . Khi bắt đầu làm có một số người đến làm thêm nên làm xong công việc đó trong 30 ngày . Hỏi có bao nhiêu người đến thêm?
30 tháng 6 2021
Ta có:(x-3)(x2+3x+9)-x(x2-4)=1
=> x3-27-x3+4x=1
=>4x=28=>x=7
EN
2
28 tháng 5 2016
a, x^2-4=8(x-2)
=> x^2 - 4 = 8.x - 16
=> x^2 = (8.x - 16) - 4
=> x^2 = 8.x - (16+4)
=> x^2 = 8.x - 20
28 tháng 5 2016
A, \(x^2-4=8\left(x-2\right)\)=> \(\left(x-2\right).\left(x+2\right)=8\left(x-2\right)=>\left(x-2\right).\left(x+2\right)-8\left(x-2\right)=0\)
=>\(\left(x-2\right).\left(x-6\right)=0\)
=> x = 2 hoặc x =6
B. \(x^2-4x+4=9\left(x-2\right)\)=> \(\left(x-2\right)^2=9\left(x-2\right)=>\left(x-2\right)^2-9\left(x-2\right)=0\)
=>\(\left(x-2\right).\left(x-11\right)=0\)=> x =2 hoặc x =11
C. \(4x^2-12x+9=\left(5-x\right)^2=>\left(2x-3\right)^2=\left(5-x\right)^2\)
=>\(\left(2x-3\right)^2-\left(5-x\right)^2=>\left(3x-8\right).\left(x+2\right)=0\)
=> x = 3/8 hoặc x = - 2
8 tháng 8 2023
a. x mũ 2 - 2x + 1 = 25
= x^2 + 2.x.1 + 1^2
= ( x + 1 ) ^2
ko bt có đúng ko nữa, mấy câu kia tui ko bt lm
DH
0
10 tháng 7 2020
Trả lời
\(x.\left(x+2\right).\left(x+4\right).\left(x+6\right)=9\)
\(\Leftrightarrow\left[x.\left(x+6\right)\right].\left[\left(x+2\right).\left(x+4\right)\right]=9\)
\(\Leftrightarrow\left(x^2+6x\right).\left(x^2+6x+8\right)=9\)
Đặt \(x^2+6x=t\) ta có
\(t.\left(t+8\right)=9\)
\(\Leftrightarrow t^2+8t-9=0\)
\(\Leftrightarrow t^2-t+9t-9=0\)
\(\Leftrightarrow t.\left(t-1\right)+9.\left(t-1\right)=0\)
\(\Leftrightarrow\left(t-1\right).\left(t+9\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}t-1=0\\t+9=0\end{cases}\Leftrightarrow}\orbr{\begin{cases}t=1\\t=-9\end{cases}}\)
TH1 \(t=1\)
\(\Rightarrow x^2+6x=1\)
\(\Leftrightarrow x^2+6x-1=0\)
\(\Leftrightarrow x^2+6x+9-10=0\)
\(\Leftrightarrow\left(x+3\right)^2=10=\left(\pm\sqrt{10}\right)^2\)
\(\Leftrightarrow\orbr{\begin{cases}x+3=\sqrt{10}\\x+3=-\sqrt{10}\end{cases}\Leftrightarrow}\orbr{\begin{cases}x=-3+\sqrt{10}\\x=-3-\sqrt{10}\end{cases}}\)
TH2: \(t=-9\)
\(\Rightarrow x^2+6x=-9\)
\(\Leftrightarrow x^2+6x+9=0\)
\(\Leftrightarrow\left(x+3\right)^2=0\)
\(\Leftrightarrow x+3=0\)
\(\Leftrightarrow x=-3\)
Vậy \(x\in\left\{-3+\sqrt{10};-3-\sqrt{10};-3\right\}\)
10 tháng 7 2020
\(x\left(x+2\right)\left(x+4\right)\left(x+6\right)=9\)
\(\Leftrightarrow x^4+12x^3+44x^2+48x=9\)
\(\Leftrightarrow x^4+12x^3+44x^2+48x-9=0\)
\(\Leftrightarrow\left(x^3+9x^2+17x-3\right)\left(x+3\right)=0\)
\(\Leftrightarrow\left(x^2+6x-1\right)\left(x+3\right)^2=0\)
TH1 : Ta có : \(6^2-4.\left(-1\right)=36+4=40>0\)Suy ra : \(x_1=\frac{-6-\sqrt{40}}{2};x_2=\frac{-6+\sqrt{40}}{2}\)
TH2 : \(\left(x+3\right)^2=0\Leftrightarrow x+3=0\Leftrightarrow x=-3\)
17 tháng 7 2021
(1-3x2)-(x-2)(9x+1)=(3x-4)(3x+4)-9(x+3)2
⇒1-3x2-(9x2+x-18x-2)=9x2-16-9(x2+6x+9)
⇒1-3x2-(9x2-17x-2)= -56x-97
⇒1-3x2-9x2+17x+2=-56x-97
⇒3-12x2+17x=-56x-97
⇒3-12x2+17x+56x+97=0
⇒-12x2+73x+100=0
⇒-(12x2-73x-100)=0
DL
9 tháng 5 2017
\(\frac{x+2}{4}=\frac{9}{x+2}\)
\(\Rightarrow\frac{\left(x+2\right)\left(x+2\right)}{4\left(x+2\right)}=\frac{36}{4\left(x+2\right)}\)
\(\Rightarrow x^2+4x+4=36\)
\(\Rightarrow x^2+4x=32\)
\(\Rightarrow x=4;-8\)
\(x\in N\left(4\right)\)(x là số nguyên dương ?)
23 tháng 7 2021
\(\left(2x+1\right)^2-4\left(x+2\right)^2=9\)
\(\Leftrightarrow4x^2+4x+1-4\left(x^2+4x+4\right)=9\)
\(\Leftrightarrow4x^2+4x+1-4x^2-16x-16=9\)
\(\Leftrightarrow-12x-15=9\Leftrightarrow-12x=24\Leftrightarrow x=-2\)
T
1
6 tháng 11 2021
\(a,\Leftrightarrow\left(x-9\right)^2-2\left(x-9\right)+1=0\\ \Leftrightarrow\left(x-9-1\right)^2=0\Leftrightarrow x=10\\ b,Sửa:49x^2-14x\sqrt{5}+5=0\\ \Leftrightarrow\left(7x-\sqrt{5}\right)^2=0\Leftrightarrow x=\dfrac{\sqrt{5}}{7}\)
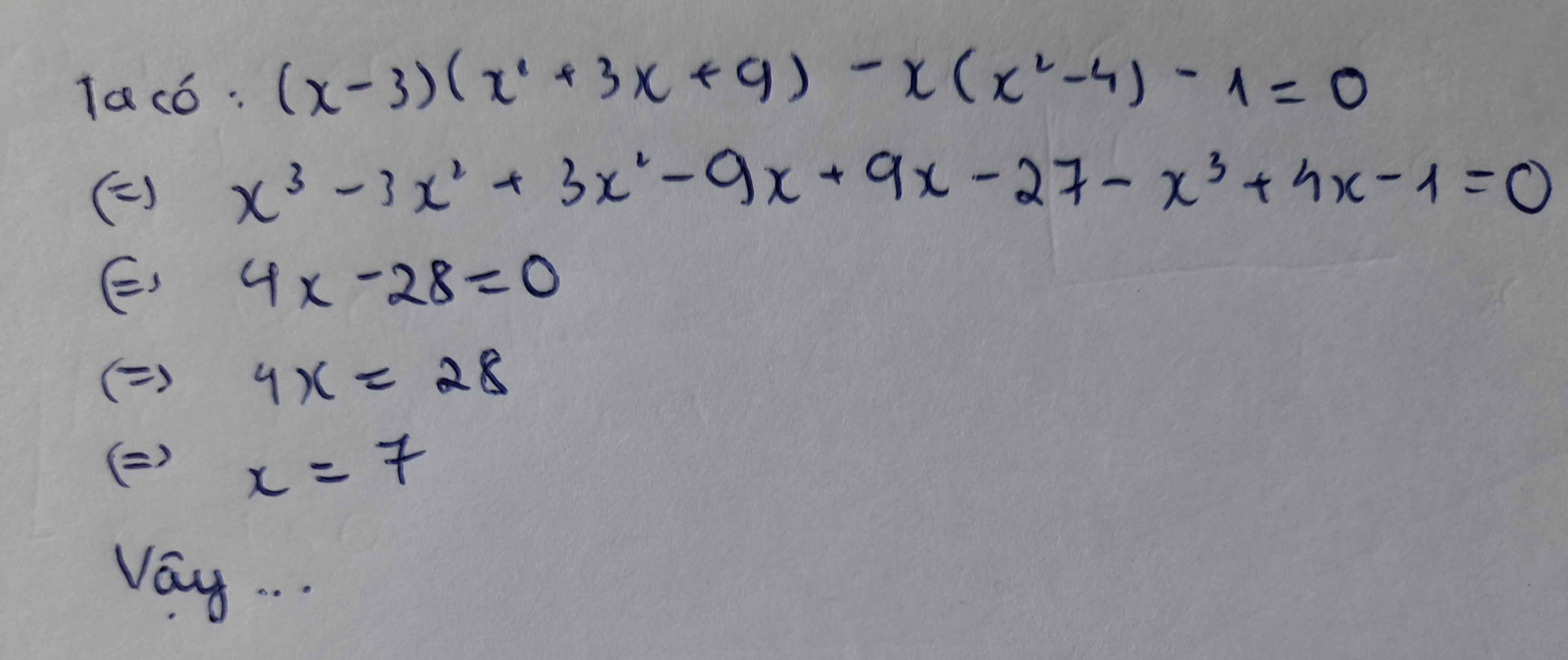
− 9 2 < x < − 4 x = - 4