Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
1.
Đặt \(\sqrt{x^2-4x+5}=t\ge1\Rightarrow x^2-4x=t^2-5\)
Pt trở thành:
\(4t=t^2-5+2m-1\)
\(\Leftrightarrow t^2-4t+2m-6=0\) (1)
Pt đã cho có 4 nghiệm pb khi và chỉ khi (1) có 2 nghiệm pb đều lớn hơn 1
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta'=4-\left(2m-6\right)>0\\\left(t_1-1\right)\left(t_2-1\right)>0\\\dfrac{t_1+t_2}{2}>1\\\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}10-2m>0\\t_1t_2-\left(t_1+t_1\right)+1>0\\t_1+t_2>2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 5\\2m-6-4+1>0\\4>2\end{matrix}\right.\) \(\Leftrightarrow\dfrac{9}{2}< m< 5\)
2.
Để pt đã cho có 2 nghiệm:
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne3\\\Delta'=1+4\left(m-3\right)\ge0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne3\\m\ge\dfrac{11}{4}\end{matrix}\right.\)
Khi đó:
\(x_1^2+x_2^2=4\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=4\)
\(\Leftrightarrow\dfrac{4}{\left(m-3\right)^2}+\dfrac{8}{m-3}=4\)
\(\Leftrightarrow\dfrac{1}{\left(m-3\right)^2}+\dfrac{2}{m-3}-1=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{1}{m-3}=-1-\sqrt{2}\\\dfrac{1}{m-3}=-1+\sqrt{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}m=4-\sqrt{2}< \dfrac{11}{4}\left(loại\right)\\m=4+\sqrt{2}\end{matrix}\right.\)
\(x-4\sqrt{x+3}+m=0\)
\(\Leftrightarrow x+3-4\sqrt{x+3}-3+m=0\left(1\right)\)
\(đăt:\sqrt{x+3}=t\left(t\ge0\right)\)
\(\left(1\right)\Leftrightarrow t^2-4t-3+m=0\Leftrightarrow f\left(t\right)=t^2-4t-3=-m\left(2\right)\)
\(\left(1\right)-có-2ngo-phân-biệt\Leftrightarrow\left(2\right)có-2ngo-phân-biệt-thỏa:t\ge0\)
\(\Rightarrow f\left(0\right)=-3\)
\(\Rightarrow f\left(t\right)min=\dfrac{-\Delta}{4a}=-7\Leftrightarrow t=2\)
\(\Rightarrow-7< -m\le-3\Leftrightarrow3\le m< 7\)
\(t^2-4t-3+m=0\Leftrightarrow t^2-4t-3=-m\)
\(có-2nghiệm-pb-trên[0;\text{+∞})\)
\(xét-bảng-biến-thiên-củaf\left(t\right)=t^2-4t-3,trên[0;\text{+∞})\)
dựa vào bảng biến thiên ta thấy số nghiệm của phương trình f(t)
là số giao điểm của đường thẳng y=-m
\(\Rightarrow-7< -m\le-3\Leftrightarrow3\le m< 7\)
Để pt có 2 nghiệm phân biệt thì \(\Delta'=m^2-\left(m+2\right)>0\Leftrightarrow\left(m+1\right)\left(m-2\right)>0\Leftrightarrow\left[{}\begin{matrix}m>2\\m< -1\end{matrix}\right.\). (1)
Khi đó theo hệ thức Viète ta có \(\left\{{}\begin{matrix}x_1+x_2=2m\\x_1x_2=m+2\end{matrix}\right.\).
Ta có \(x_1^3+x_2^3=\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)=\left(2m\right)^3-3.2m.\left(m+2\right)=8m^3-6m^2-12m\).
Do đó \(8m^3-6m^2-12m\le16\Leftrightarrow\left(m-2\right)\left(8m^2+10m+8\right)\le0\Leftrightarrow m\le2\)
(do \(8m^2+10m+8=2\left(2m+\dfrac{5}{4}\right)^2+\dfrac{39}{8}>0\forall m\)).
Kết hợp vs (1) ta có m < -1.
- Đặt \(a=x^2-2x\left(a\ge-1\right)\)
PTTT \(3\sqrt{a+3}=a+m\left(a\ge-m\right)\)
\(\Leftrightarrow9\left(a+3\right)=\left(a+m\right)^2=a^2+2am+m^2=9a+27\)
\(\Leftrightarrow a^2+a\left(2m-9\right)+m^2-27=0\)
Có : \(\Delta=\left(2m-9\right)^2-4\left(m^2-27\right)=4m^2-36m+81-4m^2+108\)
\(=-36m+189\)
- Để phương trình đề có 2 nghiệm phân biệt :
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta>0\\\left(a_1+1\right)\left(a_2+1\right)>0\\a_1+1+a_2+1>0\end{matrix}\right.\)
Lại có : Theo vi ét : \(\left\{{}\begin{matrix}a_1+a_2=-2m+9\\a_1a_2=m^2-27\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\Delta>0\\a_1a_2+a_1+a_2+1>0\\a_1+a_2+2>0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}-36m+189>0\\m^2-27-2m+9+1=m^2-2m-17>0\\-2m+9+2=-2m+11>0\end{matrix}\right.\)
\(\Rightarrow m=\left(-\infty;1-3\sqrt{2}\right)\cup\left(1+3\sqrt{2};\dfrac{21}{4}\right)\) ( * )
- Có : \(x^2-2x=a\)
- Đặt \(f\left(x\right)=x^2-2x\)
- Ta có đồ thị \(x^2-2x=0\)
- Từ độ thị hàm số : Để phương trình \(x^2-2x=a\) có 2 nghiệm phân biệt trong đoạn 0, 3 thì \(a=(-1;0]\)
Lại có : \(a=[-m;+\infty)\)
\(\Rightarrow-m\le0\)
\(\Rightarrow m\ge0\)
- Kết hợp với ( * )
\(\Rightarrow m\in\left(1+3\sqrt{2};\dfrac{21}{4}\right)\)
Vậy ...
\(PT\Leftrightarrow x^2+2x+3x=4x^2+4x+1\\ \Leftrightarrow3x^2+2x+1-3m=0\\ \text{PT có 2 nghiệm pb}\Leftrightarrow\Delta'>0\\ \Leftrightarrow1-3\left(1-3m\right)>0\\ \Leftrightarrow1+9m-1>0\Leftrightarrow m>0\)
\(PT\Leftrightarrow x^2+2x+3m=4x^2+4x+1\\ \Leftrightarrow3x^2+2x+1-3m=0\)
PT có 2 nghiệm phân biệt \(\Leftrightarrow\Delta'=1-3\left(1-3m\right)>0\)
\(\Leftrightarrow9m-2>0\\ \Leftrightarrow m>\dfrac{2}{9}\)
Vậy PT có 2 nghiệm pb \(\Leftrightarrow m>\dfrac{2}{9}\)
Trường hợp 1: \(m\ne\pm2\)
Để phương trình có đúng hai nghiệm phân biệt thì phương trình này sẽ có hai nghiệm trái dấu
=>\(m^2-4< 0\)
hay -2<m<2
Trường hợp 2: m=2
Pt sẽ là 1=0(vô lý)
Trường hợp 3: m=-2
=>-4x2+1=0(nhận)
Vậy: -2<=m<2
Số nghiệm của phương trình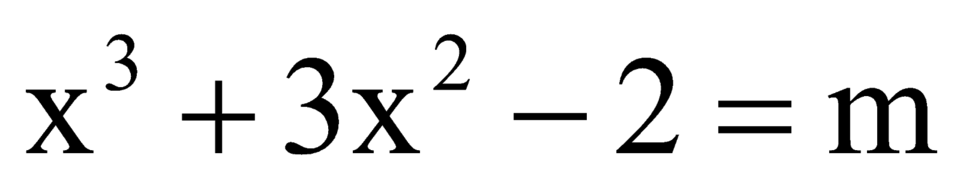
là số giao điểm của đồ thị hàm số 
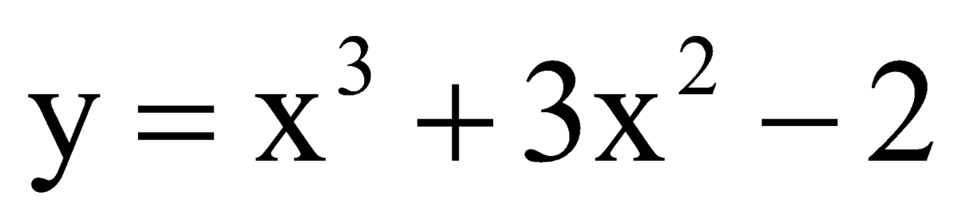và đường thẳng y=m .
Ta có: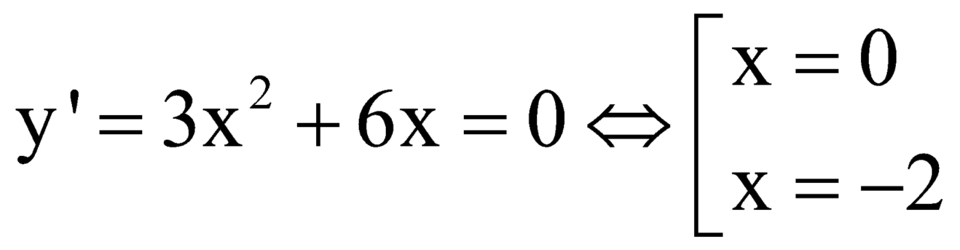
.
Ta có đồ thị hàm số như hình vẽ:
Quan sát đồ thị hàm số ta có: đường thẳng y=m cắt đồ thị hàm số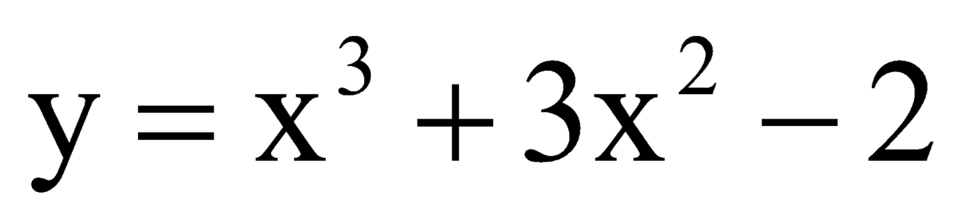
tại 2 điểm phân biệt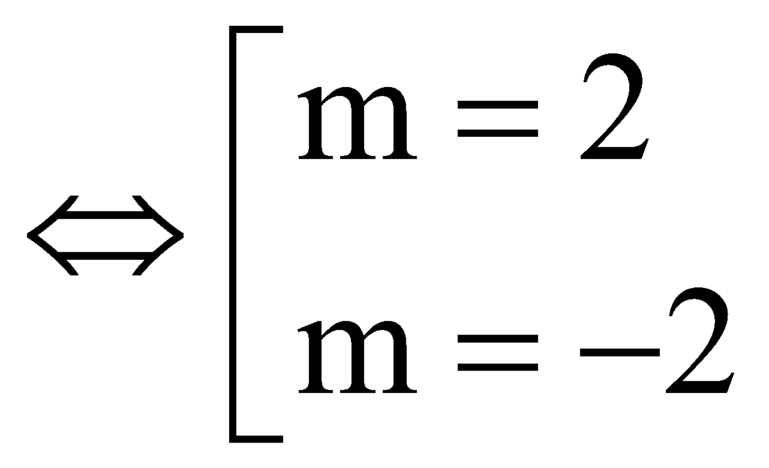 
Chọn D.
Chú ý khi giải: Để làm bài nhanh hơn, các em có thể vẽ BBT thay cho đồ thị hàm số.