Tập xác định của hàm số y = x - 1 là
A. (-∞;1]
B. (1;+∞)
C. [1;+∞)
D. R
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

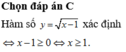

ĐKXĐ:
a. \(\left\{{}\begin{matrix}x-1\ge0\\x-3\ne0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x\ge1\\x\ne3\end{matrix}\right.\) \(\Rightarrow D=[1;+\infty)\backslash\left\{3\right\}\)
b. \(D=R\)
c. \(x+3>0\Rightarrow x>-3\Rightarrow D=\left(-3;+\infty\right)\)
d. \(\left|x-2\right|\ge0\Rightarrow x\in R\Rightarrow D=R\)

Chọn C.
Phương pháp : Kiểm tra tính đúng sai của từng mệnh đề.
Cách giải :
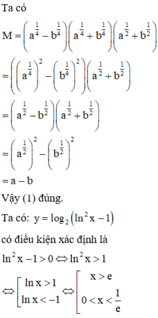
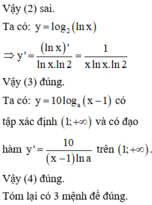

1.
ĐKXĐ: \(\left\{{}\begin{matrix}cosx\ne0\\tanx-sinx\ne0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}cosx\ne0\\\dfrac{sinx}{cosx}-sinx\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}cosx\ne0\\sinx\ne0\\cosx\ne1\end{matrix}\right.\) \(\Leftrightarrow sin2x\ne0\Leftrightarrow x\ne\dfrac{k\pi}{2}\)
2.
ĐKXĐ: \(sin2x\ne0\Leftrightarrow x\ne\dfrac{k\pi}{2}\)
3.
ĐKXĐ: \(\left\{{}\begin{matrix}sin\left(x-\dfrac{\pi}{4}\right)\ne0\\cos\left(x-\dfrac{\pi}{4}\right)\ne0\end{matrix}\right.\)
\(\Leftrightarrow sin\left(2x-\dfrac{\pi}{2}\right)\ne0\Leftrightarrow cos2x\ne0\)
\(\Leftrightarrow x\ne\dfrac{\pi}{4}+\dfrac{k\pi}{2}\)

ĐKXĐ: \(\left\{{}\begin{matrix}2x+a\ge0\\2a-1-x\ge0\end{matrix}\right.\) \(\Rightarrow-\dfrac{a}{2}\le x\le2a-1\)
Miền xác định là đoạn có độ dài 1 khi:
\(2a-1-\left(-\dfrac{a}{2}\right)=1\)
\(\Rightarrow a=\dfrac{4}{5}\)

Đáp án là B
• Hàm số y = sin x ; y = cos x có tập xác định D = ℝ .
• Hàm số y = tan x & y = cot x có tập xác định lần lượt D = ℝ \ π 2 + k π ; D = ℝ \ k π .

ĐKXĐ: \(\left\{{}\begin{matrix}2x-a\ge0\\2a-1-x\ge0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x\ge\dfrac{a}{2}\\x\le2a-1\end{matrix}\right.\)
Miền xác định là 1 đoạn có độ dài bằng 1 khi:
\(2a-1-\dfrac{a}{2}=1\Rightarrow a=\dfrac{4}{3}\)