Giả sử . Tính .
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

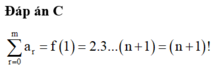

đoạn sau là x2-ax-1/(2a2)=0 nha, viết thiếu.
@nguyenthanhtuan cái này là chứng minh mà bạn.


Từ giả thiết ta có thể viết \(f\left(x\right)=g\left(x\right)\left(x+1\right)+5\) (1)
Và \(f\left(x\right)=h\left(x\right)\left(x-2\right)+7\) (2)
Do (x + 1)(x - 2) là đa thức bậc 2 nên số dư là đa thức bậc 1. Tức là:
\(f\left(x\right)=\left(x+1\right)\left(x-2\right)t\left(x\right)+ax+b\) (Với g(x) , h(x), t(x) là các đa thức)
Ta có \(f\left(x\right)=\left(x+1\right)\left(x-2\right)t\left(x\right)+a\left(x+1\right)+b-a=\left(x+1\right)\left[\left(x-2\right)t\left(x\right)+a\right]+b-a\)
Theo (1) thì b - a = 5.
Ta cũng có :
\(f\left(x\right)=\left(x+1\right)\left(x-2\right)t\left(x\right)+a\left(x-2\right)+b+2a=\left(x-2\right)\left[\left(x+1\right)t\left(x\right)+a\right]+b+2a\)
Theo (2) thì b + 2a = 7.
Từ đó ta tìm được \(a=\frac{2}{3};b=\frac{17}{3}\)

\(a)sin^4x+cos^4x=1-2sin^2x\cdot cos^2x\)
\(\Leftrightarrow sin^4x+2sin^2x\cdot cos^2x+cos^4x=1\)
\(\Leftrightarrow\left(sin^2x+cos^2x\right)^2=1\)(luôn đúng)
a) \sin ^{4} x+\cos ^{4} x=\sin ^{4} x+\cos ^{4} x+2 \sin ^{2} x \cos ^{2} x-2 \sin ^{2} x \cos ^{2} xsin4x+cos4x=sin4x+cos4x+2sin2xcos2x−2sin2xcos2x
\begin{aligned}&=\left(\sin ^{2} x+\cos ^{2} x\right)^{2}-2 \sin ^{2} x \cos ^{2} x \\&=1-2 \sin ^{2} x \cos ^{2} x\end{aligned}=(sin2x+cos2x)2−2sin2xcos2x=1−2sin2xcos2x
b) \dfrac{1+\cot x}{1-\cot x}=\dfrac{1+\dfrac{1}{\tan x}}{1-\dfrac{1}{\tan x}}=\dfrac{\dfrac{\tan x+1}{\tan x}}{\dfrac{\tan x-1}{\tan x}}=\dfrac{\tan x+1}{\tan x-1}1−cotx1+cotx=1−tanx11+

Bài 2:
a: \(A=\frac{a+4\sqrt{a}+4}{\sqrt{a}+2}+\frac{4-a}{\sqrt{a}-2}\)
\(=\frac{\left(\sqrt{a}+2\right)^2}{\sqrt{a}+2}-\frac{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}{\sqrt{a}-2}\)
\(=\left(\sqrt{a}+2\right)-\left(\sqrt{a}+2\right)=0\)
b: \(B=\frac{x\sqrt{x}+y\sqrt{y}}{\sqrt{x}+\sqrt{y}}:\left(\sqrt{x}-\sqrt{y}\right)^2\)
\(=\frac{\left(\sqrt{x}+\sqrt{y}\right)\left(x-\sqrt{xy}+y\right)}{\sqrt{x}+\sqrt{y}}\cdot\frac{1}{\left(\sqrt{x}-\sqrt{y}\right)^2}=\frac{x-\sqrt{xy}+y}{\left(\sqrt{x}-\sqrt{y}\right)^2}\)
Bài 1:
a: \(A=\sqrt{\frac{x-2\sqrt{x}+1}{x+2\sqrt{x}+1}}\)
\(=\sqrt{\frac{\left(\sqrt{x}-1\right)^2}{\left(\sqrt{x}+1\right)^2}}=\left|\frac{\sqrt{x}-1}{\sqrt{x}+1}\right|=\frac{\left|\sqrt{x}-1\right|}{\sqrt{x}+1}\)
b: \(B=\frac{x-1}{\sqrt{y}-1}\cdot\sqrt{\frac{\left(y-2\sqrt{y}+1\right)^2}{\left(x-1\right)^4}}\)
\(=\frac{\left(x-1\right)}{\sqrt{y}-1}\cdot\frac{\left|y-2\sqrt{y}+1\right|}{\left|\left(x-1\right)^2\right|}\)
\(=\left(x-1\right)\cdot\frac{\left(\sqrt{y}-1\right)^2}{\left(\sqrt{y}-1\right)\left(x-1\right)^2}=\frac{\left(\sqrt{y}-1\right)}{x-1}\)