a) Tính giá trị biểu thức
4 9 × 3 2 : 2 3
b) Tìm x
1 3 : x = 1 5
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

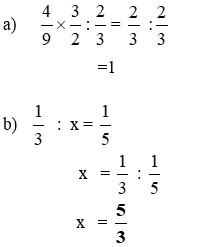

a, ĐKXĐ: x≠±3
A=\(\left(\dfrac{3-x}{x+3}.\dfrac{x^2+6x+9}{x^2-9}+\dfrac{x}{x+3}\right):\dfrac{3x^2}{x+3}\)
A=\(\left(\dfrac{3-x}{x+3}.\dfrac{\left(x+3\right)^2}{\left(x+3\right)\left(x-3\right)}+\dfrac{x}{x+3}\right):\dfrac{3x^2}{x+3}\)
A=\(\left(\dfrac{3-x}{x-3}+\dfrac{x}{x+3}\right):\dfrac{3x^2}{x+3}\)
A=\(\left(\dfrac{9-x^2}{x^2-9}+\dfrac{x^2-3x}{x^2-9}\right):\dfrac{3x^2}{x+3}\)
A=\(\left(\dfrac{-3}{x+3}\right):\dfrac{3x^2}{x+3}\)
A=\(\dfrac{-1}{x^2}\)
b, Thay x=\(-\dfrac{1}{2}\) (TMĐKXĐ) vào A ta có:
\(\dfrac{-1}{\left(-\dfrac{1}{2}\right)^2}\)=-4
c, A<0 ⇔ \(\dfrac{-1}{x^2}< 0\) ⇔ x2>0 (Đúng với mọi x)
Vậy để A<0 thì x đúng với mọi giá trị (trừ ±3)

a)a+b+c=9
=>(a+b+c)2=81
=>a2+b2+c2+2ab+2bc+2ca=81
Từ a2+b2+c2=141=>2ab+2bc+2ca=81-141=-60
=>2(ab+bc+ca)=-60=>ab+bc+ca=-30
b)x+y=1
=>(x+y)3=1
=>x3+3x2y+3xy2+y3=1
=>x3+y3+3xy(x+y)=1
=>x3+y3+3xy=1(Do x+y=1)
c)a3-3ab+2c=(x+y)3-3(x+y)(x2+y2)+2(x3+y3)
=x3+3x2y+3xy2+y3-3x3-3y3-3x2y-3xy2+2x3+2y3=0
d)đang tìm hướng giải

C = 59/10 : 3/2 - ( 5/3 x 9/2 x 14/3) : 7/4
= 59/15 - 35 : 7/4
= 59/15 - 20
= -241/15

Bài 6:
a2+b2=(a+b)2-2ab
<=> 2010 =36-2ab
<=>ab=-987
M=a3+b3
=(a+b)(a2-ab+b2)
=6(a2+987+b^2)
=6(2010+987)
=17982

điều kiện xác định của phân thức là x khác 0 và x khác -3
nên bạn nhập phân thức vào máy rồi thay x =3 ta có P =1/6

a, ĐKXĐ: \(x\ne1;x\ne-1\)
b, Với \(x\ne1;x\ne-1\)
\(B=\left[\dfrac{x+1}{2\left(x-1\right)}+\dfrac{3}{\left(x-1\right)\left(x+1\right)}-\dfrac{x+3}{2\left(x+1\right)}\right]\cdot\dfrac{4\left(x^2-1\right)}{5}\\ =\left[\dfrac{x^2+2x+1+6-x^2-2x+3}{2\left(x-1\right)\left(x+1\right)}\right]\cdot\dfrac{4\left(x^2-1\right)}{5}\\ =\dfrac{5}{x^2-1}\cdot\dfrac{4\left(x^2-1\right)}{5}\\ =4\)
=> ĐPCM

a, Thay x = -2 ; y = 3 ta được
\(A=\dfrac{4\left(-2\right)-5.3}{8\left(-2\right)-7.3}=\dfrac{-8-15}{-16-21}=\dfrac{23}{37}\)
b, Ta có \(\dfrac{x}{y}=\dfrac{5}{4}\Rightarrow\dfrac{x}{5}=\dfrac{y}{4}=k\Rightarrow x=5k;y=4k\)
Thay vào ta được \(A=\dfrac{4.5k-5.4k}{8.5k-7.4k}=\dfrac{0}{40k-28k}=0\)