Trên mặt phẳng tọa độ Oxy, cho điểm B(2; 4). Hãy xác định vị trí tương đối của đường tròn (B; 3) và các trục tọa độ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\text{Đặt }M\left(x;y\right)\\ \overrightarrow{MB}\left(-2-x,2-y\right);\overrightarrow{MC}\left(-x,1-y\right)\\ \left|\overrightarrow{MB}\right|=\left|2\overrightarrow{MC}\right|\Leftrightarrow\sqrt{\left(-2-x\right)^2+\left(2-y\right)^2}=2\sqrt{\left(-x\right)^2+\left(1-y\right)^2}\\ \Leftrightarrow x^2+4x+4+y^2-4y+4=2x^2+2y^2-4y+2\\ \Leftrightarrow x^2+y^2-4y-6=0\\ \text{Mà }M\in Ox\Leftrightarrow y=0\Leftrightarrow x^2-6=0\Leftrightarrow\left[{}\begin{matrix}x=\sqrt{6}\\x=-\sqrt{6}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}M\left(\sqrt{6};0\right)\\M\left(-\sqrt{6};0\right)\end{matrix}\right.\)

Giả sử tọa độ M(x;0). Khi đó \(\overrightarrow{MA}=\left(1-x;2\right);\overrightarrow{MB}=\left(4-x;3\right)\)
Theo giả thiết ta có \(\overrightarrow{MA}.\overrightarrow{MB}=MA.MB.\cos45^0\)
\(\Leftrightarrow\left(1-x\right)\left(4-x\right)+6=\sqrt{\left(1-x\right)^2+4}.\sqrt{\left(4-x\right)^2+9}.\frac{\sqrt{2}}{2}\)
\(\Leftrightarrow x^2-5x+10=\sqrt{x^2-2x+5}.\sqrt{x^2-8x+25}.\frac{\sqrt{2}}{2}\)
\(\Leftrightarrow2\left(x^2-5x+10\right)^2=\left(x^2-5x+10\right)\left(x^2-8x+25\right)\) (do \(x^2-5x+10>0\))
\(\Leftrightarrow x^4-10x^3+44x^2-110x+75=0\)
\(\Leftrightarrow\left(x-1\right)\left(x-5\right)\left(x^2-4x+15\right)=0\)
\(\Leftrightarrow x=1;x=5\)
Vậy ta có 2 điểm cần tìm là M(1;0) hoặc M(5;0)

Chắc là A,B,M thẳng hàng chứ?
Do M thuộc Oy nên tọa độ có dạng: \(M\left(0;m\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{BA}=\left(2;5\right)\\\overrightarrow{BM}=\left(1;m+2\right)\end{matrix}\right.\)
A, B, M thẳng hàng \(\Rightarrow\overrightarrow{BA}\) cùng phương \(\overrightarrow{BM}\)
\(\Rightarrow\dfrac{1}{2}=\dfrac{m+2}{5}\Rightarrow m=\dfrac{1}{2}\)
\(\Rightarrow M\left(0;\dfrac{1}{2}\right)\)

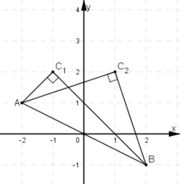
B đối xứng với A qua O ⇒ O là trung điểm của AB
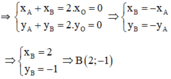
C có tung độ bằng 2 nên C(x; 2)
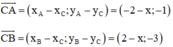
Tam giác ABC vuông tại C
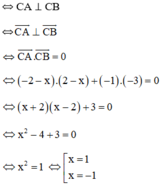
Vậy có hai điểm C thỏa mãn là C1(1; 2) và C2(–1; 2).

a.
\(\left\{{}\begin{matrix}x_I=\dfrac{x_A+x_B}{2}=\dfrac{2-4}{2}=-1\\y_I=\dfrac{y_A+y_B}{2}=\dfrac{1+5}{2}=3\end{matrix}\right.\)
\(\Rightarrow I\left(-1;3\right)\)
b.
Do C thuộc trục hoành, gọi tọa độ C có dạng \(C\left(c;0\right)\)
Do D thuộc trục tung, gọi tọa độ D có dạng \(D\left(0;d\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{AC}=\left(c-2;-1\right)\\\overrightarrow{DB}=\left(-4;5-d\right)\Rightarrow2\overrightarrow{DB}=\left(-8;10-2d\right)\end{matrix}\right.\)
Để \(\overrightarrow{AC}=2\overrightarrow{DB}\)
\(\Leftrightarrow\left\{{}\begin{matrix}c-2=-8\\-1=10-2d\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}c=-6\\d=\dfrac{11}{2}\end{matrix}\right.\)
Vậy \(C\left(-6;0\right)\) và \(D\left(0;\dfrac{11}{2}\right)\)

\(\overrightarrow{AB}=\left(-3;7\right)\)
\(\overrightarrow{DC}=\left(1-x_D;5-y_D\right)\)
Để ABCD là hbh thì
\(\left\{{}\begin{matrix}1-x_D=-3\\5-y_D=7\end{matrix}\right.\Leftrightarrow D\left(2;-2\right)\)
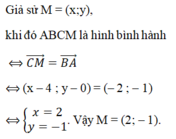
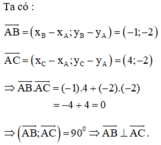
(B) Cắt Oy tại hai điểm phân biệt và (B) không cắt Ox