Tìm y: a) 5 16 + y = 1 2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
CM
Cao Minh Tâm
7 tháng 7 2019
Đúng(0)
Những câu hỏi liên quan
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
15 tháng 8 2023
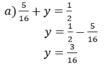
a, y \(\times\) \(\dfrac{4}{3}\) = \(\dfrac{16}{9}\)
y = \(\dfrac{16}{9}\) : \(\dfrac{4}{3}\)
y = \(\dfrac{4}{3}\)
b, ( y - \(\dfrac{1}{2}\)) + 0,5 = \(\dfrac{3}{4}\)
y - 0,5 + 0,5 = \(\dfrac{3}{4}\)
y = \(\dfrac{3}{4}\)
c, \(\dfrac{4}{5}-\dfrac{2}{5}y\) = 0,2
0,8 - 0,4y = 0,2
0,4y = 0,8 - 0,2
0,4y = 0,6
y = 1,5
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
15 tháng 8 2023
d, (y + \(\dfrac{3}{4}\)) \(\times\) \(\dfrac{5}{7}\) = \(\dfrac{10}{9}\)
y + \(\dfrac{3}{4}\) = \(\dfrac{10}{9}\) : \(\dfrac{5}{7}\)
y + \(\dfrac{3}{4}\) = \(\dfrac{14}{9}\)
y = \(\dfrac{14}{9}\) - \(\dfrac{3}{4}\)
y = \(\dfrac{29}{36}\)
e, y : \(\dfrac{5}{4}\) = \(\dfrac{9}{5}\) + \(\dfrac{1}{2}\)
y : \(\dfrac{5}{4}\) = \(\dfrac{23}{10}\)
y = \(\dfrac{23}{10}\)
y = \(\dfrac{23}{8}\)
f, y \(\times\) \(\dfrac{1}{2}\) + \(\dfrac{3}{2}\) \(\times\) y = \(\dfrac{4}{5}\)
y \(\times\) ( \(\dfrac{1}{2}+\dfrac{3}{2}\)) = \(\dfrac{4}{5}\)
2y = \(\dfrac{4}{5}\)
y = \(\dfrac{2}{5}\)
AH
Akai Haruma
Giáo viên
7 tháng 3 2023
a. Áp dụng tính chất dãy tỉ số bằng nhau:
$\frac{x}{2}=\frac{y}{5}=\frac{x+y}{2+5}=\frac{-21}{7}=-3$
$\Rightarrow x=2(-3)=-6; y=5(-3)=-15$
b. Áp dụng tính chất dãy tỉ số bằng nhau:
$7x=3y=\frac{x}{\frac{1}{7}}=\frac{y}{\frac{1}{3}}=\frac{x-y}{\frac{1}{7}-\frac{1}{3}}=\frac{16}{\frac{-4}{21}}=-84$
$\Rightarrow x=(-84):7=-12; y=-84:3=-28$
AH
Akai Haruma
Giáo viên
7 tháng 3 2023
c. $\frac{x}{y}=\frac{5}{9}\Rightarrow \frac{x}{5}=\frac{y}{9}$
Áp dụng tính chất dãy tỉ số bằng nhau:
$\frac{x}{5}=\frac{y}{9}=\frac{3x}{15}=\frac{2y}{18}=\frac{3x+2y}{15+18}=\frac{66}{33}=2$
$\Rightarrow x=2.5=10; y=9.2=18$
d. Áp dụng tính chất dãy tỉ số bằng nhau:
$\frac{x}{15}=\frac{y}{7}=\frac{2y}{14}=\frac{x-2y}{15-14}=\frac{16}{1}=16$
$\Rightarrow x=16.15=240; y=7.16=112$
e.
Đặt $\frac{x}{5}=\frac{y}{2}=k\Rightarrow x=5k ; y=2k$
Khi đó: $xy=5k.2k=10k^2=1000\Rightarrow k^2=100\Rightarrow k=\pm 10$
Với $k=10$ thì $x=5k=50; y=2k=20$
Với $k=-10$ thì $x=5k=-50; y=2k=-20$
YN
5 tháng 10 2021
a) \(\left(3x-5\right)\left(5-3x\right)+9\left(x+1\right)^2=30\)
\(\Rightarrow15x-9x^2-25+15x+9\left(x^2+2x+1\right)-30=0\)
\(\Rightarrow30x-9x^2-25+9x^2+18x+9-30=0\)
\(\Rightarrow48x-46=0\)
\(\Rightarrow x=\frac{23}{24}\)
b) \(\left(x+4\right)^2-\left(x+1\right)\left(x-1\right)=16\)
\(\Rightarrow\left(x^2+8x+16\right)-\left(x^2-1\right)=16\)
\(\Rightarrow x^2+8x+16-x^2+1=16\)
\(\Rightarrow8x+17=16\)
\(\Rightarrow8x=-1\)
\(\Rightarrow x=\frac{-1}{8}\)
YN
5 tháng 10 2021
c) \(\left(y-2\right)^3-\left(y-3\right)\left(y^2+3y+9\right)+6\left(y+1\right)^2=49\)
\(\Rightarrow\left(y-2\right)^3-\left(y^3-3^3\right)+6\left(y^2+2y+1\right)=49\)
\(\Rightarrow y^3-6y^2+12y-8-y^3+27+6y^2+12y+6=49\)
\(\Rightarrow\left(y^3-y^3\right)+\left(-6y^2+6y^2\right)+\left(12y+12y\right)+\left(-8+27+6\right)=49\)
\(\Rightarrow24y+25=49\)
\(\Rightarrow24y=24\)
\(\Rightarrow y=1\)
d) \(\left(y+3\right)^3-\left(y+1\right)^3=56\)
\(\Rightarrow\left(y+3-y-1\right)[\left(y+3\right)^2+\left(y+3\right)\left(y+1\right)+\left(y+1\right)^2]=56\)
\(\Rightarrow2\left(y^2+6y+9+y^2+4y+3+y^2+2y+1\right)=56\)
\(\Rightarrow3y^2+12y+13=28\)
\(\Rightarrow\left(3y^2+15y\right)-\left(3y+15\right)=0\)
\(\Rightarrow3y\left(y+5\right)-3\left(y+5\right)=0\)
\(\Rightarrow3\left(y-1\right)\left(y+5\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x-1=0\\x+5=0\end{cases}}\Rightarrow\orbr{\begin{cases}x=1\\x=-5\end{cases}}\)
VN
1
3 tháng 7 2021
Giải:
a) \(\dfrac{-5}{8}=\dfrac{x}{16}\)
\(\Rightarrow x=\dfrac{16.-5}{8}=-10\)
\(\dfrac{3x}{9}=\dfrac{2}{6}\)
\(\Rightarrow3x=\dfrac{2.9}{6}=3\)
\(\Rightarrow x=1\)
b) \(\dfrac{x+3}{15}=\dfrac{1}{3}\)
\(\Rightarrow x+3=\dfrac{1.15}{3}=5\)
\(\Rightarrow x=2\)
\(\dfrac{6}{2x+1}=\dfrac{2}{7}\)
\(\Rightarrow2x+1=\dfrac{6.7}{2}=21\)
\(\Rightarrow x=10\)
c) \(\dfrac{4}{x-6}=\dfrac{y}{24}=\dfrac{-12}{18}\)
\(\Rightarrow\dfrac{4}{x-6}=\dfrac{-12}{18}\)
\(\Rightarrow x-6=\dfrac{18.4}{-12}=-6\)
\(\Rightarrow x=0\)
\(\Rightarrow\dfrac{y}{24}=\dfrac{-12}{18}\)
\(\Rightarrow y=\dfrac{-12.24}{18}=-16\)
\(\dfrac{3-x}{-12}=\dfrac{16}{y+1}=\dfrac{192}{-72}\)
\(\Rightarrow\dfrac{3-x}{-12}=\dfrac{192}{-72}\)
\(\Rightarrow3-x=\dfrac{192.-12}{-72}=32\)
\(\Rightarrow x=-29\)
\(\Rightarrow\dfrac{16}{y+1}=\dfrac{192}{-72}\)
\(\Rightarrow y+1=\dfrac{16.-72}{192}=-6\)
d) \(\dfrac{-2}{3}< \dfrac{x}{5}< \dfrac{-1}{6}\)
\(\Rightarrow\dfrac{-20}{30}< \dfrac{6x}{30}< \dfrac{-5}{30}\)
\(\Rightarrow6x\in\left\{-18;-12;-6\right\}\)
\(\Rightarrow x\in\left\{-3;-2;-1\right\}\)
\(\dfrac{-1}{5}\le\dfrac{x}{8}\le\dfrac{1}{4}\)
\(\Rightarrow\dfrac{-8}{40}\le\dfrac{5x}{40}\le\dfrac{10}{40}\)
\(\Rightarrow5x\in\left\{-5;0;5;10\right\}\)
\(\Rightarrow x\in\left\{-1;0;1;2\right\}\)
e) \(\dfrac{x+46}{20}=x\dfrac{2}{5}\)
\(\Rightarrow\dfrac{x+46}{20}=x+\dfrac{2}{5}\)
\(\Rightarrow\dfrac{x+46}{20}=\dfrac{5x+2}{5}\)
\(\Rightarrow5.\left(x+46\right)=20.\left(5x+2\right)\)
\(\Rightarrow5x+230=100x+40\)
\(\Rightarrow5x-100x=40-230\)
\(\Rightarrow-95x=-190\)
\(\Rightarrow x=-190:-95\)
\(\Rightarrow x=2\)
\(y\dfrac{5}{y}=\dfrac{86}{y}\)
\(\Rightarrow y+\dfrac{5}{y}=\dfrac{86}{y}\)
\(\Rightarrow\dfrac{y^2+5}{y}=\dfrac{86}{y}\)
\(\Rightarrow y^2+5=86\)
\(\Rightarrow y^2=86-5\)
\(\Rightarrow y^2=81\)
\(\Rightarrow\left[{}\begin{matrix}y=9\\y=-9\end{matrix}\right.\)
Chúc bạn học tốt!
VC
Vũ Cao Minh⁀ᶦᵈᵒᶫ ( ✎﹏IDΣΛ亗 )
CTV
VIP
11 tháng 8 2021
a) \(\frac{-9}{x}=\frac{x}{-49}\Leftrightarrow x^2=\left(-9\right)\left(-49\right)=441\Leftrightarrow x=\pm21\)
b) \(\frac{x}{3}=\frac{y}{5};x+y=-16\). Áp dụng tính chất dãy tỉ số bằng nhau ta có :
\(\frac{x}{3}=\frac{y}{5}=\frac{x+y}{3+5}=\frac{-16}{8}=-2\Rightarrow x=-6;y=-10\)
c) \(\frac{x}{2}=\frac{y}{-5};y-x=-14\). Áp dụng tính chất dãy tỉ số bằng nhau ta có :
\(\frac{x}{2}=\frac{y}{-5}=\frac{y-x}{-5-2}=\frac{-14}{-7}=2\Leftrightarrow x=4;y=-10\)