Tính giá trị của biểu thức:
a. 205 – 36 x 4 + 4
b. (445 x 3 – 246) : 3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
CM
Cao Minh Tâm
13 tháng 5 2018
Đúng(0)
Những câu hỏi liên quan
26 tháng 1 2022
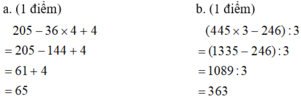
\(\text{a. 78,9 : 2,5 : 4. }\)
\(=31,56:4=7,89.\)
\(\text{ b. 56,75 + 8,4 : 4}.\)
\(=56,75+2,1=58,85.\)
\(\text{c. 23,6 x 100 + 2,9 : 0,1. }\)
\(=2360+29=2389.\)
\(\text{ d. 58 - 4,4 : 0,1}.\)
\(=58-44=14.\)
11 tháng 10 2021
\(a,A=\dfrac{x+\sqrt{x}+2+\sqrt{x}-2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{\sqrt{x}\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}=\dfrac{\sqrt{x}}{\sqrt{x}-2}\\ b,x=36\Leftrightarrow A=\dfrac{6}{6-2}=\dfrac{6}{4}=\dfrac{3}{2}\\ c,A=-\dfrac{1}{3}\Leftrightarrow\dfrac{\sqrt{x}}{\sqrt{x}-2}=-\dfrac{1}{3}\Leftrightarrow3\sqrt{x}=2-\sqrt{x}\\ \Leftrightarrow\sqrt{x}=\dfrac{1}{2}\Leftrightarrow x=\dfrac{1}{4}\left(tm\right)\\ d,A\in Z\Leftrightarrow1+\dfrac{2}{\sqrt{x}-2}\in Z\\ \Leftrightarrow\sqrt{x}-2\inƯ\left(2\right)=\left\{-2;-1;1;2\right\}\\ \Leftrightarrow\sqrt{x}\in\left\{0;1;3;4\right\}\\ \Leftrightarrow x\in\left\{0;1;9;16\right\}\)
\(e,A:B=\dfrac{\sqrt{x}}{\sqrt{x}-2}\cdot\dfrac{\sqrt{x}-2}{\sqrt{x}+1}=\dfrac{\sqrt{x}}{\sqrt{x}+1}=-2\\ \Leftrightarrow\sqrt{x}=-2\sqrt{x}-2\\ \Leftrightarrow\sqrt{x}=-\dfrac{2}{3}\left(ktm\right)\\ \Leftrightarrow x\in\varnothing\)
11 tháng 10 2021
a: Ta có: \(A=\dfrac{x}{x-4}+\dfrac{1}{\sqrt{x}-2}+\dfrac{1}{\sqrt{x}+2}\)
\(=\dfrac{x+\sqrt{x}+2+\sqrt{x}-2}{x-4}\)
\(=\dfrac{\sqrt{x}}{\sqrt{x}-2}\)
S
27 tháng 11 2021
a) 570 - 225 - 167 + 67
= 345 - 167 + 67
= 178 + 67
= 245
168 x 2 : 6 x 4
= 336 : 6 x 4
= 56 x 4
= 224
b) 468 : 6 + 61 x 2
= 78 + 122
= 200
5625 - 5000 : (726 : 6 - 113)
=5625-5000:(121-113)
=5625-5000:8
=5625-625
=5000
Q
2
11 tháng 10 2021
a) \(2x-x^2-4=-\left(x^2-2x+1\right)-3\)
\(=-\left(x-1\right)^2-3\le-3\)
Dấu "=" xảy ra \(\Leftrightarrow x=1\)
b) \(-9x^2+24x-18=-\left(9x^2-24x+16\right)-2\)
\(=-\left(3x-4\right)^2-2\le-2\)
Dấu "=" xảy ra \(\Leftrightarrow x=\dfrac{4}{3}\)
30 tháng 4 2023
a: \(A=\dfrac{x^2+4x+4+4x^2-x^2+4x-4}{\left(x-2\right)\left(x+2\right)}\cdot\dfrac{x-2}{x\left(x^2+x+2\right)}\)
\(=\dfrac{4x^2+8x}{\left(x+2\right)}\cdot\dfrac{1}{x\left(x^2+x+2\right)}=\dfrac{4}{x^2+x+2}\)
|x+3|=5
=>x=2(loại) hoặc x=-8(nhận)
Khi x=-8 thì \(A=\dfrac{4}{64-8+2}=\dfrac{4}{58}=\dfrac{2}{29}\)
b: A nguyên
=>x^2+x+2 thuộc {1;-1;2;-2;4;-4}
=>x^2+x+2=2 hoặc x^2+x+2=4
=>x^2+x-2=0 hoặc x(x+1)=0
=>\(x\in\left\{1;0;-1\right\}\)
HQ
Hà Quang Minh
Giáo viên
30 tháng 11 2023
a) 96 : 3 x 5 = 32 x 5 = 160 | b) 60 : (2 x 3) = 60 : 6 = 10 |
LT
10 tháng 9 2021
a) thay x=4 và y=5 vào biểu thức ta đc :129
b) tương tự....To be continued
10 tháng 9 2021
a:\(A=x^2+2xy-3x^3+2y^3+3x^3-y^3\)
\(=x^2+2xy+y^3\)
\(=5^2+2\cdot5\cdot4+4^3\)
\(=25+40+64=129\)
QT
1
HQ
Hà Quang Minh
Giáo viên
1 tháng 11 2023
a) 4 500 : 90 : 25 = 50 : 25 = 2
b) 840 : (3 x 4) = 840 : 12 = 70
c) 682 + 96 : 12 = 682 + 8 = 690
d) 2 784 : 24 – 16 = 116 – 16 = 100
7 tháng 11 2021
\(A=\left(x-1\right)^2+8\ge8\\ A_{min}=8\Leftrightarrow x=1\\ B=\left(x+3\right)^2-12\ge-12\\ B_{min}=-12\Leftrightarrow x=-3\\ C=x^2-4x+3+9=\left(x-2\right)^2+8\ge8\\ C_{min}=8\Leftrightarrow x=2\\ E=-\left(x+2\right)^2+11\le11\\ E_{max}=11\Leftrightarrow x=-2\\ F=9-4x^2\le9\\ F_{max}=9\Leftrightarrow x=0\)