Tính nguyên hàm của hàm số sau: K = ∫ 2 x 2 + 1 x + 1 5 d x
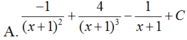
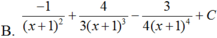

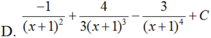
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a/ \(\int\dfrac{x^2-3x+1}{x}dx=\int\left(x-3+\dfrac{1}{x}\right)dx=\int x.dx-3x+\int\dfrac{dx}{x}=\dfrac{1}{2}.x^2-3x+ln\left|x\right|+C\)
b/ \(I=\int x.e^{2x}dx\)
\(\left\{{}\begin{matrix}u=x\\dv=e^{2x}dx\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}du=dx\\v=\dfrac{1}{2}e^{2x}\end{matrix}\right.\)
\(\Rightarrow I=\dfrac{1}{2}.x.e^{2x}-\dfrac{1}{2}\int e^{2x}.dx=\dfrac{1}{2}x.e^{2x}-\dfrac{1}{4}e^{2x}\)

a. \(y'=\dfrac{-1}{\left(x-1\right)}\)
b. \(y'=\dfrac{5}{\left(1-3x\right)^2}\)
c. \(y=\dfrac{\left(x+1\right)^2+1}{x+1}=x+1+\dfrac{1}{x+1}\Rightarrow y'=1-\dfrac{1}{\left(x+1\right)^2}=\dfrac{x^2+2x}{\left(x+1\right)^2}\)
d. \(y'=\dfrac{4x\left(x^2-2x-3\right)-2x^2\left(2x-2\right)}{\left(x^2-2x-3\right)^2}=\dfrac{-4x^2-12x}{\left(x^2-2x-3\right)^2}\)
e. \(y'=1+\dfrac{2}{\left(x-1\right)^2}=\dfrac{x^2-2x+3}{\left(x-1\right)^2}\)
g. \(y'=\dfrac{\left(4x-4\right)\left(2x+1\right)-2\left(2x^2-4x+5\right)}{\left(2x+1\right)^2}=\dfrac{4x^2+4x-14}{\left(2x+1\right)^2}\)
2.
a. \(y'=4\left(x^2+x+1\right)^3.\left(x^2+x+1\right)'=4\left(x^2+x+1\right)^3\left(2x+1\right)\)
b. \(y'=5\left(1-2x^2\right)^4.\left(1-2x^2\right)'=-20x\left(1-2x^2\right)^4\)
c. \(y'=3\left(\dfrac{2x+1}{x-1}\right)^2.\left(\dfrac{2x+1}{x-1}\right)'=3\left(\dfrac{2x+1}{x-1}\right)^2.\left(\dfrac{-3}{\left(x-1\right)^2}\right)=\dfrac{-9\left(2x+1\right)^2}{\left(x-1\right)^4}\)
d. \(y'=\dfrac{2\left(x+1\right)\left(x-1\right)^3-3\left(x-1\right)^2\left(x+1\right)^2}{\left(x-1\right)^6}=\dfrac{-x^2-6x-5}{\left(x-1\right)^4}\)
e. \(y'=-\dfrac{\left[\left(x^2-2x+5\right)^2\right]'}{\left(x^2-2x+5\right)^4}=-\dfrac{2\left(x^2-2x+5\right)\left(2x-2\right)}{\left(x^2-2x+5\right)^4}=-\dfrac{4\left(x-1\right)}{\left(x^2-2x+5\right)^3}\)
f. \(y'=4\left(3-2x^2\right)^3.\left(3-2x^2\right)'=-16x\left(3-2x^2\right)^3\)

Chọn đáp án C
Phương pháp
Sử dụng công thức nguyên hàm:
![]()
dựa dữ kiện đề bài tìm được C, từ đó tính F(2)-F(-1)


Ý bạn là \(f(x)=\frac{1}{9+x^2}+\frac{3}{9+x^2}\) hay thế nào? Bạn cần viết đề bằng công thức toán (biểu tượng $\sum$ góc trái khung soạn thảo để được hỗ trợ tốt hơn).

\(f\left(x\right)=\dfrac{x^2-1}{x^2}=1-\dfrac{1}{x^2}\)
\(\int f\left(x\right)dx=\int\left(1-\dfrac{1}{x^2}\right)dx=\int1dx-\int x^{-2}dx\)
=\(x-\dfrac{x^{-2+1}}{-2+1}+C=x-\dfrac{x^{-1}}{-1}+C=x+\dfrac{1}{x}+C\)
C=-1 ta được phương án A(ko tm câu hỏi)
C=0 ta được phương án B(ko tm câu hỏi)
C=2 ta được phương án C(ko tm câu hỏi)
=>chọn D

Chọn B.
Ta phân tích 2 x 2 + 1 = 2 x + 1 2 - 4 x + 1 + 3
Suy ra: