Giải các phương trình:
2 x + 3 x - 3 - 4 x + 3 = 24 x 2 - 9 + 2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
CM
Cao Minh Tâm
15 tháng 10 2017
Đúng(0)
Những câu hỏi liên quan
PG
3
6 tháng 4 2018
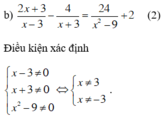
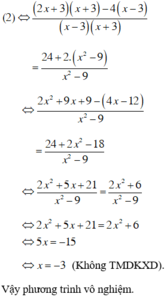
1, \(_{\left|x^2-5x-6\right|=x^2+x-24}\) (1)
Điều kiện \(x^2+x-24\ge0\) <=> \(\orbr{\begin{cases}x\ge\frac{-1+\sqrt{97}}{2}\\x\le\frac{-1-\sqrt{97}}{2}\end{cases}}\)
Khi đó (1) <=> \(\orbr{\begin{cases}x^2-5x-6=x^2+x-24\\x^2-5x-6=-x^2-x+24\end{cases}}\)
<=> \(\orbr{\begin{cases}-6x=-18\\2x^2-4x-30=0\end{cases}}\)
<=> \(\orbr{\begin{cases}x=3\\x^2-2x-15=0\end{cases}}\)
<=> \(x\in\left\{-3;3;5\right\}\)
Loại 2 giá trị x = -3 và x = 3 do ko t/m đk bên trên, ta đc x = 5 là nghiệm duy nhất của pt
Vậy tập nghiệm của pt là S = {5}
6 tháng 4 2018
|x^2-5x-6|=x^2+x-24
=>x= 5
|x-1|-2|x-2|+3|x-3|=4
=> x= 5 hoac bang 1
TM
11 tháng 8 2021
1/ \(7x-5=13-5x\)
\(\Leftrightarrow12x=18\)
\(\Leftrightarrow x=\dfrac{3}{2}\)
Vậy: \(S=\left\{\dfrac{3}{2}\right\}\)
==========
2/ \(19+3x=5-18x\)
\(\Leftrightarrow21x=-14\)
\(\Leftrightarrow x=-\dfrac{2}{3}\)
Vậy: \(S=\left\{-\dfrac{2}{3}\right\}\)
==========
3/ \(x^2+2x-4=-12+3x+x^2\)
\(\Leftrightarrow-x=-8\)
\(\Leftrightarrow x=8\)
Vậy: \(S=\left\{8\right\}\)
===========
4/ \(-\left(x+5\right)=3\left(x-5\right)\)
\(\Leftrightarrow-x-5=3x-15\)
\(\Leftrightarrow-4x=-10\)
\(\Leftrightarrow x=\dfrac{5}{2}\)
Vậy: \(S=\left\{\dfrac{5}{2}\right\}\)
==========
5/ \(3\left(x+4\right)=\left(-x+4\right)\)
\(\Leftrightarrow3x+12=-x+4\)
\(\Leftrightarrow4x=-8\)
\(\Leftrightarrow x=-2\)
Vậy: \(S=\left\{-2\right\}\)
[----------]
NT
11 tháng 8 2021
1. \(7x-5=13-5x\) \(\Leftrightarrow12x=18\Leftrightarrow x=\dfrac{3}{2}\)
2. \(19+3x=5-18x\Leftrightarrow21x=-14\Leftrightarrow x=-\dfrac{2}{3}\)
3. \(x^2+2x-4=-12+3x+x^2\Leftrightarrow-x=-8\Leftrightarrow x=8\)
4. \(-\left(x+5\right)=3\left(x-5\right)\Leftrightarrow-x-5=3x-15\Leftrightarrow4x=10\Leftrightarrow x=\dfrac{5}{2}\)
5. \(3\left(x+4\right)=-x+4\Leftrightarrow3x+12=-x+4\Leftrightarrow4x=-8\Leftrightarrow x=-2\)
9 tháng 3 2018
1 ) đặt ẩn phụ
căn(x+4) = a
căn(4-x) = b
=> a^2 + b^2 = 8 ; a^2 - b^2 = 2x
Thay vào phương trình giải rất dễ
2) điều kiện xác định " x lớn hơn hoặc = 1
từ ĐKXĐ => vế trái lớn hơn hoặc = 1
=> 2 - x lớn hơn hoặc = 1
=> x nhỏ hơn hoặc = 1
kết hợp ĐKXĐ => x = 1
3) mk chưa biết làm
TN
30 tháng 1 2016
1)\(\Leftrightarrow2x^2+3x-14=0\)
\(\Rightarrow3^2-\left(-4\left(2.14\right)\right)=121\)
\(\Rightarrow x_{1,2}=\frac{-b+-\sqrt{D}}{2a}=\frac{-3+-\sqrt{121}}{4}\)
=>\(x=2hoặc-\frac{7}{2}\)
tối nay tôi làm tiếp cho
CC
2
13 tháng 7 2017
1. Ta có \(\left(x+2\right)\left(x+4\right)\left(x+6\right)\left(x+8\right)+16=0\)
\(\Rightarrow\)\(\left[\left(x+2\right)\left(x+8\right)\right].\left[\left(x+4\right)\left(x+6\right)\right]+16=0\)
\(\Rightarrow\left(x^2+10x+16\right)\left(x^2+10x+24\right)+16=0\)
Đặt \(x^2+10x=t\)
Pt \(\Leftrightarrow\left(t+16\right)\left(t+24\right)+16=0\Leftrightarrow t^2+40t+400=0\Leftrightarrow t=-20\)
\(\Rightarrow x^2+10x+20=0\Rightarrow\orbr{\begin{cases}x=-5+\sqrt{5}\\x=-5-\sqrt{5}\end{cases}}\)
2. Ta có \(\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(x+5\right)-24=0\)
\(\Rightarrow\left[\left(x+2\right)\left(x+5\right)\right].\left[\left(x+3\right)\left(x+4\right)\right]-24=0\)\(\Rightarrow\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24=0\)
Đặt \(x^2+7x=t\Rightarrow\left(t+10\right)\left(t+12\right)-24=0\Rightarrow t^2+22t+96=0\)\(\Rightarrow\orbr{\begin{cases}t=-6\\t=-16\end{cases}}\)
Với \(t=-6\Rightarrow x^2+7x+6=0\Rightarrow\orbr{\begin{cases}x=-6\\x=-1\end{cases}}\)
Với \(t=-16\Rightarrow x^2+7x+16=0\left(l\right)\)
Vậy pt có 2 nghiệm là \(\orbr{\begin{cases}x=-6\\x=-1\end{cases}}\)
GL
18 tháng 7 2017
Quản lí Hoàng Thị Lan Hương giúp em giải bài toán vừa đăng lên đc ko ạ.??? ^^
PB
2
18 tháng 6 2020
\(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)=24\)
<=> \(\left[\left(x+1\right)\left(x+4\right)\right]\left[\left(x+2\right)\left(x+3\right)\right]=2\)
<=> \(\left(x^2+5x+4\right)\left(x^2+5x+6\right)=24\)
Đặt: \(x^2+5x+4=t\) ta có phương trình:
\(t\left(t+2\right)=24\)
<=> \(t^2+2t-24=0\)
<=> t = 4 hoặc t = -6
Với t = 4 ta có: \(x^2+5x+4=4\)<=> x = 0 hoặc x = - 5
Với t = - 6 ta có: \(x^2+5x+4=-6\) phương trình vô nghiệm
Vậy x = 0 hoặc x = -5
DB
27 tháng 6 2020
[(x+1).(x+4].[(x+2).(x+3)] =24
<-> (x2+4X+X+4).(x2+3x+2x+6)=24
<-> (x2+5x+4).(x2+5x+6)=24
đặt x2+5x+4=a
<-> a.(a+2)=24
<-> a2+2a-24+0
ta có \(\Delta\)= 22-4.1.(-24)
=4+96
=100 >0
-> \(\sqrt{\Delta}\)=\(\sqrt{100}\)=10
=> pt có 2 nghiệm pb
x1= \(\frac{2+10}{2}\)=6
x2=\(\frac{2-10}{2}\)=-4
AH
Akai Haruma
Giáo viên
30 tháng 8 2021
1.
$2(-2x+1)\leq -x+3$
$\Leftrightarrow -4x+2\leq -x+3$
$\Leftrightarrow -1\leq 3x$
$\Leftrightarrow x\geq \frac{-1}{3}$
2.
$2(x+1)\leq -x+3$
$\Leftrightarrow 2x+2\leq -x+3$
$\Leftrightarrow 3x\leq 1$
$\Leftrightarrow x\leq \frac{1}{3}$
AH
Akai Haruma
Giáo viên
30 tháng 8 2021
3.
$5-3(x-1)>2$
$\Leftrightarrow 5-(3x-3)>2$
$\Leftrightarrow 8-3x>2$
$\Leftrightarrow 8-3x-2>0$
$\Leftrightarrow 6-3x>0$
$\Leftrightarrow 6>3x$
$\Leftrightarrow x< 2$
4.
$x^2-12x+3-(x-3)^2>0$
$\Leftrightarrow x^2-12x+3-(x^2-6x+9)>0$
$\Leftrightarrow -6x-6>0$
$\Leftrightarrow -6>6x$
$\Leftrightarrow x< -1$
5 tháng 8 2015
Xét tích (x+1)(x+2)(x+3)(x+4) là tích của 4 số tự nhiên liên tiếp.
Mà ta thấy 24 = 1 . 2 . 3 . 4
Vậy x + 1 = 1 ; x + 2 = 2 ; x + 3 = 3 ; x + 4 = 4
Do đó x = 0
MT
5 tháng 8 2015
(x+1)(x+2)(x+3)(x+4)= 24
<=> (x+1)(x+2)(x+3)(x+4)-24=0
<=>(x+1)(x+4)(x+2)(x+3)-24=0
<=>(x2+5x+4)(x2+5x+6)-24=0
Đặt t=x2+5x+4 ta được:
t.(t+2)-24=0
<=>t2+2t-24=0
<=>t2-4t+6t-24=0
<=>t.(t-4)+6.(t-4)=0
<=>(t-4)(t+6)=0
<=>t-4=0 hoặc t+6=0
thay t=x2+5x+4 ta được:
x2+5x=0 hoặc x2+5x+10=0
Vì x2+5x+10=x2+2.x.5/2+25/4+15/4
=(x+5/2)2+15/4>0
nên
x2+5x=0
<=>x.(x+5)=0
<=>x=0 hoặc x=-5