Cho x thỏa mãn điều kiện log 140 63 = x . log x 3 . log 7 x 1 log x 3...

PT

Pham Trong Bach

21 tháng 8 2019

Cho x thỏa mãn điều kiện log 140 63 = x . log x 3 . log 7 x + 1 log x 3 . log 3 5 . log 7 x + 1 . Tìm giá trị của x: A. x = 2 B. x = 4 C. x = 3 D. x =...

Đọc tiếp

#Mẫu giáo

1

CM

Cao Minh Tâm

21 tháng 8 2019

Sử dụng chức năng CALC trong máy tính Casio và nhập từ giá trị ta thấy x = 1 thỏa.

Đúng(0)

PT

Pham Trong Bach

27 tháng 8 2018

Cho x, y > 0 thỏa mãn log(x + 2y) = log x + log y. Khi đó, giá trị nhỏ nhất của biểu thức P = x 2 1 + 2 y + 4 y 2 1 + x là: A. 6 B. 32 5 C. 31 5 D. 29 5 ...

Đọc tiếp

#Toán lớp 12

1

CM

Cao Minh Tâm

27 tháng 8 2018

Đáp án B

Ta có log(x + 2y) = log x + log y

<=> log 2 (x+2y) = log 2xy

<=> 2 (x+2y) = 2xy (*).

Đ ặ t a = x > 0 b = 2 y > 0 , khi đó

* ⇔ 2 a + b = a b

và P = a 2 1 + b + b 2 1 + a ≥ a + b 2 a + b + 2

Lại có a b ≤ a + b 2 4 ⇒ 2 a + b ≤ a + b 2 4 ⇔ a + b ≥ 8 .

Đặt t = a + b, do đó

P ≥ f t = t 2 t + 2 .

X é t h à m s ố f t = t 2 t + 2 t r ê n [ 8 ; + ∞ )

c ó f ' t = t 2 + 2 t t + 2 2 > 0 ; ∀ ≥ 8

Suy ra f(t) là hàm số đồng biến trên [ 8 ; + ∞ )

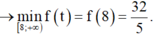
Vậy gía trị nhỏ nhất của biểu thức P là 32 5 .

Đúng(0)

PT

Pham Trong Bach

2 tháng 1 2019

Cho các số thực dương a,b thỏa mãn log a = x , log b = y . Tính P = log ( a 2 b 3 ) ...

Đọc tiếp

#Toán lớp 12

1

CM

Cao Minh Tâm

2 tháng 1 2019

Đúng(0)

PT

Pham Trong Bach

3 tháng 9 2018

Cho x, y > 0 thỏa mãn log x + 2 y = log x + log y . Khi đó, giá trị nhỏ nhất của biểu thức P = x 2 1 + 2 y + 4 y 2 1 + x

A. 6

B. 31 5

C. 32 5

D. 39 5

#Mẫu giáo

1

CM

Cao Minh Tâm

3 tháng 9 2018

Đáp án C

Đúng(0)

PT

Pham Trong Bach

28 tháng 1 2019

Cho các số thực x, y, z thỏa mãn y = 10 1 1 - log x , z = 10 1 1 - log y . Mệnh đề nào sau đây đúng? ...

Đọc tiếp

#Toán lớp 12

1

CM

Cao Minh Tâm

28 tháng 1 2019

Đáp án D

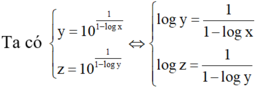
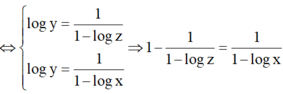
⇔ log z - 1 log z = 1 1 - log x

⇔ 1 - log x = log z log z - 1

⇔ log x = - 1 log z - 1 ⇔ x = 10 1 1 - log z .

Đúng(0)

PT

Pham Trong Bach

15 tháng 11 2019

Cho hai số thực dương x, y thỏa mãn log x + log y ≥ log ( x 3 + 2 y ) Giá trị nhỏ nhất của P = 25x + y là A. 375/4 B. 45/2 C. 195/2 D. 14 26 ...

Đọc tiếp

#Mẫu giáo

1

CM

Cao Minh Tâm

15 tháng 11 2019

Đúng(0)

PT

Pham Trong Bach

12 tháng 3 2019

Có bao nhiêu giá trị nguyên dương của x thỏa mãn bất phương trình dưới đây:

log (x - 40) + log (60 - x) < 2?

A. 20

B. 10

C. Vô số

D. 18

#Toán lớp 12

1

CM

Cao Minh Tâm

12 tháng 3 2019

Đáp án D

Điều kiện 40 < x < 60

Vậy x cần tìm theo yêu cầu đề là các số nguyên dương chạy từ 41 đến 59; trừ giá trị 50. Có tất cả 18 giá trị thỏa mãn.

Đúng(0)

B

Buddy

13 tháng 8 2023

Giải mỗi phương trình sau:

a) \({\log _5}\left( {2x - 4} \right) + {\log _{\frac{1}{5}}}\left( {x - 1} \right) = 0\)

b) \({\log _2}x + {\log _4}x = 3\)

#Toán lớp 11

1

D

datcoder

CTVVIP

14 tháng 8 2023

a)

ĐK: \(\left\{{}\begin{matrix}2x-4>0\\x-1>0\end{matrix}\right.\Leftrightarrow x>1\)

\(\log_5\left(2x-4\right)+\log_{\dfrac{1}{5}}\left(x-1\right)=0\\ \Leftrightarrow\log_5\left(2x-4\right)-\log_5\left(x-1\right)=0\\ \Leftrightarrow\log_5\left(\dfrac{2x-4}{x-1}\right)=\log_51\\ \Leftrightarrow\dfrac{2x-4}{x-1}=1\\ \Leftrightarrow2x-4=x-1\\ \Leftrightarrow x=3\left(tm\right)\)

Vậy x = 3.

b) ĐK: x > 0

\(\log_2x+\log_4x=3\\ \Leftrightarrow\log_2x+\dfrac{1}{2}\log_2x=3\\ \Leftrightarrow\left(1+\dfrac{1}{2}\right)\log_2x=3\\ \Leftrightarrow\dfrac{3}{2}\log_2x=3\\ \Leftrightarrow\log_2x=2\\ \Leftrightarrow x=4\left(tm\right)\)

Vậy x= 4

Đúng(1)

PT

Pham Trong Bach

16 tháng 8 2018

Tìm tập nghiệm của phương trình l o g ( x + 3 ) + l o g ( x - 1 ) = l o g ( x 2 - 2 x - 3 )

A. ∅

B. {0}

C. R

D. (1; +∞)

#Toán lớp 12

1

CM

Cao Minh Tâm

16 tháng 8 2018

Đúng(0)

B

Buddy

15 tháng 8 2023

Cho bốn số thực dương a, b, x, y với \(a,b \ne 1\). Khẳng định nào sau đây là sai?A. \({\log _a}(xy) = {\log _a}x + {\log _b}y\). B. \({\log _a}\frac{x}{y} = {\log _a}x - {\log _a}y\).C. \({\log _a}\frac{1}{x} = \frac{1}{{{{\log }_a}x}}\). D. \({\log _a}b \cdot {\log _b}x = {\log...

Đọc tiếp

#Toán lớp 11

1

HQ

Hà Quang Minh

Giáo viên

22 tháng 9 2023

Đáp án C.

Đúng(0)

B

Buddy

15 tháng 8 2023

Giải các bất phương trình sau:

a) \({\log _{\frac{1}{7}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right);\)

b) \(2\log \left( {2x + 1} \right) > 3.\)

#Toán lớp 11

1

HQ

Hà Quang Minh

Giáo viên

22 tháng 9 2023

a) \({\log _{\frac{1}{7}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\) (ĐK: \(x + 1 > 0;2 - x > 0 \Leftrightarrow - 1 < x < 2\))

\(\begin{array}{l} \Leftrightarrow {\log _{{7^{ - 1}}}}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow - {\log _7}\left( {x + 1} \right) > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow {\log _7}{\left( {x + 1} \right)^{ - 1}} > {\log _7}\left( {2 - x} \right)\\ \Leftrightarrow {\left( {x + 1} \right)^{ - 1}} > 2 - x\\ \Leftrightarrow \frac{1}{{x + 1}} - 2 + x > 0\\ \Leftrightarrow \frac{{1 + \left( {x - 2} \right)\left( {x + 1} \right)}}{{x + 1}} > 0\\ \Leftrightarrow \frac{{1 + {x^2} - x - 2}}{{x + 1}} > 0 \Leftrightarrow \frac{{{x^2} - x - 1}}{{x + 1}} > 0\end{array}\)

Mà – 1 < x < 2 nên x + 1 > 0

\( \Leftrightarrow {x^2} - x - 1 > 0 \Leftrightarrow \left[ \begin{array}{l}x < \frac{{1 - \sqrt 5 }}{2}\\x > \frac{{1 + \sqrt 5 }}{2}\end{array} \right.\)

KHĐK ta có \(\left[ \begin{array}{l} - 1 < x < \frac{{1 - \sqrt 5 }}{2}\\\frac{{1 + \sqrt 5 }}{2} < x < 2\end{array} \right.\)

b) \(2\log \left( {2x + 1} \right) > 3\) (ĐK: \(2x + 1 > 0 \Leftrightarrow x > \frac{{ - 1}}{2}\))

\(\begin{array}{l} \Leftrightarrow \log \left( {2x + 1} \right) > \frac{3}{2}\\ \Leftrightarrow 2x + 1 > {10^{\frac{3}{2}}} = 10\sqrt {10} \\ \Leftrightarrow x > \frac{{10\sqrt {10} - 1}}{2}\end{array}\)

KHĐK ta có \(x > \frac{{10\sqrt {10} - 1}}{2}\)

Đúng(0)

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP