tìm x, biết: x3+x=0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: \(\Leftrightarrow x\left(x-5\right)\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=5\\x=-5\end{matrix}\right.\)

Ta có: x 3 + x = 0 ⇒ x( x 2 + 1) = 0
Vì x 2 ≥ 0 nên x 2 + 1 ≥ 1 > 0 với mọi x
Vậy x = 0

x 3 – x 2 – x + 1 = 0 ⇔ ( x 3 – x 2 ) – ( x – 1 ) = 0 ⇔ x 2 ( x – 1 ) – ( x – 1 ) = 0 ⇔ ( x 2 – 1 ) ( x – 1 ) = 0 ⇔ ( x – 1 ) ( x + 1 ) ( x – 1 ) = 0 ⇔ ( x – 1 ) 2 ( x + 1 ) = 0
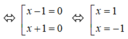
Vậy x = 1 hoặc x = -1
Đáp án cần chọn là: A

x 3 – x 2 – x + 1 = 0 ⇔ ( x 3 – x 2 ) – ( x – 1 ) = 0 ⇔ x 2 ( x – 1 ) – ( x – 1 ) = 0 ⇔ ( x 2 – 1 ) ( x – 1 ) = 0 ⇔ ( x – 1 ) ( x + 1 ) ( x – 1 ) = 0 ⇔ ( x – 1 ) 2 ( x + 1 ) = 0
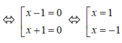
Vậy x = 1 hoặc x = -1
Đáp án cần chọn là: A

a) x = -1. b) x = 4 hoặc x = 5.
c) x = ± 2 . d) x = 1 hoặc x = 2.

\(a,\Leftrightarrow\left(x+3\right)\left(x+3-2x-1\right)=0\\ \Leftrightarrow\left(x+3\right)\left(2-x\right)=0\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=2\end{matrix}\right.\\ b,\Leftrightarrow x\left(x^2-12x+36\right)=0\\ \Leftrightarrow x\left(x-6\right)^2=0\Leftrightarrow\left[{}\begin{matrix}x=0\\x=6\end{matrix}\right.\)
a, (x+3)2 - ( 2x + 1 ).( x+3)=0 b, x3-12x2+36x =0
=> (x+3).(x+3-2x-1) => x(x2-12x+36) = 0
=>(x+3).(-x+2) => x(x-6)2 = 0
=> x+3=0 <=> x=-3 => x=0 <=> x=0
-x+2=0 <=> x=-2 x-6= 0 <=> x=6

x3 - 3x = 0
x.x2 - 3x = 0
x.(x2 - 3) = 0
=> x = 0 hoặc x2 - 3 = 0
=> x = 0 hoặc x2 = 3
=> x = 0 hoặc x = √3
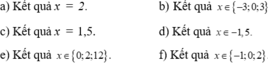
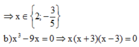
Ta có : x3 + x = 0
=> x(x2 + 1) = 0
=> x = 0 (Vì x2 + 1 \(\ge1>0\forall x\))
Vậy x = 0
Ta có : x3 + x = 0
<=> x( x2 + 1 ) = 0
<=> x = 0 hoặc x2 + 1 = 0
Vì x2 + 1 ≥ 1 > 0 ∀ x
<=> x = 0
Vậy x = 0