Cho biểu thức P=2a2 +9a-6
Tìm giá trị nhỏ nhất của biểu thức P
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NT
1
23 tháng 9 2021
C=2a2+b2-2ab+10a+42
=a2-2ab+b2+a2+10a+25+17
=(a-b)2+(a+5)2+17
=>MIN(C)=17 <=>a-b=0 và a+5=0
<=>a=b=-5
vậy ..................
21 tháng 8 2021
- p lon nhat khi x = 7 , p nho nhat khi x = 6
- p lon nhat = 2554 , p nho nhat = 2014
dung khong ta ?
27 tháng 2 2021
a) Thay x=4 vào biểu thức \(B=\dfrac{3}{\sqrt{x}-1}\), ta được:
\(B=\dfrac{3}{\sqrt{4}-1}=\dfrac{3}{2-1}=3\)
Vậy: Khi x=4 thì B=3
b) Ta có: P=A-B
\(\Leftrightarrow P=\dfrac{6}{x-1}+\dfrac{\sqrt{x}}{\sqrt{x}+1}-\dfrac{3}{\sqrt{x}-1}\)
\(\Leftrightarrow P=\dfrac{6}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}+\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}-\dfrac{3\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Leftrightarrow P=\dfrac{6+x-\sqrt{x}-3\sqrt{x}-3}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Leftrightarrow P=\dfrac{x-\sqrt{x}-3\sqrt{x}+3}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Leftrightarrow P=\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)-3\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Leftrightarrow P=\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Leftrightarrow P=\dfrac{\sqrt{x}-3}{\sqrt{x}+1}\)
CM
25 tháng 3 2019
Giả sử phương trình đã cho có 3 nghiệm
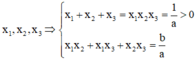
Khi đó
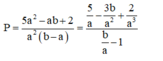
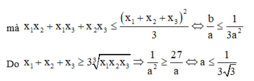
Suy ra
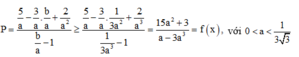
Xét hàm số:
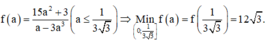
Chọn D.
22 tháng 1 2024
a: Để \(\dfrac{3x-2}{4}\) không nhỏ hơn \(\dfrac{3x+3}{6}\) thì \(\dfrac{3x-2}{4}>=\dfrac{3x+3}{6}\)
=>\(\dfrac{6\left(3x-2\right)}{24}>=\dfrac{4\left(3x+3\right)}{24}\)
=>18x-12>=12x+12
=>6x>=24
=>x>=4
b: Để \(\left(x+1\right)^2\) nhỏ hơn \(\left(x-1\right)^2\) thì \(\left(x+1\right)^2< \left(x-1\right)^2\)
=>\(x^2+2x+1< x^2-2x+1\)
=>4x<0
=>x<0
c: Để \(\dfrac{2x-3}{35}+\dfrac{x\left(x-2\right)}{7}\) không lớn hơn \(\dfrac{x^2}{7}-\dfrac{2x-3}{5}\) thì
\(\dfrac{2x-3}{35}+\dfrac{x\left(x-2\right)}{7}< =\dfrac{x^2}{7}-\dfrac{2x-3}{5}\)
=>\(\dfrac{2x-3+5x\left(x-2\right)}{35}< =\dfrac{5x^2-7\cdot\left(2x-3\right)}{35}\)
=>\(2x-3+5x^2-10x< =5x^2-14x+21\)
=>-8x-3<=-14x+21
=>6x<=24
=>x<=4
P = 2a2 + 9a - 6
= 2( a2 + 9/2a + 81/16 ) - 129/8
= 2( a + 9/4 )2 - 129/8 ≥ -129/8 ∀ a
Dấu "=" xảy ra khi a = -9/4
=> MinP = -129/8 <=> a = -9/4