Tìm Min, Max: y= cosx + cos(x+\(\frac{\pi}{3}\))
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
8 tháng 9 2020
a/ \(y=2\left(\frac{\sqrt{3}}{2}sinx-\frac{1}{2}cosx\right)+5=2sin\left(x-\frac{\pi}{6}\right)+5\)
Do \(-1\le sin\left(x-\frac{\pi}{6}\right)\le1\Rightarrow3\le y\le7\)
b/ \(y=2cos\left(x+\frac{\pi}{6}\right)cos\left(-\frac{\pi}{6}\right)=\sqrt{3}cos\left(x+\frac{\pi}{6}\right)\)
Do \(-1\le cos\left(x+\frac{\pi}{6}\right)\le1\Rightarrow-\sqrt{3}\le y\le\sqrt{3}\)
c/ \(y=2\left(\frac{1}{2}sinx+\frac{\sqrt{3}}{2}cosx\right)+12=2sin\left(x+\frac{\pi}{3}\right)+12\)
Do \(-1\le sin\left(x+\frac{\pi}{3}\right)\le1\Rightarrow10\le y\le14\)
NL
1
TA
22 tháng 5 2021
ĐK: Biểu thức xác định với mọi `x`.
`y_(min) <=> (\sqrt(2-cos(x-π/6))+3)_(max) <=> (cos(x-π/6))_(max)`
`<=> cos(x-π/6)=1 <=> x-π/6=k2π <=> x = π/6+k2π ( k \in ZZ)`.
`=> y_(min) = 1`
`y_(max) <=> (\sqrt(2-cos(x-π/6))+3)_(min) <=> (cos(x-π/6))_(min)`
`<=> cos(x-π/6)=-1 <=> x -π/6= π+k2π <=> x = (7π)/6+k2π (k \in ZZ)`
`=> y_(max) = (6-2\sqrt3)/3`.
12 tháng 9 2021
1, \(y=2-sin\left(\dfrac{3x}{2}+x\right).cos\left(x+\dfrac{\pi}{2}\right)\)
\(y=2-\left(-cosx\right).\left(-sinx\right)\)
y = 2 - sinx.cosx
y = \(2-\dfrac{1}{2}sin2x\)
Max = 2 + \(\dfrac{1}{2}\) = 2,5
Min = \(2-\dfrac{1}{2}\) = 1,5
2, y = \(\sqrt{5-\dfrac{1}{2}sin^22x}\)
Min = \(\sqrt{5-\dfrac{1}{2}}=\dfrac{3\sqrt{2}}{2}\)
Max = \(\sqrt{5}\)
NV
Nguyễn Việt Lâm
Giáo viên
16 tháng 9 2020
\(y=sinx.cosx\left(sin^2x-cos^2x\right)=\frac{1}{2}sin2x.\left(-cos2x\right)=-\frac{1}{4}sin4x\)
Do \(-1\le sin4x\le1\Rightarrow-\frac{1}{4}\le y\le\frac{1}{4}\)
\(y_{min}=-\frac{1}{4}\) khi \(sin4x=1\)
\(y_{max}=\frac{1}{4}\) khi \(sin4x=-1\)
QT
Quoc Tran Anh Le
Giáo viên
21 tháng 9 2023
a) Với mọi \(x \in \mathbb{R}\) ta có \( - 1 \le cosx \le 1\)
Vậy phương trình \(cosx = - 3\;\) vô nghiệm.
\(\begin{array}{l}b)\,\;cosx = cos{15^o}\;\\ \Leftrightarrow \left[ \begin{array}{l}x = {15^o} + k{360^o},k \in \mathbb{Z}\\x = - {15^o} + k{360^o},k \in \mathbb{Z}\end{array} \right.\end{array}\)
Vậy phương trình có nghiệm \(x = {15^o} + k{360^o}\) hoặc \(x = - {15^o} + k{360^o},k \in \mathbb{Z}\).
\(\begin{array}{l}c)\;\,cos(x + \frac{\pi }{{12}}) = cos\frac{{3\pi }}{{12}}\\ \Leftrightarrow \left[ \begin{array}{l}x + \frac{\pi }{{12}} = \frac{{3\pi }}{{12}} + k2\pi ,k \in \mathbb{Z}\\x + \frac{\pi }{{12}} = - \frac{{3\pi }}{{12}} + k2\pi ,k \in \mathbb{Z}\end{array} \right.\\ \Leftrightarrow \left[ \begin{array}{l}x = \frac{\pi }{6} + k2\pi ,k \in \mathbb{Z}\\x = - \frac{\pi }{3} + k2\pi ,k \in \mathbb{Z}\end{array} \right.\end{array}\)
Vậy phương trình có nghiệm \(x = \frac{\pi }{6} + k2\pi ,\) hoặc \(x = - \frac{\pi }{3} + k2\pi ,k \in \mathbb{Z}\).
JP
1
NV
Nguyễn Việt Lâm
Giáo viên
14 tháng 8 2020
\(0\le cos^2\left(x-\frac{\pi}{4}\right)\le1\Rightarrow1\le y\le2\)
\(y_{min}=1\) khi \(cos\left(x-\frac{\pi}{4}\right)=0\)
\(y_{max}=2\) khi \(cos^2\left(x-\frac{\pi}{4}\right)=1\)
TA
6 tháng 8 2021
1. Hàm số xác định `<=> 1-cosx \ne 0<=>cosx \ne 1<=>x \ne k2π`
Vì: `1+cosx >=0 forallx ; 1-cosx >=0 forall x`
2. Hàm số xác định `<=> sin^2x \ne cos^2x <=> (1-cos2x)/2 \ne (1+cos2x)/2`
`<=>cos2x \ne 0<=> 2x \ne π/2+kπ <=> x \ne π/4+kπ/2`
3. Hàm số xác định `<=> cos2x \ne 0<=> x \ne π/4+kπ/2 (k \in ZZ)`.
LA
14 tháng 8 2021
Bạn cho mình hỏi tại sao x khác k2\(\pi\) là lý thuyết ở đoạn nào thế ạ?
HQ
Hà Quang Minh
Giáo viên
21 tháng 9 2023
a) Vẽ đồ thị:
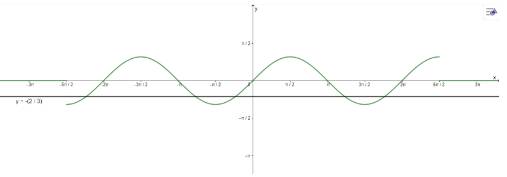
\(3\sin x + 2 = 0\) trên đoạn \(\left( { - \frac{{5\pi }}{2};\frac{{5\pi }}{2}} \right)\) có 5 nghiệm
b) Vẽ đồ thị:
\(\cos x = 0\) trên đoạn \(\left[ { - \frac{{5\pi }}{2};\frac{{5\pi }}{2}} \right]\) có 6 nghiệm
\(y=2cos\left(x+\frac{\pi}{6}\right)cos\left(\frac{\pi}{6}\right)=\sqrt{3}cos\left(x+\frac{\pi}{6}\right)\)
Do \(-1\le cos\left(x+\frac{\pi}{6}\right)\le1\) nên \(-\sqrt{3}\le y\le\sqrt{3}\)
\(y_{min}=-\sqrt{3}\) khi \(cos\left(x+\frac{\pi}{6}\right)=-1\)
\(y_{max}=\sqrt{3}\) khi \(cos\left(x+\frac{\pi}{6}\right)=1\)