Cho phương trình \(\left(m+1\right)x^2-2\left(m+1\right)x+m-3=0\) Tìm m để phương tình có hai nghiệm thỏa mãn \(4\left(x_1+1\right)\left(4x_2+1\right)=18\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a, Thay m vào pt ta được :
(3+1).x2-2(3+1).x+3-3=0
\(\Leftrightarrow\)4x2-8x=0
\(\Leftrightarrow4x\left(x-2\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\\\x-2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\end{matrix}\right.\)
Vậy m=3 phương trình có 2 nghiệm là 0 và 2
b, Theo Vi et ta có :
\(\left\{{}\begin{matrix}x_1.x_2=\dfrac{m-3}{m+1}\\x_1+x_2=\dfrac{2\left(m+1\right)}{m+1}\end{matrix}\right.\left(vớim\ne-1\right)\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1.x_2=\dfrac{m-3}{m+1}\\x_1+x_2=2\end{matrix}\right.\) (1)
Ta có : (4x1+1)(4x2+1)=18
\(\Leftrightarrow16x_1.x_2+4x_1+4x_2+1=18\)
\(\Leftrightarrow16.x_1.x_2+4\left(x_1+x_2\right)=17\) (2)
Thay (1) vào (2) ta được :
16.\(\dfrac{m-3}{m+1}+4.2=17\)
\(\Leftrightarrow\dfrac{16m-48}{m+1}=9\)
\(\Leftrightarrow9\left(m+1\right)=16m-48\)
\(\Leftrightarrow9m+9=16m-48\)
\(\Leftrightarrow7m=57\)
\(\Leftrightarrow m=\dfrac{57}{7}\) (thỏa mãn m\(\ne-1\))
Vậy ..

b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)

\(\Delta=\left[-2\left(m+1\right)\right]^2-4\left(m^2+4\right)\)
\(=4m^2+8m+4-4m^2-16\)
\(=8m-12\)
Để pt có 2 nghiệm thì \(\Delta>0\)
\(\Leftrightarrow8m-12>0\Leftrightarrow m>\dfrac{3}{2}\)
Theo hệ thức Vi-ét,ta có: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\left(1\right)\\x_1x_2=m^2+4\end{matrix}\right.\)
\(\left(1\right)\rightarrow x_2=2\left(m+1\right)-x_1\)
\(x_1^2+2\left(m+1\right)x_2=3m^2+16\)
\(\Leftrightarrow x_1^2+2\left(m+1\right)\left[2\left(m+1\right)-x_1\right]=3m^2+16\)
\(\Leftrightarrow x_1^2+4\left(m+1\right)^2-2x_1\left(m+1\right)=3m^2+16\)
\(\Leftrightarrow x_1^2+4m^2+8m+4-2x_1\left(m+1\right)=3m^2+16\)
\(\Leftrightarrow x_1^2+m^2+8m-12-2x_1\left(m+1\right)=0\)
\(\Leftrightarrow x_1^2+m^2+8m-12-x_1\left(x_1+x_2\right)=0\)
\(\Leftrightarrow x_1^2+m^2+8m-12-x_1^2-x_1x_2=0\)
\(\Leftrightarrow m^2+8m-12-m^2-4=0\)
\(\Leftrightarrow m^2+8m-16=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=-4+4\sqrt{2}\left(tm\right)\\m=-4-4\sqrt{2}\left(ktm\right)\end{matrix}\right.\)
Vậy \(m=\left\{-4+4\sqrt{2}\right\}\)

Lời giải:
Để pt có 2 nghiệm $x_1,x_2$ thì:
$\Delta'=1-(m+2)\geq 0\Leftrightarrow m\leq -1$
Áp dụng định lý Viet:
$x_1+x_2=2$
$x_1x_2=m+2$
Khi đó:
\(\text{VT}=\sqrt{[(x_1-2)^2+mx_2][(x_2-2)^2+mx_1]}=\sqrt{[(x_1-x_1-x_2)^2+mx_2][(x_2-x_1-x_2)^2+mx_1]}\)
\(=\sqrt{(x_2^2+mx_2)(x_1^2+mx_1)}=\sqrt{x_1x_2(x_2+m)(x_1+m)}\)
\(=\sqrt{x_1x_2[x_1x_2+m(x_1+x_2)+m^2]}\)
\(=\sqrt{(m+2)[m+2+2m+m^2]}=\sqrt{(m+2)(m^2+3m+2)}\)
\(=\sqrt{(m+2)^2(m+1)}\)
Lại có:
\(\text{VP}=|x_1-x_2|\sqrt{x_1x_2}=\sqrt{(x_1-x_2)^2x_1x_2}=\sqrt{[(x_1+x_2)^2-4x_1x_2]x_1x_2}\)
\(=\sqrt{-4(m+1)(m+2)}\)
YCĐB thỏa mãn khi:
$\sqrt{(m+1)(m+2)^2}=\sqrt{-4(m+1)(m+2)}$
$\Leftrightarrow (m+1)(m+2)^2=-4(m+1)(m+2)$
$\Leftrightarrow m=-1; m=-2$ hoặc $m=-6$ (đều tm)
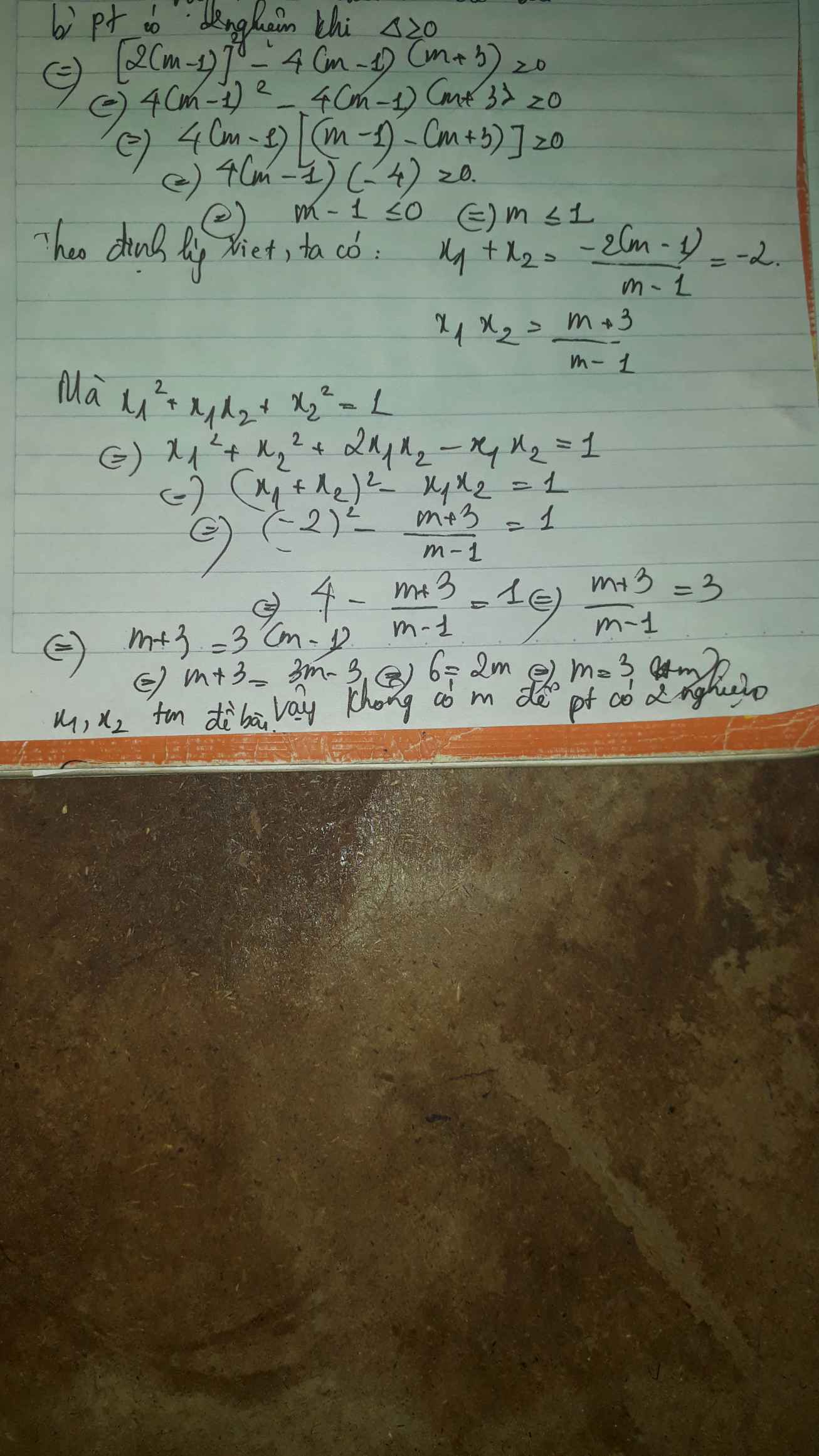

Để pt có 2 nghiệm
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne-1\\\Delta'=\left(m+1\right)^2-\left(m+1\right)\left(m-3\right)\ge0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne-1\\4m+4\ge0\end{matrix}\right.\) \(\Rightarrow m>-1\)
Theo Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=\frac{m-3}{m+1}\end{matrix}\right.\)
Sửa đề: \(\left(4x_1+1\right)\left(4x_2+1\right)=18\)
\(\Leftrightarrow16x_1x_2+4\left(x_1+x_2\right)+1=18\)
\(\Leftrightarrow\frac{16\left(m-3\right)}{m+1}+9=18\)
\(\Leftrightarrow16\left(m-3\right)=9\left(m+1\right)\Rightarrow m=\frac{57}{7}\)