tìm m để f(x) = x2 -2( m-1) x+m-2≤0 ∀ x∈ [ 0,1]
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(f\left(x\right)=\left(m-4\right)x^2+\left(m+1\right)x+2m-1\)
\(f\left(x\right)< 0,\forall x\in R\Leftrightarrow\left\{{}\begin{matrix}a< 0\\\Delta< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m-4< 0\\\left(m+1\right)^2-4\left(m-4\right)\left(2m-1\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 4\\m^2+2m+1-4\left(2m^2-m-8m+4\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow m^2+2m+1-8m^2+36m-16< 0\)
\(\Leftrightarrow-7m^2+38m-15< 0\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 4\\\left[{}\begin{matrix}m< \dfrac{3}{7}\\m>5\end{matrix}\right.\end{matrix}\right.\)
\(KL:m\in\left(5;+\infty\right)\)

\(f\left(x\right)>0,\forall x\in R\Leftrightarrow\left\{{}\begin{matrix}a>0\\\Delta< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m+1>0\\\left[-2\left(m-1\right)\right]^2-4\left(m+1\right)\left(-m+4\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m>-1\\4\left(m^2-2m+1\right)-4\left(-m^2+4m-m+4\right)< 0\end{matrix}\right.\)
\(\Leftrightarrow4m^2-8m+4+4m^2-12m-16< 0\)
\(\Leftrightarrow8m^2-20m-12< 0\)
\(KL:m\in\left(-1;3\right)\)

Với m=−1m=−1 thì PT f(x)=0f(x)=0 có nghiệm x=1x=1 (chọn)
Với m≠−1m≠−1 thì f(x)f(x) là đa thức bậc 2 ẩn xx
f(x)=0f(x)=0 có nghiệm khi mà Δ′=m2−2m(m+1)≥0Δ′=m2−2m(m+1)≥0
⇔−m2−2m≥0⇔m(m+2)≤0⇔−m2−2m≥0⇔m(m+2)≤0
⇔−2≤m≤0⇔−2≤m≤0
Tóm lại để f(x)=0f(x)=0 có nghiệm thì m∈[−2;0]

Do \(a=1>0\) nên \(f\left(x\right)\ge0;\forall x\) khi:
\(\Delta'=\left(m-3\right)^2-4m\le0\)
\(\Leftrightarrow m^2-10m+9\le0\)
\(\Rightarrow1\le m\le9\)

Tìm m để f(x) < 0 vô nghiệm
⇔ f(x) ≥ 0 ∀ x ∈ R
⇔ Δ' ≤ 0
⇔ m2 - 3m - 4 ≤ 0
⇔ -1 ≤ m ≤ 4
Vậy bpt có nghiệm khi \(\left[{}\begin{matrix}m< -1\\m>4\end{matrix}\right.\)

f(x) = (m + 1) x 2 - 2(3 - 2m)x + m + 1 ≥ 0 (1)
Với m = -1:
(1) ⇔ -10x ≥ 0 ⇔ x ≤ 0
Vậy với m = -1 bất phương trình (1) có nghiệm x ≤ 0
Suy ra, m = -1 (loại)
Với m ≠ -1:
f(x) = (m +1 ) x 2 - 2(3 - 2m)x + m + 1
Δ' = [-(3 - 2m) ] 2 - (m + 1)(m + 1) = (2m - 3 ) 2 - (m + 1 ) 2
= (2m - 3 + m + 1)(2m - 3 - m - 1) = (3m - 2)(m - 4)
Để bất phương trình (1) vô nghiệm thì:
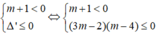

Vậy không có giá trị nào của m để bất phương trình (1) vô nghiệm

f(x) = (m-2) x 2 - 2mx + m + 1 > 0 (*)
Với m = 2 thì bất phương trình (*) trở thành:
f(x) = -4x + 3 > 0 ⇔ x < 3/4
Vậy với m = 2 thì bất phương trình (*) có nghiệm x < 3/4 nên m = 2 (loại)
Với m ≠ 2 thì bất phương trình (*) vô nghiệm khi và chỉ khi
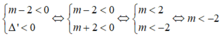
Vậy với m < -2 thì bất phương trình (*) vô nghiệm

ĐKXĐ: \(x\ge0\)
\(\left(x^2-x-m\right)\sqrt{x}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x^2-x-m=0\left(1\right)\end{matrix}\right.\)
Giả sử (1) có nghiệm thì theo Viet ta có \(x_1+x_2=1>0\Rightarrow\left(1\right)\) luôn có ít nhất 1 nghiệm dương nếu có nghiệm
Do đó:
a. Để pt có 1 nghiệm \(\Leftrightarrow\left(1\right)\) vô nghiệm
\(\Leftrightarrow\Delta=1+4m< 0\Leftrightarrow m< -\dfrac{1}{4}\)
b. Để pt có 2 nghiệm pb
TH1: (1) có 1 nghiệm dương và 1 nghiệm bằng 0
\(\Leftrightarrow m=0\)
TH2: (1) có 2 nghiệm trái dấu
\(\Leftrightarrow x_1x_2=-m< 0\Leftrightarrow m>0\)
\(\Rightarrow m\ge0\)
c. Để pt có 3 nghiệm pb \(\Leftrightarrow\) (1) có 2 nghiệm dương pb
\(\Leftrightarrow\left\{{}\begin{matrix}\Delta=1+4m>0\\x_1x_2=-m>0\\\end{matrix}\right.\) \(\Leftrightarrow-\dfrac{1}{4}< m< 0\)
f(x) ≤ 0 ∀ x∈ \([0;1]\) ⇔ \(\left\{{}\begin{matrix}\Delta'>0\\\left(x_1-1\right)\left(x_2-1\right)\ge0\\x_1+x_2\ge2\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}m^2-3m+3>0\\-m+1\ge0\\2m-2\ge2\end{matrix}\right.\)
⇔\(\left\{{}\begin{matrix}\forall m\\m\le1\\m\ge2\end{matrix}\right.\)
⇔∅. Vậy không có giá trị m thỏa mãn