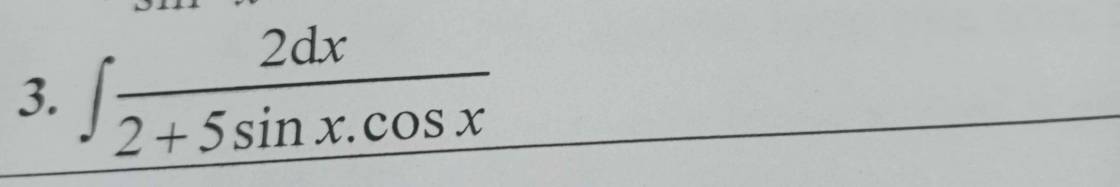mọi người giải giúp mk với
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
MN
25 tháng 8 2021
5.
\(n_{Na}=a\left(mol\right)\)
\(Na+H_2O\rightarrow NaOH+\dfrac{1}{2}H_2\)
\(a...............a.....0.5a\)
\(m_{\text{dung dịch sau phản ứng}}=23a+500-0.5a\cdot2=22a+500\left(g\right)\)
\(m_{NaOH}=40a\left(g\right)\)
\(C\%_{NaOH}=\dfrac{40a}{22a+500}\cdot100\%=20\%\)
\(\Rightarrow a=2.8\)
\(b.\)
\(n_{H^+}=10^{-3}\cdot V\cdot\left(1+0.5\cdot2\right)=2\cdot10^{-3}V\left(mol\right)\)
\(\Rightarrow n_{NaOH}=2\cdot10^{-3}V=2.8\left(mol\right)\)
\(\Rightarrow V=1400\)
4 tháng 7 2021
Bài 5 :
a, ĐKXĐ ; \(\left\{{}\begin{matrix}x\ge0\\x\ne1\end{matrix}\right.\)
Ta có : \(P=1:\left(\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}+\dfrac{\sqrt{x}+1}{x+\sqrt{x}+1}-\dfrac{\sqrt{x}+1}{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\right)\)
\(=1:\left(\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}+\dfrac{x-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}-\dfrac{x+\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right)\)
\(=1:\left(\dfrac{x+2+x-1-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right)\)
\(=1:\left(\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right)\)
\(=\dfrac{x+\sqrt{x}+1}{\sqrt{x}}\)
b, - Xét \(P-3=\dfrac{x+\sqrt{x}+1-3\sqrt{x}}{\sqrt{x}}=\dfrac{\left(\sqrt{x}-1\right)^2}{\sqrt{x}}>0\)
\(\Rightarrow P>3\)
4 tháng 7 2021
\(P=1:\left(\dfrac{x+2}{x\sqrt{x}-1}+\dfrac{\sqrt{x}+1}{x+\sqrt{x}+1}-\dfrac{\sqrt{x}+1}{x-1}\right)\) (Đk:\(x\ge0;x\ne1\))
\(=1:\left[\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}+\dfrac{\left(\sqrt{x}+1\right)^2}{\left(\sqrt{x}+1\right)\left(x+\sqrt{x}+1\right)}-\dfrac{\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\right]\)
\(=1:\left[\dfrac{x+2}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}+\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}-\dfrac{x+\sqrt{x}+1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\right]\)
\(=1:\dfrac{x+2+x-1-x-\sqrt{x}-1}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=1:\dfrac{x-\sqrt{x}}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=1:\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-1\right)\left(x+\sqrt{x}+1\right)}\)
\(=\dfrac{x+\sqrt{x}+1}{\sqrt{x}}=\sqrt{x}+1+\dfrac{1}{\sqrt{x}}\)
b) Áp dụng AM-GM có:
\(\sqrt{x}+\dfrac{1}{\sqrt{x}}\ge2\sqrt{\sqrt{x}.\dfrac{1}{\sqrt{x}}}=2\)
Dấu "=" xảy ra khi \(\sqrt{x}=\dfrac{1}{\sqrt{x}}\Leftrightarrow x=1\left(ktm\right)\)
\(\Rightarrow\)Dấu "=" không xảy ra
\(\Rightarrow\sqrt{x}+\dfrac{1}{\sqrt{x}}>2\)\(\Rightarrow\sqrt{x}+1+\dfrac{1}{\sqrt{x}}>3\)
hay P>3
Vậy...
23 tháng 1 2022
1) \(HPT.\) \(\Leftrightarrow\left\{{}\begin{matrix}6\sqrt{x}+4\sqrt{y}=32.\\6\sqrt{x}-9\sqrt{y}=-33.\end{matrix}\right.\) \(\left(x\ge0;y\ge0\right).\)
\(\Leftrightarrow\left\{{}\begin{matrix}3\sqrt{x}+2\sqrt{y}=16.\\13\sqrt{y}=65.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}\sqrt{x}=2.\\\sqrt{y}=5.\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=4.\\y=25.\end{matrix}\right.\) (TM).
2) \(HPT.\Leftrightarrow\) \(\left\{{}\begin{matrix}3\left|x\right|+12\left|y\right|=54.\\3\left|x\right|+\left|y\right|=10.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}\left|x\right|+4\left|y\right|=18.\\\left|y\right|=4.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}\left|x\right|=2.\\\left|y\right|=4.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}\left[{}\begin{matrix}x=2.\\x=-2.\end{matrix}\right.\\\left[{}\begin{matrix}y=4.\\y=-4.\end{matrix}\right.\end{matrix}\right.\)
TH
1
KR
28 tháng 1 2023
\(I=\int\dfrac{2}{2+5sinxcosx}dx=\int\dfrac{2sec^2x}{2sec^2x+5tanx}dx\\ =\int\dfrac{2sec^2x}{2tan^2x+5tanx+2}dx\)
We substitute :
\(u=tanx,du=sec^2xdx\\ I=\int\dfrac{2}{2u^2+5u+2}du\\ =\int\dfrac{2}{2\left(u+\dfrac{5}{4}\right)^2-\dfrac{9}{8}}du\\ =\int\dfrac{1}{\left(u+\dfrac{5}{4}\right)^2-\dfrac{9}{16}}du\\ \)
Then,
\(t=u+\dfrac{5}{4}\\I=\int\dfrac{1}{t^2-\dfrac{9}{16}}dt\\ =\int\dfrac{\dfrac{2}{3}}{t-\dfrac{3}{4}}-\dfrac{\dfrac{2}{3}}{t+\dfrac{3}{4}}dt\)
Finally,
\(I=\dfrac{2}{3}ln\left(\left|\dfrac{t-\dfrac{3}{4}}{t+\dfrac{3}{4}}\right|\right)+C=\dfrac{2}{3}ln\left(\left|\dfrac{tanx+\dfrac{1}{2}}{tanx+2}\right|\right)+C\)
4D
2
18 tháng 1 2022
vì nó lấy từ một loại cây có chứa nhiều hàm lượng pechoin (cái này mình lấy từ trên mạng nha)
17 tháng 10 2018
a x b = 2/3 = 6/9
a x (b+5) = 28/9
=> 5a = 22/9
=> a = 22/45
b = 15/11
KH
1
4D
2
MH
11 tháng 1 2022
Tham khảo
Ngoài ra trong nhiều cuộc nghiên cứu các nhà y học đã chỉ ra rằng việc thường xuyên sử dụng thức ăn nhanh cũng có khả năng làm tăng lượng triglyceride trong máu và làm giảm lượng cholesterol HDL có lợi. Đây cũng là một yếu tố nguy cơ khác của bệnh tim.
S
11 tháng 1 2022
TK
Ăn nhanh là một thói quen không tốt cho sức khỏe. Khi nhai, các tuyến nước bọt bắt đầu hoạt động, một thông báo được gửi đến cơ thể rằng thức ăn đang được cung cấp và chuẩn bị cho quá trình tiêu hóa. Bạn thậm chí không thể thưởng thức hương vị nếu bạn ăn nhanh. Thực phẩm không được nghiền nát. Thời gian tiêu hóa thức ăn càng nhiều thì sức căng của dạ dày càng lớn. Sẽ gây ra các bệnh về dạ dày, ảnh hưởng đến sức khỏe mà không thể thưởng thức được vị ngon của thức ăn.