sin25x-cos23x+1=0
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(1-sin^23x-5sin3x+5=0\)
\(\Leftrightarrow-sin^23x-5sin3x+6=0\)
\(\Rightarrow\left[{}\begin{matrix}sin3x=1\\sin3x=-6< -1\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow3x=\dfrac{\pi}{2}+k2\pi\)
\(\Rightarrow x=\dfrac{\pi}{6}+\dfrac{k2\pi}{3}\)


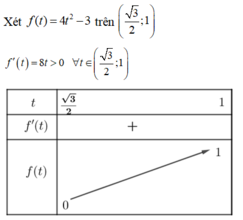
Dựa vào bảng biến thiên phương trình có nghiệm khi 0<m<1
Chọn D

Đáp án C
Ta có: cos 2 3 x 1 + cos 6 x 2 = 4 cos 3 2 x − 3 cos 2 x + 1 2 và cos 4 x = 2 cos 2 2 x − 1
Khi đó, phương trình đã cho
⇔ 2 cos 2 2 x − 1 = 4 cos 3 2 x − 3 cos 2 x + 1 2 + 1 − cos 2 x 2 m
⇔ 4 cos 2 2 x − 2 = 4 cos 3 2 x − 3 cos 2 x + 1 + 1 − cos 2 x m
⇔ cos 2 x − 1 m = 4 cos 3 2 x − 4 cos 2 2 x − 3 cos 2 x + 3
Đặt t = cos 2 x , với x ∈ 0 ; π 12 → t ∈ 3 2 ; 1 do đó: * ⇔ m 4 t 3 − 4 t 2 − 3 t + 3 t − 1 = 4 t 2 − 3
Xét hàm số f t = 4 t 2 − 3 trên khoảng 3 2 ; 1 → min f t = 0 max f t = 1
Vậy để phương trình m = f t có nghiệm khi và chỉ khi m ∈ 0 ; 1

Đáp án C
Ta có c os 2 3 x = 1 + c os 6 x 2 = 4 c os 3 2 x − 3 c os 2 x + 1 2
và c os 4 x = 2 c os 2 2 x − 1
Khi đó, phương trình đã cho
⇔ 2 c os 2 2 x − 1 = 4 c os 3 2 x − 3 c os 2 x + 1 2 + 1 − c os 2 x 2 m
⇔ 4 c os 2 2 x − 2 = 4 c os 3 2 x − 3 c os 2 x + 1 + 1 − c os 2 x m ⇔ c os 2 x − 1 m = 4 c os 3 2 x − 4 c os 2 2 x − 3 c os 2 x + 3
Đặt t = c os 2 x , với x ∈ 0 ; π 12 → t ∈ 3 2 ; 1 ,
do đó (*) ⇔ m = 4 t 3 − 4 t 2 − 3 t + 3 t − 1 = 4 t 2 − 3.
Xét hàm số f t = 4 t 2 − 3 trên khoảng 3 2 ; 1 → min f t = 0 max f t = 1 .
Vậy để phương trình m = f t có nghiệm khi và chỉ khi m ∈ 0 ; 1 .

\(\Leftrightarrow1-cos6x-1-cos8x=1-cos10x-1-cos12x\)
\(\Leftrightarrow cos10x+cos12x-cos6x-cos8x=0\)
\(\Leftrightarrow cos11x.cosx+cos7x.cosx=0\)
\(\Leftrightarrow cosx\left(cos11x+cos7x\right)=0\)
\(\Leftrightarrow cosx.cos9x.cos2x=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cos2x=0\\cos9x=0\end{matrix}\right.\) \(\Leftrightarrow...\)
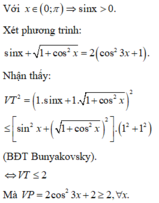


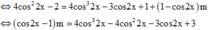
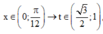 , do đó (*)
, do đó (*) 
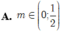


\(\Leftrightarrow sin^25x+sin^23x=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin3x=0\\sin5x=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{k\pi}{3}\\x=\dfrac{k\pi}{5}\end{matrix}\right.\)
\(\Leftrightarrow x=k\pi\)