Tính đạo hàm cấp hai của hàm số y = tanx
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Đáp án D
Ta có: y ' = 1 cos 2 x ;
y ' ' = - 1 cos 4 x . cos 2 x ' = - 2 cos ( - sin x ) cos 4 x = 2 sin x cos 3 x

y = tan x + cot x ⇒ y ' = ( tan x + cot x ) ' = ( tan x ) ' + ( cot x ) ' = 1 cos 2 x − 1 sin 2 x
Chọn đáp án D

Ta có: y ' = 2 x . sin x + x 2 . cos x
y
'
'
=
2
sin
x
+
2
x
.
c
osx + 2x.cosx - x
2
s
inx
=
(
2
−
x
2
)
sin
x
+
4
x
.
cos
x
Chọn đáp án C

Chọn C.
y' = cos2x – 2xsin2x;
y” = -2sin2x – (2sin2x + 4xcos2x) = -4sin2x – 4xcos2x.

y ' = 2 2 − 3 x 2 x + 1 . 2 − 3 x 2 x + 1 ' = 2 2 − 3 x 2 x + 1 . − 3 2 x + 1 − 2 2 − 3 x 2 x + 1 2 = 2 2 − 3 x 2 x + 1 . − 7 2 x + 1 2 = − 14 2 x + 1 2 . 2 − 3 x 2 x + 1
Chọn đáp án A
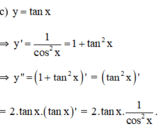
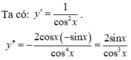
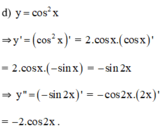
y′n =2sinxcos3xy″=2sinxcos3x
\(y^n=\)\(\frac{2\sin x}{\cos^3x}\)