{3/2x-1 -1/3y-2=11
{6/2x+1 + 5/3y-2=8
Giải hệt phương trình
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


9: \(\left\{{}\begin{matrix}3x-2=y\\2x+3y=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x-y=2\\2x+3y=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}6x-2y=4\\6x+9y=18\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-11y=-14\\3x-y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=\dfrac{14}{11}\\x=\dfrac{y+2}{3}=\dfrac{\dfrac{14}{11}+2}{3}=\dfrac{12}{11}\end{matrix}\right.\)
\(9,\Leftrightarrow\left\{{}\begin{matrix}3x-2=y\\2x+3\left(3x-2\right)=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}3x-2=y\\11x=12\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{12}{11}\\y=\dfrac{14}{11}\end{matrix}\right.\)
\(10,\Leftrightarrow\left\{{}\begin{matrix}2x=2-3y\\2\left(2-3y\right)-y-1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x=2-3y\\4-6y-y-1=0\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{5}{14}\\y=\dfrac{3}{7}\end{matrix}\right.\)

giải
A=(3x-5)(2x+11)-(2x+3)(3x+7)
=6x^2+33x-10x-55-(6x^2+14x+9x+21)
=6x^2+33x-10x-55-6x^2-14x-9x-21
= -76
vậy biểu thức không phụ thuộc vào biến x,y
B=(2x+3)(4x^2-6x+9)-2(4x^3-1)
=8x^3-12x^2+18x+12x^2-18x+27-8x^3+2
=29
vậy biểu thức không phụ thuộc vào biến x

Bài 3:
3: \(6x\left(x-y\right)-9y^2+9xy\)
\(=6x\left(x-y\right)+9xy-9y^2\)
\(=6x\left(x-y\right)+9y\left(x-y\right)\)
\(=\left(x-y\right)\left(6x+9y\right)\)
\(=3\left(2x+3y\right)\left(x-y\right)\)
Bài 4:
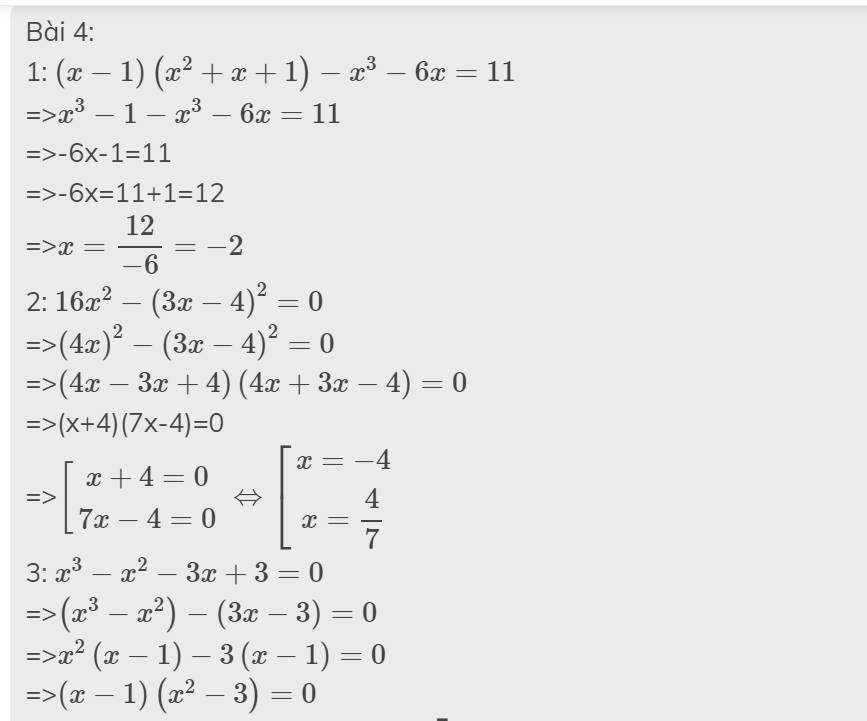

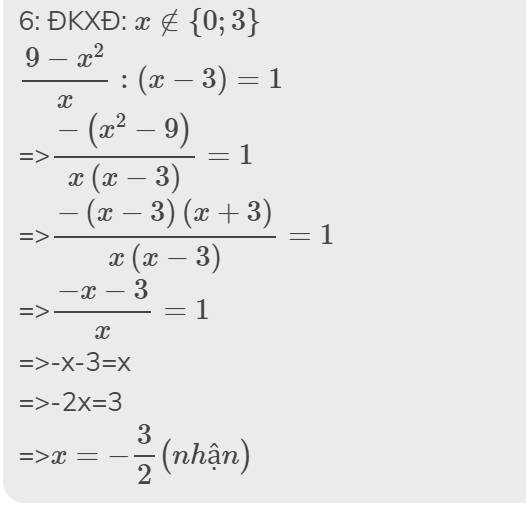

\(_{\hept{2y^2}-x^2+1=\sqrt{3y^4-4x^2+6y^2-2x^2y^2\left(2\right)}}2x^4+3x^3+45x=27x^2\left(1\right)\)
ĐK: \(2y^2+1\ge1\)
Phương trình 2 tương đương:
\(\left(2y^2-x^2+1\right)^2=3y^4-4x^2+6x^2-2x^2y^2\)
\(\Leftrightarrow y^4+2x^2-2x^2y^2+x^{2+2}+1-2y^2=0\)
Các lập phương được cấu tạo từ \(x^2y^2\)nên :
\(\Leftrightarrow\left(y^4-2x^2y^2+y^4\right)-2\left(y^2-x^2\right)+1=0\)
Đảo chiều:
\(\Leftrightarrow\left(y^2-x^2-1\right)^2=0\)
\(\Leftrightarrow y^2=x^2+1\left(3\right)\)
Thế \(x^2+1=y^2\)vào phương trình (1) ta có :
\(2x^4+3x^3+45x=27\left(x^2+1\right)\)
\(\Leftrightarrow2x^4+3x^3-27x^2+45x-27=0\)
\(\Leftrightarrow\left(x-\frac{3}{2}\right)\left(2x^3+6x^2-18x+18\right)=0\)
Chuyển: \(x=\frac{3}{2}\Rightarrow y=\frac{\sqrt{13}}{2}\)
\(\Leftrightarrow[x=-\sqrt[3]{16-\sqrt[3]{4}}-1\Rightarrow y=\sqrt{\left(\sqrt[3]{16}+\sqrt[3]{4}+1\right)^2+1}\)
