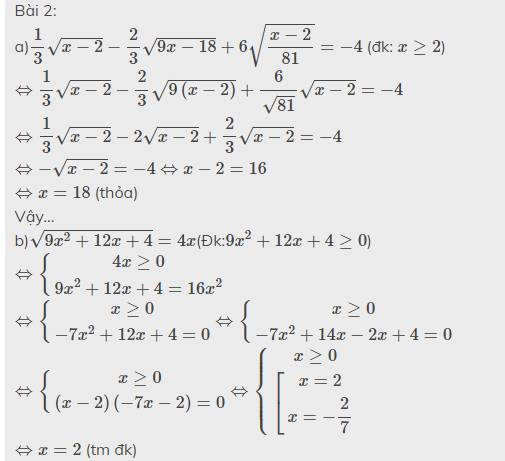BÀI 1
a. Thực hiện phép tính: \(\sqrt{75}-4\sqrt{48}+\sqrt{108}\)
b. Giải phương trình: \(x^2-\left(2+\sqrt{7}\right)x+2\sqrt{7}=0\)
BÀI 2
Trong một kỳ thi Tú tài, kết quả của trường phổ thông trung học A được biết như sau: số học sinh thi đỗ nhiều hơn số học sinh thi hỏng là 64 em, số học sinh thi đỗ bằng \(\frac{5}{9}\)lần tổng số học sinh dự thi. Hỏi có bao nhiêu học sinh thi đỗ, bao nhiêu học sinh thi hỏng.
Giúp mình vs ạ! cảm ơn rất nhiềuuuuu