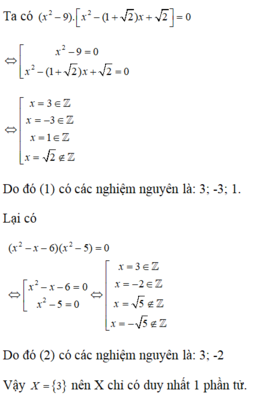1. Cho tập X = {x ϵ Z/ (x2 - 9).[x2 - (1 \(\sqrt{2}\))x \(\sqrt{2}\)] = 0}. Hỏi tập X có bao nhiêu phần tử? 2. Tập A = {0

HP

Hương Phạm

15 tháng 12 2019

1. Cho tập X = {x ϵ Z/ (x2 - 9).[x2 - (1 + \(\sqrt{2}\))x + \(\sqrt{2}\)] = 0}. Hỏi tập X có bao nhiêu phần tử? 2. Tập A = {0;2;4;6} có bao nhiêu tập hợp con có đúng hai phần tử? 3. Trong số 45 học sinh của lớp 10A có 15 bạn được xếp loại học lực giỏi, 20 bạn được xếp loại hạnh kiểm tốt, trong đó có 10 bạn vừa được học sinh giỏi vừa được hạnh kiểm tốt. Khi đó lớp 10A có bao nhiêu bạn được khen...

Đọc tiếp

#Toán lớp 10

0

HH

Hân Hân

27 tháng 9 2021

Hãy liệt kê cấc phần tử của tập X={x∈Q | (x²-x-6)(x²-5)=0}

A. x={-\(\sqrt{5}\); -2; \(\sqrt{5}\); 3}

B. x={\(\sqrt{5}\); 3}

C. x={-2; 3}

D. x={-\(\sqrt{5}\); \(\sqrt{5}\)}

#Toán lớp 10

2

LL

Lấp La Lấp Lánh

27 tháng 9 2021

\(\left(x^2-x-6\right)\left(x^2-5\right)=0\)

\(\Rightarrow\left(x-3\right)\left(x+2\right)\left(x-\sqrt{5}\right)\left(x+\sqrt{5}\right)=0\)

\(\Rightarrow\left[{}\begin{matrix}x=3\\x=-2\\x=\sqrt{5}\\x=-\sqrt{5}\end{matrix}\right.\)

Mà \(x\in Q\)

\(\Rightarrow x=\left\{-2;3\right\}\)

Đúng(2)

NT

nguyễn thị hương giang

27 tháng 9 2021

Pt\(\Leftrightarrow\)\(\left[{}\begin{matrix}x^2-x-6=0\\x^2-5=0\end{matrix}\right.\)\(\Leftrightarrow\)\(\left[{}\begin{matrix}3\\-2\\-\sqrt{5}\\\sqrt{5}\end{matrix}\right.\)

Đáp án A

Đúng(0)

HH

Hận Hâh

15 tháng 9 2021

Trong các tập hợp sau đây, tập nào là tập rỗng: a/A={x ∈ Z | |x| < 1}b/B={x ∈ R | x2 - x + 1= 0}c/C={x ∈ N | x2 + 7x + 12 = 0} Cho tập hợp A ={1;2;3}a/ Viết tất cả các tập hợp con gồm 2 phần tử của tập hợp Ab/ Viết tất cả các tập hợp con của tập hợp ATìm tất cả các tập X sao cho{1;3} ⊂...

Đọc tiếp

#Toán lớp 10

2

OY

OH-YEAH^^

15 tháng 9 2021

Tập C là tập rỗng

Đúng(0)

NH

Nguyễn Hoàng Minh

15 tháng 9 2021

Tập hợp C rỗng vì \(x^2+7x+12=0\Leftrightarrow x\in\left\{-3;-4\right\}\notin N\)

\(a,\left\{1;2\right\};\left\{1;3\right\};\left\{2;3\right\}\\ b,\left\{1\right\};\left\{2\right\};\left\{3\right\};\left\{1;2\right\};\left\{1;3\right\};\left\{2;3\right\};\left\{1;2;3\right\}\)

\(X=\left\{1;3\right\}\\ X=\left\{1;2;3\right\}\\ X=\left\{1;3;4\right\}\\ X=\left\{1;3;5\right\}\\ X=\left\{1;2;3;4\right\}\\ X=\left\{1;2;3;5\right\}\\ X=\left\{1;3;4;5\right\}\\ X=\left\{1;2;3;4;5\right\}\)

Đúng(1)

TT

trương tấn thanh

22 tháng 9 2021

Bài 1. Liệt kê các phần tử của tập hợp sau:a) A = {x Î N | x < 6} b) B = {x Î N | 1 < x £ 5}c) C = {x Î Z , |x| £ 3} d) D = {x Î Z | x2 - 9 = 0}e) E = {x Î R | (x - 1)(x2 + 6x + 5) = 0} f) F = {x Î R | x2 - x + 2 = 0}g) G = {x Î N | (2x - 1)(x2 - 5x + 6) = 0} h) H = {x | x = 2k với k Î Z và -3 < k < 13}i) I = {x Î Z | x2 > 4 và |x| < 10} j) J = {x | x = 3k với k Î Z và -1 < k < 5}k) K = {x Î R | x2 - 1 = 0 và x2 - 4x + 3 = 0} l) L = {x Î Q | 2x - 1 = 0 hay x2 - 4 =...

Đọc tiếp

#Toán lớp 10

1

NL

Nguyễn Lê Phước Thịnh

22 tháng 9 2021

a: \(A=\left\{0;1;2;3;4;5\right\}\)

b: \(B=\left\{2;3;4;5\right\}\)

c: \(C=\left\{0;1;-1;2;-2;3;-3\right\}\)

Đúng(1)

KB

không bít

20 tháng 11 2021

Viết tập hợp A gồm các số tự nhiên x sao cho x + 3 = 12. Tập hợp A có bao nhiêu phần tử?

A. A = {9}, A có 1 phần tử.

B. A = {9}, A có 2 phần tử.

C. A = {0}, A có 1 phần tử.

D. A = {0; 9}, A có 2 phần tử.

#Toán lớp 6

3

H

๖ۣۜHả๖ۣۜI

20 tháng 11 2021

A

Đúng(1)

MH

Minh Hồng

20 tháng 11 2021

A

Đúng(1)

HN

Hoàng Nguyễn

18 tháng 10 2023

Viết mỗi tập hợp sau bằng cách liệt kê các phần tử

a) A= {x ∈ R | (2x – x²)( 3x – 2) = 0}

b, B = { x∈ Z | 2x³-3x²-5x = 0 }

c , C= { x ∈ Z | 2x² -75x -77 = 0 }

d , D = { x ∈ R | (x² - x - 2 ) (x² - 9 ) = 0 } .

#Toán lớp 10

2

KT

『Kuroba ム Tsuki Ryoo ︵²ᵏ⁷』

18 tháng 10 2023

`#3107.101107`

a,

\(\text{A = }\left\{x\in R\text{ | }\left(2x-x^2\right)\left(3x-2\right)=0\right\}\)

`<=> (2x - x^2)(3x - 2) = 0`

`<=>`\(\left[{}\begin{matrix}2x-x^2=0\\3x-2=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x\left(2-x\right)=0\\3x=2\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\2-x=0\\x=\dfrac{2}{3}\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\x=2\\x=\dfrac{2}{3}\end{matrix}\right.\)

Vậy, `A = {0; 2; 2/3}`

b,

\(\text{B = }\left\{x\in R\text{ | }2x^3-3x^2-5x=0\right\}\)

`<=> 2x^3 - 3x^2 - 5x = 0`

`<=> x(2x^2 - 3x - 5) = 0`

`<=>`\(\left[{}\begin{matrix}x=0\\2x^2-3x-5=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\2x^2-2x+5x-5=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\\left(2x^2-2x\right)+\left(5x-5\right)=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\2x\left(x-1\right)+5\left(x-1\right)=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\\left(2x+5\right)\left(x-1\right)=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\2x+5=0\\x-1=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=0\\x=-\dfrac{5}{2}\\x=1\end{matrix}\right.\)

Vậy, `B = {-5/2; 0; 1}.`

Đúng(3)

KT

『Kuroba ム Tsuki Ryoo ︵²ᵏ⁷』

18 tháng 10 2023

c,

\(\text{C = }\left\{x\in Z\text{ | }2x^2-75x-77=0\right\}\)

`<=> 2x^2 - 75x - 77 = 0`

`<=> 2x^2 - 2x + 77x - 77 = 0`

`<=> (2x^2 - 2x) + (77x - 77) = 0`

`<=> 2x(x - 1) + 77(x - 1) = 0`

`<=> (2x + 77)(x - 1) = 0`

`<=>`\(\left[{}\begin{matrix}2x+77=0\\x-1=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}2x=-77\\x=1\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=-\dfrac{77}{2}\\x=1\end{matrix}\right.\)

Vậy, `C = {-77/2; 1}`

d,

\(\text{D = }\left\{x\in R\text{ | }\left(x^2-x-2\right)\left(x^2-9\right)=0\right\}\)

`<=> (x^2 - x - 2)(x^2 - 9) = 0`

`<=>`\(\left[{}\begin{matrix}x^2-x-2=0\\x^2-9=0\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x^2+x-2x-2=0\\x^2=9\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}\left(x^2+x\right)-\left(2x+2\right)=0\\x^2=\left(\pm3\right)^2\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x\left(x+1\right)-2\left(x+1\right)=0\\x=\pm3\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}\left(x-2\right)\left(x+1\right)=0\\x=\pm3\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x-2=0\\x+1=0\\x=\pm3\end{matrix}\right.\)

`<=>`\(\left[{}\begin{matrix}x=2\\x=-1\\x=\pm3\end{matrix}\right.\)

Vậy, `D = {-1; -3; 2; 3}.`

Đúng(2)

K

khoa

10 tháng 9 2021 - olm

1)Viết các tập hợp sau dưới dạng liệt kê các phần tử:

a)A={x N/2 <10} d)D={x Z / 9 x<26}

b)B={x Z/|x|<5} e) E={x Q/x2-x+1=0}

c)C={x R/(x+2)(x-3)(x2-5x+6)=0} f) F={3+2k/k N,k<5}

#Toán lớp 10

0

PT

Pham Trong Bach

19 tháng 12 2017

Gọi X là tập hợp các nghiệm nguyên chung của hai phương trình: ( x 2 − 9 ) . x 2 − ( 1 + 2 ) x + 2 = 0 (1) và ( x 2 − x − 6 ) ( x 2 − 5 ) = 0 (2). Số phẩn tử của X là:

A. 0

B. 3

C. 2

D. 1

#Toán lớp 10

1

CM

Cao Minh Tâm

19 tháng 12 2017

Đáp án D

Đúng(0)

PT

Pham Trong Bach

30 tháng 10 2019

Cho A = {x ∈ Z | x2 < 4}; B = { x ∈ Z | (5x - 3x2)(x2 - 2x - 3)= 0}. Số phần tử của tập hợp (A∪B) \ (A ∩ B) là: A. 0 B. 3 C. 2 D....

Đọc tiếp

#Toán lớp 10

1

CM

Cao Minh Tâm

30 tháng 10 2019

Đáp án: C

A = {0;-1;1}; B = {0;-1;3}

A ∪ B = {0;-1;1;3}; A ∩ B = {0;-1}

(A ∪ B) \ (A ∩ B) = {1;3} => có 2 phần tử.

Đúng(0)

TP

Toàn Phạm

30 tháng 9 2021

Liệt kê phần tử của các tập hợp sau: a) A={x∈Z:(2x2−3x+1)(x+5)=0}. b) B={x∈Q:(x2−3)(x2−3x+2)=0}. c) TậphợpC là số chính phương không vượt quá 50. d) D={n∈N:n là ước chung của 12và18} e) E ={x∈R:x−3=5}

#Toán lớp 10

1

NL

Nguyễn Lê Phước Thịnh

30 tháng 9 2021

a: \(A=\left\{1;-5\right\}\)

b: \(B=\left\{1;2\right\}\)

c: \(C=\left\{0;1;4;9;16;25;36;49\right\}\)

d: \(D=\left\{1;2;3;6\right\}\)

e: E={8}

Đúng(0)

Chúng tôi đề xuất

Tài nguyên

Ứng dụng mobile

Bảng xếp hạng

Các khóa học có thể bạn quan tâm

Yêu cầu VIP