Cho hàm số y=\(\sqrt{x-2m+7}\). Số các giá trị nguyên dương của m để hàm số xác định trên khoảng (5;\(\infty\))
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
22 tháng 10 2021
ĐKXĐ: \(\left\{{}\begin{matrix}x-m+1\ge0\\-x+2m>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge m-1\\x< 2m\end{matrix}\right.\)
\(\Rightarrow x\in[m-1;2m)\)
Để hàm xác định trên (3;4)
\(\Rightarrow\left(3;4\right)\subset[m-1;2m)\)
\(\Rightarrow\left\{{}\begin{matrix}m-1\le3\\2m\ge4\end{matrix}\right.\) \(\Rightarrow2\le m\le4\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 1 2021
\(\Leftrightarrow\left(m+1\right)x\ge-2m-3\)
- Với \(m=-1\) thỏa mãn
- Với \(m>-1\Rightarrow x\ge\dfrac{-2m-3}{m+1}\)
\(\Rightarrow\dfrac{-2m-3}{m+1}\le-3\) \(\Leftrightarrow\dfrac{2m+3}{m+1}-3\ge0\Leftrightarrow\dfrac{-m}{m+1}\ge0\)
\(\Rightarrow-1< m\le0\Rightarrow m=0\)
- Với \(m< -1\Rightarrow x\le\dfrac{-2m-3}{m+1}\Rightarrow\dfrac{-2m-3}{m+1}\ge-1\)
\(\Rightarrow\dfrac{2m+3}{m+1}-1\le0\Leftrightarrow\dfrac{m+2}{m+1}\le0\)
\(\Rightarrow-2\le m< -1\Rightarrow m=-2\)
Vậy \(m=\left\{-2;-1;0\right\}\)
AH
Akai Haruma
Giáo viên
11 tháng 12 2023
Lời giải:
ĐKXĐ: $x\geq 7$ và $x\geq m$
Để TXĐ là $D=[7;+\infty)$ thì m\leq 7$
NV
Nguyễn Việt Lâm
Giáo viên
22 tháng 6 2021
\(y=\dfrac{x^2-m^2+2m+1}{x-m}\) đúng không nhỉ?
\(y'=\dfrac{x^2-2mx+m^2-2m-1}{\left(x-m\right)^2}\)
Hàm đồng biến trên các khoảng xác định khi và chỉ khi:
\(x^2-2mx+m^2-2m-1\ge0\) ; \(\forall x\)
\(\Leftrightarrow\Delta'=m^2-\left(m^2-2m-1\right)\le0\)
\(\Leftrightarrow m\le-\dfrac{1}{2}\)
12 tháng 11 2023
a: TXĐ: D=R\{3}
\(y=\dfrac{2m-x}{x-3}\)
=>\(y'=\dfrac{\left(2m-x\right)'\left(x-3\right)-\left(2m-x\right)\left(x-3\right)'}{\left(x-3\right)^2}\)
\(=\dfrac{-\left(x-3\right)-2m+x}{\left(x-3\right)^2}\)
\(=\dfrac{3-2m}{\left(x-3\right)^2}\)
Để hàm số đồng biến trên từng khoảng xác định thì y'>0 với mọi x thỏa mãn ĐKXĐ
=>\(\dfrac{3-2m}{\left(x-3\right)^2}>0\)
=>3-2m>0
=>2m<3
=>\(m< \dfrac{3}{2}\)
b: TXĐ: D=R\{-m}
\(y=\dfrac{x+3}{x+m}\)
=>\(y'=\dfrac{\left(x+3\right)'\left(x+m\right)-\left(x+3\right)\left(x+m\right)'}{\left(x+m\right)^2}\)
\(=\dfrac{x+m-x-3}{\left(x+m\right)^2}=\dfrac{m-3}{\left(x+m\right)^2}\)
Để hàm số nghịch biến trên từng khoảng xác định thì \(y'< 0\forall x\in TXĐ\)
=>\(\dfrac{m-3}{\left(x+m\right)^2}< 0\)
=>m-3<0
=>m<3
HP
28 tháng 8 2021
1.
\(3cos2x-7=2m\)
\(\Leftrightarrow cos2x=\dfrac{2m-7}{3}\)
Phương trình đã cho có nghiệm khi:
\(-1\le\dfrac{2m-7}{3}\le1\)
\(\Leftrightarrow2\le m\le5\)
HP
28 tháng 8 2021
2.
\(2cos^2x-\sqrt{3}cosx=0\)
\(\Leftrightarrow cosx\left(2cosx-\sqrt{3}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\\cosx=\dfrac{\sqrt{3}}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k\pi\\x=\pm\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)
\(\Rightarrow\) Có 4 nghiệm \(\dfrac{\pi}{2};\dfrac{3\pi}{2};\dfrac{\pi}{6};\dfrac{11\pi}{6}\) thuộc đoạn \(\left[0;2\pi\right]\)
NV
Nguyễn Việt Lâm
Giáo viên
9 tháng 11 2021
ĐKXĐ: \(\left\{{}\begin{matrix}x\ge m-2\\x\ne m\\x< m+5\\\end{matrix}\right.\)
\(\Rightarrow x\in D=[m-2;m)\cup\left(m;m+5\right)\)
Hàm xác định khi \((1;3]\subset D\)
\(\Rightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}m>3\\m-2< 1\end{matrix}\right.\\\left\{{}\begin{matrix}m< 1\\m+5>3\end{matrix}\right.\end{matrix}\right.\) \(\Rightarrow-2< m< 1\)
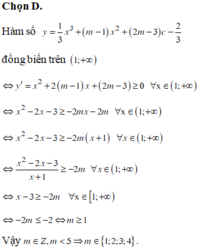
ĐK: \(x\ge2m-7\)
TXĐ: D = [2m-7; \(+\infty\))
Để hàm số xác định trên khoảng (5,\(+\infty\))
\(\Rightarrow2m-7\le5\) \(\Rightarrow m\le6\)