Phân tích đa thức thành nhân tử: (x2 + 2x). (x2 + 4x + 3) - 24
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
22 tháng 11 2019
Cách 1: x2 – 4x + 3
= x2 – x – 3x + 3
(Tách –4x = –x – 3x)
= x(x – 1) – 3(x – 1)
(Có x – 1 là nhân tử chung)
= (x – 1)(x – 3)
Cách 2: x2 – 4x + 3
= x2 – 2.x.2 + 22 + 3 – 22
(Thêm bớt 22 để có HĐT (2))
= (x – 2)2 – 1
(Xuất hiện HĐT (3))
= (x – 2 – 1)(x – 2 + 1)
= (x – 3)(x – 1)
NQ
2
23 tháng 8 2023
\(x^2\left(x-3\right)+4\left(3-x\right)\)\(=x^2\left(x-3\right)-4\left(x-3\right)\)
\(=\left(x^2-4\right)\left(x-3\right)\)\(=\left(x-2\right)\left(x+2\right)\left(x-3\right)\)
23 tháng 8 2023
\(x^2\left(x-3+12-4x\right)\)
\(=x^2\left(x-3\right)-4\left(x-3\right)\)
\(=\left(x-3\right)\left(x^2-4\right)\)
\(=\left(x-3\right)\left(x-2\right)\left(x+2\right)\)
CM
4 tháng 12 2019
x2 + 2x – 3
= x2 + 2x + 1 – 4
= (x + 1)2 – 22
= (x + 1 + 2)(x + 1 – 2)
= (x + 3)(x – 1)
CM
12 tháng 11 2017
Chọn D.
x 4 + 8x = x( x 3 +8)= x( x 3 + 2 3 ) = x(x + 2)( x 2 − 2x + 4)
TL
2
7 tháng 8 2023
a) \(x^4+8x+63\)
\(=x^4+4x^3+9x^2-4x^3-16x^2-36x+7x^2+28x+63\)
\(=x^2\left(x^2+4x+9\right)-4x\left(x^2+4x+9\right)+7\left(x^2+4x+9\right)\)
\(=\left(x^2+4x+9\right)\left(x^2-4x+7\right)\)
7 tháng 8 2023
c) \(\left(x^2+2x+7\right)+\left(x^2-2x+4\right)\left(x^2+2x+3\right)\left(1\right)\)
Ta có : \(x^3-8=\left(x-2\right)\left(x^2+2x+4\right)\)
\(\Rightarrow x^2+2x+4=\dfrac{x^3-8}{x-2}\)
\(\left(1\right)\Rightarrow\left[\left(\dfrac{x^3-8}{x-2}+3\right)\right]+\left(x^2-2x+4\right)\left[\left(\dfrac{x^3-8}{x-2}-1\right)\right]\)
\(=\left[\left(\dfrac{x^3-3x-14}{x-2}\right)\right]+\left(x^2-2x+4\right)\left[\left(\dfrac{x^3-2x-5}{x-2}\right)\right]\)
\(=\dfrac{1}{x-2}\left[x^3-3x-14+\left(x^2-2x+4\right)\left(x^3-2x-5\right)\right]\)
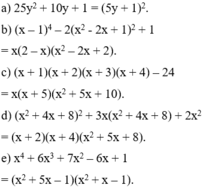
Ta có: (x^2+2x).(x^2+4x+3)-24
=x.(x+2).(x^2+x+3x+3)-24
=x.(x+2).[x.(x+1)+3.(x+1)]-24
=x.(x+2).(x+1).(x+3)-24
=[x.(x+3)].[(x+1).(x+2)]-24
=(x^2+3x).(x^2+3x+2)-24
Đặt x^2+3x=t,phương trình trở thành: t.(t+2)-24=t^2+2t-24=t^2-4t+6t-24=t.(t-4)+6.(t-4)=(t-4).(t+6)=(x^2+3x-4).(x^2+3x+6)
cám ơn bạn ^^