Tìm gtln của hàm số y=sin2x.cosx
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
12 tháng 7 2019
Chọn A.
Ta có y’ = (sin2x)’.cosx + sin2x.(cosx)’ = 2cos2xsinx – sin3x
= sinx(2cos2x – sin2x) = sinx(3cos2x – 1)
Suy ra dy = sinx(3cos2x = 1)dx.
NV
Nguyễn Việt Lâm
Giáo viên
16 tháng 7 2021
24.
\(cos\left(x-\dfrac{\pi}{2}\right)\le1\Rightarrow y\le3.1+1=4\)
\(y_{max}=4\)
26.
\(y=\sqrt{2}cos\left(2x-\dfrac{\pi}{4}\right)\)
Do \(cos\left(2x-\dfrac{\pi}{4}\right)\le1\Rightarrow y\le\sqrt{2}\)
\(y_{max}=\sqrt{2}\)
b.
\(\dfrac{1}{2}sinx+\dfrac{\sqrt{3}}{2}cosx=\dfrac{1}{2}\)
\(\Leftrightarrow cos\left(x-\dfrac{\pi}{6}\right)=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x-\dfrac{\pi}{6}=\dfrac{\pi}{3}+k2\pi\\x-\dfrac{\pi}{6}=-\dfrac{\pi}{3}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{2}+k2\pi\\x=-\dfrac{\pi}{6}+k2\pi\end{matrix}\right.\)
NT
0
PT
1
CM
21 tháng 7 2018
Tập xác định R.
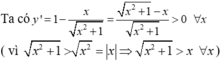
Ta có bảng biến thiên:
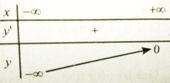
Hàm số không có GTLN trên R . Chọn đáp án C.
HP
10 tháng 9 2021
\(y=\sqrt{3}cosx-sinx=2\left(\dfrac{\sqrt{3}}{2}cosx-\dfrac{1}{2}sinx\right)=2cos\left(x+\dfrac{\pi}{6}\right)\)
Vì \(cos\left(x+\dfrac{\pi}{6}\right)\in\left[-1;1\right]\Rightarrow y=\sqrt{3}cosx-sinx\in\left[-2;2\right]\)
\(\Rightarrow y_{min}=-2\Leftrightarrow cos\left(x+\dfrac{\pi}{6}\right)=-1\Leftrightarrow x+\dfrac{\pi}{6}=\pi+k2\pi\Leftrightarrow x=\dfrac{5\pi}{6}+k2\pi\)
\(y_{max}=2\Leftrightarrow cos\left(x+\dfrac{\pi}{6}\right)=1\Leftrightarrow x+\dfrac{\pi}{6}=k2\pi\Leftrightarrow x=-\dfrac{\pi}{6}+k2\pi\)
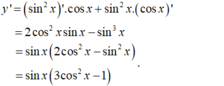
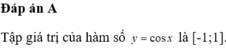
vì: sin bình phương x= 1-cos bình phương x
vậy y = (1-cos bình phương x) nhân cos c
=cos x nhân cos mũ ba x
đặt a= cos x (đk : -1<= a<= 1)
khi đó y =a-a^3
đạo hàm = -3a^2=1
theo bản biến thiên thì trên khoảng -1 đến 1 thì giá trị y đạt dược lớn nhất tại a=căn ba trên 3
a=căn 3 trên ba => cos x=căn ba trên ba => x=........
Có cách nào khác ko, e chưa học đạo hàm. Mới lên 11 thôi 😭