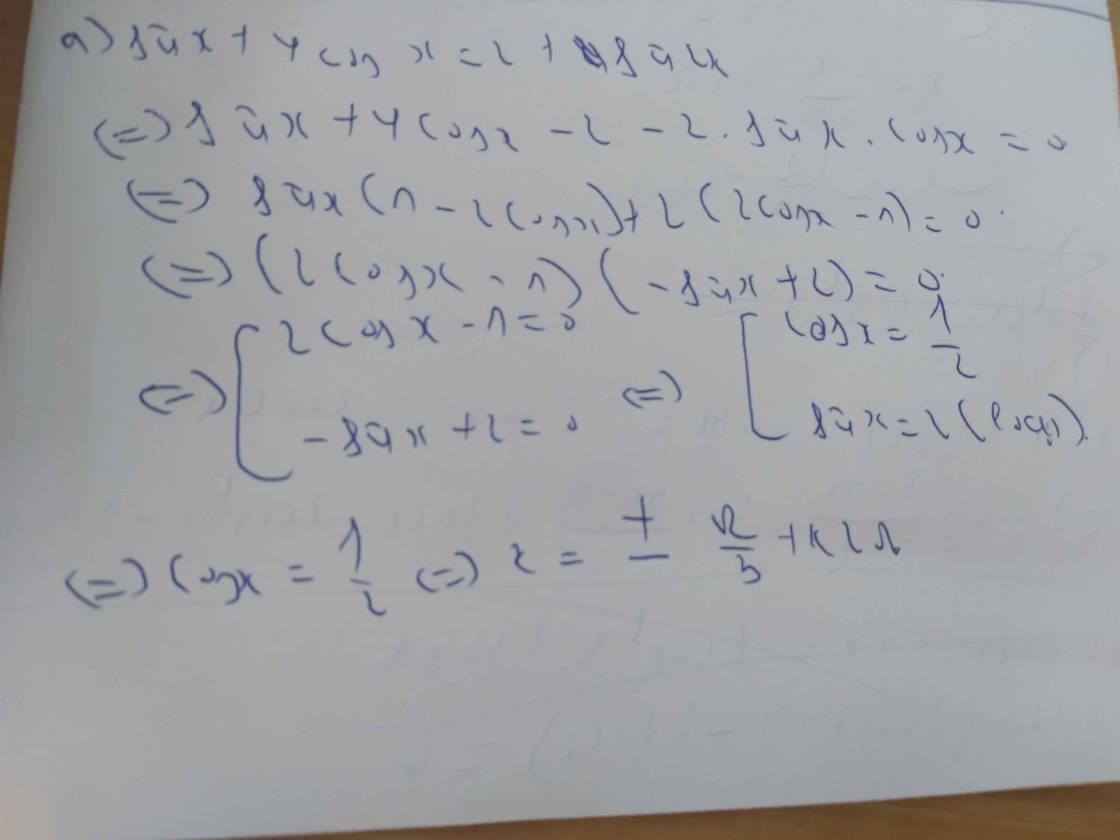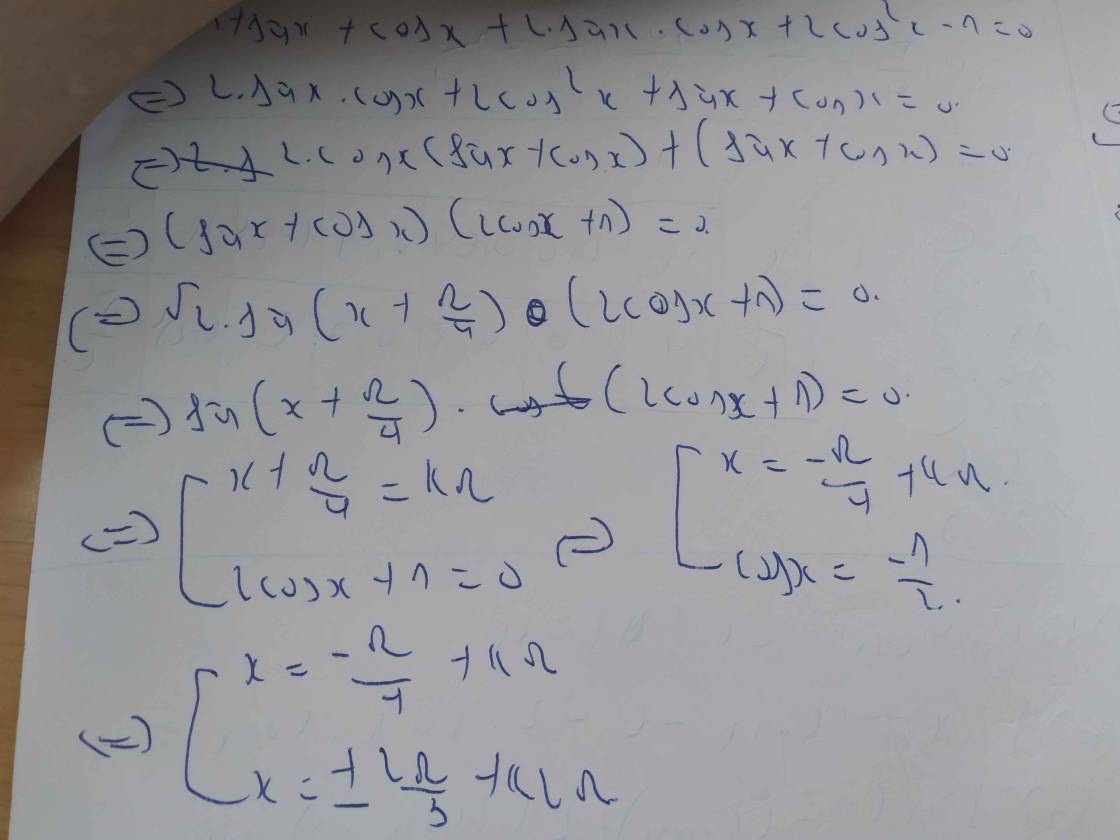\(\int_0^{\dfrac{\pi}{6}}\)\(\dfrac{1-sin2x+cos2x}{sinx-cos2x}dx\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
HP
1 tháng 6 2021
1.
\(2sin\left(x+\dfrac{\pi}{6}\right)+sinx+2cosx=3\)
\(\Leftrightarrow\sqrt{3}sinx+cosx+sinx+2cosx=3\)
\(\Leftrightarrow\left(\sqrt{3}+1\right)sinx+3cosx=3\)
\(\Leftrightarrow\sqrt{13+2\sqrt{3}}\left[\dfrac{\sqrt{3}+1}{\sqrt{13+2\sqrt{3}}}sinx+\dfrac{3}{\sqrt{13+2\sqrt{3}}}cosx\right]=3\)
Đặt \(\alpha=arcsin\dfrac{3}{\sqrt{13+2\sqrt{3}}}\)
\(pt\Leftrightarrow\sqrt{13+2\sqrt{3}}sin\left(x+\alpha\right)=3\)
\(\Leftrightarrow sin\left(x+\alpha\right)=\dfrac{3}{\sqrt{13+2\sqrt{3}}}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\alpha=arcsin\dfrac{3}{\sqrt{13+2\sqrt{3}}}+k2\pi\\x+\alpha=\pi-arcsin\dfrac{3}{\sqrt{13+2\sqrt{3}}}+k2\pi\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=k2\pi\\x=\pi-2arcsin\dfrac{3}{\sqrt{13+2\sqrt{3}}}+k2\pi\end{matrix}\right.\)
Vậy phương trình đã cho có nghiệm:
\(x=k2\pi;x=\pi-2arcsin\dfrac{3}{\sqrt{13+2\sqrt{3}}}+k2\pi\)
HP
1 tháng 6 2021
2.
\(\left(sin2x+cos2x\right)cosx+2cos2x-sinx=0\)
\(\Leftrightarrow2sinx.cos^2x+cos2x.cosx+2cos2x-sinx=0\)
\(\Leftrightarrow\left(2cos^2x-1\right)sinx+cos2x.cosx+2cos2x=0\)
\(\Leftrightarrow cos2x.sinx+cos2x.cosx+2cos2x=0\)
\(\Leftrightarrow cos2x.\left(sinx+cosx+2\right)=0\)
\(\Leftrightarrow cos2x=0\)
\(\Leftrightarrow2x=\dfrac{\pi}{2}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\)
Vậy phương trình đã cho có nghiệm \(x=\dfrac{\pi}{4}+\dfrac{k\pi}{2}\)
TD
0
TD
1
NV
Nguyễn Việt Lâm
Giáo viên
6 tháng 10 2021
\(=\int\limits^{\dfrac{\pi}{4}}_0\dfrac{2cos^2x-1-3\left(1-cos^2x\right)}{cos^2x}dx=\int\limits^{\dfrac{\pi}{4}}_0\dfrac{5cos^2x-4}{cos^2x}dx\)
\(=\int\limits^{\dfrac{\pi}{4}}_0\left(5-\dfrac{4}{cos^2x}\right)dx=\left(5x-4tanx\right)|^{\dfrac{\pi}{4}}_0=...\)
HT
17 tháng 1 2021
Nhìn đề dữ dội y hệt cr của tui z :( Để làm từ từ
Lập bảng xét dấu cho \(\left|x^2-1\right|\) trên đoạn \(\left[-2;2\right]\)
| x | -2 | -1 | 1 | 2 |
| \(x^2-1\) | 0 | 0 |
\(\left(-2;-1\right):+\)
\(\left(-1;1\right):-\)
\(\left(1;2\right):+\)
\(\Rightarrow I=\int\limits^{-1}_{-2}\left|x^2-1\right|dx+\int\limits^1_{-1}\left|x^2-1\right|dx+\int\limits^2_1\left|x^2-1\right|dx\)
\(=\int\limits^{-1}_{-2}\left(x^2-1\right)dx-\int\limits^1_{-1}\left(x^2-1\right)dx+\int\limits^2_1\left(x^2-1\right)dx\)
\(=\left(\dfrac{x^3}{3}-x\right)|^{-1}_{-2}-\left(\dfrac{x^3}{3}-x\right)|^1_{-1}+\left(\dfrac{x^3}{3}-x\right)|^2_1\)
Bạn tự thay cận vô tính nhé :), hiện mình ko cầm theo máy tính
HT
17 tháng 1 2021
2/ \(I=\int\limits^e_1x^{\dfrac{1}{2}}.lnx.dx\)
\(\left\{{}\begin{matrix}u=lnx\\dv=x^{\dfrac{1}{2}}\end{matrix}\right.\Rightarrow\left\{{}\begin{matrix}du=\dfrac{dx}{x}\\v=\dfrac{2}{3}.x^{\dfrac{3}{2}}\end{matrix}\right.\)
\(\Rightarrow I=\dfrac{2}{3}.x^{\dfrac{3}{2}}.lnx|^e_1-\dfrac{2}{3}\int\limits^e_1x^{\dfrac{1}{2}}.dx\)
\(=\dfrac{2}{3}.x^{\dfrac{3}{2}}.lnx|^e_1-\dfrac{2}{3}.\dfrac{2}{3}.x^{\dfrac{3}{2}}|^e_1=...\)
19 tháng 8 2023
a: pi/2<x<pi
=>cosx<0
=>\(cosx=-\sqrt{1-\left(\dfrac{1}{5}\right)^2}=-\dfrac{2\sqrt{6}}{5}\)
\(sin2x=2\cdot sinx\cdot cosx=2\cdot\dfrac{1}{5}\cdot\dfrac{-2\sqrt{6}}{5}=\dfrac{-4\sqrt{6}}{25}\)
\(cos2x=2\cdot cos^2x-1=2\cdot\dfrac{24}{25}-1=\dfrac{48}{25}-1=\dfrac{23}{25}\)
\(tan2x=-\dfrac{4\sqrt{6}}{25}:\dfrac{23}{25}=-\dfrac{4\sqrt{6}}{23}\)
\(cot2x=1:\dfrac{-4\sqrt{6}}{23}=\dfrac{-23}{4\sqrt{6}}\)
b: \(sin\left(x-\dfrac{pi}{6}\right)=sinx\cdot cos\left(\dfrac{pi}{6}\right)-cosx\cdot sin\left(\dfrac{pi}{6}\right)\)
\(=sinx\cdot\dfrac{\sqrt{3}}{2}-cosx\cdot\dfrac{1}{2}\)
\(=\dfrac{1}{5}\cdot\dfrac{\sqrt{3}}{2}-\dfrac{-2\sqrt{6}}{5}\cdot\dfrac{1}{2}=\dfrac{\sqrt{3}+2\sqrt{6}}{10}\)
c: \(cos\left(x-\dfrac{pi}{3}\right)=cosx\cdot cos\left(\dfrac{pi}{3}\right)+sinx\cdot sin\left(\dfrac{pi}{3}\right)\)
\(=-\dfrac{2\sqrt{6}}{5}\cdot\dfrac{1}{2}+\dfrac{1}{5}\cdot\dfrac{1}{2}=\dfrac{-2\sqrt{6}+1}{10}\)
d: \(tan\left(x-\dfrac{pi}{4}\right)=\dfrac{tanx-tan\left(\dfrac{pi}{4}\right)}{1+tanx\cdot tan\left(\dfrac{pi}{4}\right)}\)
\(=\dfrac{tanx-1}{1+tanx}\)
\(=\dfrac{\dfrac{1}{-2\sqrt{6}}-1}{1+\dfrac{1}{-2\sqrt{6}}}=\dfrac{-25-4\sqrt{6}}{23}\)
TD
0
19 tháng 8 2023
a: pi<x<3/2pi
=>cosx<0
=>\(cosx=-\sqrt{1-\left(-\dfrac{3}{5}\right)^2}=-\dfrac{4}{5}\)
\(tanx=\dfrac{-3}{5}:\dfrac{-4}{5}=\dfrac{3}{4}\)
cot x=1:3/4=4/3
\(sin2x=2\cdot sinx\cdot cosx=2\cdot\dfrac{-3}{5}\cdot\dfrac{-4}{5}=\dfrac{24}{25}\)
\(cos2x=1-2\cdot sin^2x=1-2\cdot\left(-\dfrac{3}{5}\right)^2=\dfrac{7}{25}\)
\(tan2x=\dfrac{24}{25}:\dfrac{7}{25}=\dfrac{24}{7}\)
cot 2x=1:24/7=7/24
b: \(sin\left(x+\dfrac{pi}{3}\right)=sinx\cdot cos\left(\dfrac{pi}{3}\right)+sin\left(\dfrac{pi}{3}\right)\cdot cosx\)
\(=\dfrac{-3}{5}\cdot\dfrac{1}{2}+\dfrac{\sqrt{3}}{2}\cdot\dfrac{-4}{5}=\dfrac{-3-4\sqrt{3}}{10}\)