3x+2x=x2×23
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1) \(\left(x+1\right)^2=x^2+2x+1\)
2) \(\left(2x+1\right)^2=4x^2+4x+1\)
3) \(\left(2x+y\right)^2=4x^2+4xy+y^2\)
4) \(\left(2x+3\right)^2=4x^2+12x+9\)
5) \(\left(3x+2y\right)^2=9x^2+12xy+4y^2\)
6) \(\left(2x^2+1\right)^2=4x^4+4x^2+1\)
7) \(\left(x^3+1\right)^2=x^6+2x^3+1\)
8) \(\left(x^2+y^3\right)^2=x^4+2x^2y^3+y^6\)
9) \(\left(x^2+2y^2\right)^2=x^4+4x^2y^2+4y^4\)
10) \(\left(\dfrac{1}{2}x+\dfrac{1}{3}y\right)^2=\dfrac{1}{4}x^2+\dfrac{1}{3}xy+\dfrac{1}{9}y^2\)

Bài 1:
a: \(\Leftrightarrow x-1\in\left\{1;-1;3;-3\right\}\)
hay \(x\in\left\{2;0;4;-2\right\}\)

a: Ta có: \(3\left(2x-3\right)+2\left(2-x\right)=-3\)
\(\Leftrightarrow6x-9+4-2x=-3\)
\(\Leftrightarrow4x=2\)
hay \(x=\dfrac{1}{2}\)

\(A=6x^2+23x+21-\left(6x^2+23x-55\right)=76\\ B=x^4+x^3-x^2-2x^2-2x+2-x^4-x^3+3x^2+2x\\ =2\\ C=x^4+x^3-3x^2-2x-\left(x^4+x^3-x^2-2x^2-2x+2\right)\\ =-2\)

Ta có: \(\dfrac{3x+2}{x^2-2x+1}-\dfrac{6}{x^2-1}-\dfrac{3x-2}{x^2+2x+1}\)
\(=\dfrac{3x+2}{\left(x-1\right)^2}-\dfrac{6}{\left(x-1\right)\left(x+1\right)}-\dfrac{3x-2}{\left(x+1\right)^2}\)
\(=\dfrac{\left(3x+2\right)\left(x^2+2x+1\right)}{\left(x-1\right)^2\cdot\left(x+1\right)^2}-\dfrac{6\left(x-1\right)\left(x+1\right)}{\left(x-1\right)^2\cdot\left(x+1\right)^2}-\dfrac{\left(3x-2\right)\left(x^2-2x+1\right)}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)
\(=\dfrac{3x^3+6x^2+3x+2x^2+4x+2-6\left(x^2-1\right)-\left(3x^3-6x^2+3x-2x^2+4x-2\right)}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)
\(=\dfrac{3x^3+8x^2+7x+2-6x^2+6-\left(3x^3-8x^2+7x-2\right)}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)
\(=\dfrac{3x^3+2x^2+7x+8-3x^3+8x^2-7x+2}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)
\(=\dfrac{10x^2+10}{\left(x-1\right)^2\cdot\left(x+1\right)^2}\)


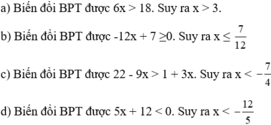
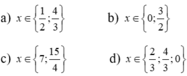
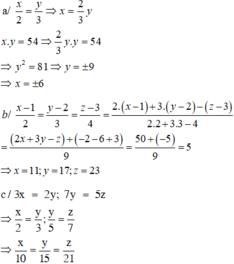
Đề :\(3x+2x=x^2\cdot23\)
\(\Rightarrow3x+2x=23x^2\)
\(\Rightarrow5x=23x^2\)
\(\Rightarrow5x-23x^2=0\)
\(\Rightarrow x\left(5-23x\right)=0\)
Xét 2 TH :
TH1 : \(x=0\)
TH2 : \(5-23x=0\)
\(\Rightarrow23x=5\)
\(\Rightarrow x=\frac{5}{23}\)
Vậy \(x\in\left\{0;\frac{5}{23}\right\}\)
how to??
WTF??