Cho tam giác ABC có trọng tâm G và trung tuyến AM. Khẳng định nào sau đây sai:
A. \(\overrightarrow{GA}\) + 2. \(\overrightarrow{GM}\) = 0
B. \(\overrightarrow{OA}\) + \(\overrightarrow{OB}\) + \(\overrightarrow{OC}\) = 3. \(\overrightarrow{OG}\) , với mọi điểm O
C. \(\overrightarrow{GA}\) + \(\overrightarrow{GB}\) + \(\overrightarrow{GC}\) = 0
D. \(\overrightarrow{AM}\) = -2 . \(\overrightarrow{MG}\)
...
Đọc tiếp
Cho tam giác ABC có trọng tâm G và trung tuyến AM. Khẳng định nào sau đây sai:
A. \(\overrightarrow{GA}\) + 2. \(\overrightarrow{GM}\) = 0
B. \(\overrightarrow{OA}\) + \(\overrightarrow{OB}\) + \(\overrightarrow{OC}\) = 3. \(\overrightarrow{OG}\) , với mọi điểm O
C. \(\overrightarrow{GA}\) + \(\overrightarrow{GB}\) + \(\overrightarrow{GC}\) = 0
D. \(\overrightarrow{AM}\) = -2 . \(\overrightarrow{MG}\)
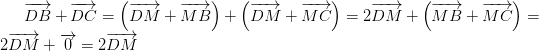
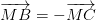
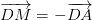
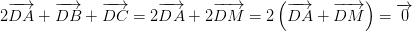
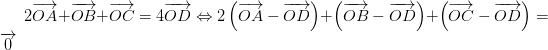
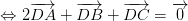luôn đúng theo câu a
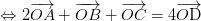, với O là điểm tùy ý
A. Ta có G là trọng tâm của tam giác ABC
\(\Rightarrow\overrightarrow{AG}=2\overrightarrow{GM}\)
Hay: \(\overrightarrow{GA}=-2\overrightarrow{GM}\)
\(\Rightarrow\overrightarrow{GA}+2\overrightarrow{GM}=\overrightarrow{0}\) ( Đúng)
B. Ta có:
\(\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}=\overrightarrow{OG}+\overrightarrow{GA}+\overrightarrow{OG}+\overrightarrow{GB}+\overrightarrow{OG}+\overrightarrow{GC}\)
\(=\left(\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}\right)+3\overrightarrow{OG}=\overrightarrow{0}+3\overrightarrow{OG}=\overrightarrow{3OG}\) ( Đúng)
C. \(\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow{0}\) ( Đúng, đây là điều hiển nhiên)
D. Ta có G là trọng tâm của tam giác ABC
\(\Rightarrow\overrightarrow{AM}=3\overrightarrow{GM}\)
\(\Rightarrow\overrightarrow{AM}=-3\overrightarrow{GM}\)
Vậy đáp án D sai
Câu D sửa lạ là:
⇒ \(\overrightarrow{AM}=-3\overrightarrow{MG}\) ( Nãy vội nên ghi nhầm)