gọi x1,x2 là 2 nghiệm của phương trình \(3x^2+5X-6=0\) không giải phương trình hãy lập phương trình bậc hai ẩn y có 2 nghiệm y1,y2 thỏa mãn y1=2x1-x2 và y2=2x2-x1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
H
9 tháng 4 2023
\(3x^2+5x-6=0\\ \Delta=5^2-4.3.\left(-6\right)=97\\ \Rightarrow\left\{{}\begin{matrix}x_1=\dfrac{-5+\sqrt{97}}{2}\\x_2=\dfrac{-5-\sqrt{97}}{2}\end{matrix}\right.\)
\(\left(x_1-2x_2\right).\left(2x_1-x_2\right)=2x^2_1-4x_1x_2+2x_2^2\)
\(=2.\left(\dfrac{-5+\sqrt{97}}{2}\right)^2-4.\left(\dfrac{-5+\sqrt{97}}{2}\right).\left(\dfrac{-5-\sqrt{97}}{2}\right)+2.\left(\dfrac{-5-\sqrt{97}}{2}\right)^2\\ =\left(\dfrac{-5+\sqrt{97}}{2}\right)^2-2.\left(\dfrac{-5+\sqrt{97}}{2}\right).\left(\dfrac{-5-\sqrt{97}}{2}\right)+\dfrac{\left(-5-\sqrt{97}\right)^2}{2^2}\\ =\left(\dfrac{-5+\sqrt{97}}{2}-\dfrac{-5-\sqrt{97}}{2}\right)^2\\ =\left(\dfrac{-5+\sqrt{97}+5+\sqrt{97}}{2}\right)^2\\ =\left(\dfrac{2\sqrt{97}}{2}\right)^2\\ =\left(\sqrt{97}\right)^2=97\)
30 tháng 5 2021
\(x^2-2x-1=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=2\\x_1x_2=-1\end{matrix}\right.\)
Đặt \(\left\{{}\begin{matrix}u=x_1+\left(x_2\right)^2\\v=x_2+\left(x_1\right)^2\end{matrix}\right.\)
\(\Rightarrow\)\(\left\{{}\begin{matrix}u+v=\left(x_1+x_2\right)+\left(x_2+x_1\right)^2-2x_1x_2\\uv=2x_1x_2+x_1^3+x_2^3=2x_1x_2+\left(x_1+x_2\right)^3-3x_1x_2\left(x_1+x_2\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u+v=8\\uv=12\end{matrix}\right.\)
=>u và v là nghiệm của pt \(t^2-8t+12=0\)
17 tháng 5 2021
a) Ta có: \(\text{Δ}=\left[-2\left(m-1\right)\right]^2-4\cdot1\cdot\left(-m\right)\)
\(=\left(2m-2\right)^2+4m\)
\(=4m^2-8m+4+4m\)
\(=4m^2-4m+4\)
\(=4m^2-4m+1+3\)
\(=\left(2m-1\right)^2+3>0\forall x\)
Do đó: Phương trình luôn có hai nghiệm x1,x2 với mọi m(Đpcm)
17 tháng 5 2021
b) Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)=2m-2\\x_1\cdot x_2=-m\end{matrix}\right.\)
Ta có: \(y_1+y_2=x_1+\dfrac{1}{x_2}+x_2+\dfrac{1}{x_1}\)
\(=\left(x_1+x_2\right)+\left(\dfrac{1}{x_1}+\dfrac{1}{x_2}\right)\)
\(=\left(2m-2\right)+\dfrac{2m-2}{-m}\)
\(=2m-2-\dfrac{2m-2}{m}\)
\(=\dfrac{2m^2-2m-2m+2}{m}\)
\(=\dfrac{2m^2-4m+2}{m}\)
\(=\dfrac{2\left(m^2-2m+1\right)}{m}\)
\(=\dfrac{2\left(m-1\right)^2}{m}\)
Ta có: \(y_1y_2=\left(x_1+\dfrac{1}{x_2}\right)\left(x_2+\dfrac{1}{x_1}\right)\)
\(=x_1x_2+2+\dfrac{1}{x_1x_2}\)
\(=-m+2+\dfrac{1}{-m}\)
\(=-m+2-\dfrac{1}{m}\)
\(=\dfrac{-m^2}{m}+\dfrac{2m}{m}-\dfrac{1}{m}\)
\(=\dfrac{-m^2+2m-1}{m}\)
\(=\dfrac{-\left(m-1\right)^2}{m}\)
Phương trình đó sẽ là:
\(x^2-\dfrac{2\left(m-1\right)^2}{m}x-\dfrac{\left(m-1\right)^2}{m}=0\)
AH
Akai Haruma
Giáo viên
9 tháng 5 2023
Lời giải:
Theo định lý Viet:
$x_1+x_2=2$
$x_1x_2=-6$
Khi đó:
$A=2x_1-x_1x_2+2x_2=2(x_1+x_2)-x_1x_2$
$=2.2-(-6)=4+6=10$
29 tháng 8 2022
\(y_1+y_2=\left(x_1+x_2\right)+\dfrac{x_1+x_2}{x_1x_2}\)
\(=\dfrac{-5}{3}+\dfrac{-5}{3}:\left(-2\right)=\dfrac{-5}{3}+\dfrac{5}{6}=\dfrac{-5}{6}\)
\(y_1y_2=\left(x_1+\dfrac{1}{x_2}\right)\left(x_2+\dfrac{1}{x_1}\right)\)
\(=x_1x_2+2+\dfrac{1}{x_1x_2}=\left(-2\right)+2+\dfrac{1}{\left(-2\right)}=\dfrac{-1}{2}\)
Pt cần tìm có dạng là \(y^2+\dfrac{5}{6}y-\dfrac{1}{2}=0\)
16 tháng 2 2022
bạn đăng tách ra cho mn giúp nhé
a, Để pt có 2 nghiệm pb
\(\Delta'=1-m\ge0\Leftrightarrow m\le1\)
Theo Vi et \(\left\{{}\begin{matrix}x_1+x_2=-2\left(1\right)\\x_1x_2=m\left(2\right)\end{matrix}\right.\)
\(x_1-3x_2=0\)(3)
Từ (1) ; (3) ta có hệ \(\left\{{}\begin{matrix}x_1+x_2=-2\\x_1-3x_2=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x_1=-2\\x_2=-2-x_1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_1=-\dfrac{1}{2}\\x_2=-\dfrac{3}{2}\end{matrix}\right.\)
Thay vào (2) ta được \(m=\left(-\dfrac{1}{2}\right)\left(-\dfrac{3}{2}\right)=\dfrac{3}{4}\)
16 tháng 2 2022
\(b,\Delta=\left(m+5\right)^2-4\left(-m+6\right)\ge0\Leftrightarrow\left[{}\begin{matrix}m\le-7-4\sqrt{3}\\m\ge-7+4\sqrt{3}\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x1+x2=m+5\\2x1+3x2=13\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}2x1+2x2=2m+10\\2x1+3x2=13\end{matrix}\right.\)\(\)
\(\Rightarrow x2=13-2m-10=3-2m\Rightarrow x1=m+5-x2=m+5-3+2m=3m+2\)
\(x1x2=6-m\Rightarrow\left(3-2m\right)\left(3m+2\right)=6-m\Leftrightarrow\left[{}\begin{matrix}m=0\left(tm\right)\\m=1\left(tm\right)\end{matrix}\right.\)
\(c,\Delta'=\left(m+1\right)^2-\left(m^2-2m+29\right)\ge0\Leftrightarrow m\ge7\)
\(\Rightarrow\left\{{}\begin{matrix}x1+x2=2m+2\\x1=2x2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x2=\dfrac{2m+2}{3}\\x1=\dfrac{2\left(2m+2\right)}{3}\end{matrix}\right.\)
\(\Rightarrow x1.x2=\dfrac{\left(2m+2\right).2\left(2m+2\right)}{9}=m^2-2m+29\Leftrightarrow\left[{}\begin{matrix}m=11\left(tm\right)\\m=23\left(tm\right)\end{matrix}\right.\)
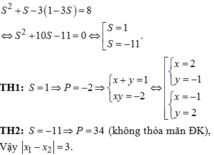
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{5}{3}\\x_1x_2=-2\end{matrix}\right.\)
Ta có: \(\left\{{}\begin{matrix}y_1+y_2=2x_1-x_2+2x_2-x_1\\y_1y_2=\left(2x_1-x_2\right)\left(2x_2-x_1\right)\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y_1+y_2=x_1+x_2\\y_1y_2=-2x_1^2-2x_2^2+5x_1x_2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y_1+y_2=-\dfrac{5}{3}\\y_1y_2=-2\left(x_1+x_2\right)^2+9x_1x_2\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}y_1+y_2=-\dfrac{5}{3}\\y_1y_2=-2.\left(-\dfrac{5}{3}\right)^2+9.\left(-2\right)=-\dfrac{212}{9}\end{matrix}\right.\)
\(\Rightarrow y_1;y_2\) là nghiệm của:
\(y^2+\dfrac{5}{3}y-\dfrac{212}{9}=0\Leftrightarrow9y^2+10y-212=0\)