Rút gọn biểu thức A=\(\dfrac{a^{\dfrac{1}{3}}-a^{\dfrac{7}{3}}}{a^{\dfrac{1}{3}}-a^{\dfrac{4}{3}}}-\dfrac{a^{\dfrac{1}{3}}-a^{\dfrac{5}{3}}}{a^{\dfrac{2}{3}}+a^{\dfrac{1}{3}}}\) ?
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


b, B = \(\dfrac{1}{2}\) - \(\dfrac{1}{2^2}\) + \(\dfrac{1}{2^3}\) - \(\dfrac{1}{2^4}\)+.....+ \(\dfrac{1}{2^{99}}\) - \(\dfrac{1}{2^{100}}\)
2 \(\times\) B = 1 + \(\dfrac{1}{2}\) + \(\dfrac{1}{2^2}\) - \(\dfrac{1}{2^3}\) + \(\dfrac{1}{2^4}\)-.......-\(\dfrac{1}{2^{99}}\)
2 \(\times\) B + B = 1 - \(\dfrac{1}{2^{100}}\)
3B = ( 1 - \(\dfrac{1}{2^{100}}\))
B = ( 1 - \(\dfrac{1}{2^{100}}\)) : 3
A = 1 + \(\dfrac{1}{3}\) + \(\dfrac{1}{3^2}\)+ \(\dfrac{1}{3^3}\)+......+ \(\dfrac{1}{3^{n-1}}\) + \(\dfrac{1}{3^n}\)
A\(\times\) 3 = 3 + 1 + \(\dfrac{1}{3}\) + \(\dfrac{1}{3^2}\) + \(\dfrac{1}{3^2}\)+....+ \(\dfrac{1}{3^{n-1}}\)
A \(\times\) 3 - A = 3 - \(\dfrac{1}{3^n}\)
2A = 3 - \(\dfrac{1}{3^n}\)
A = ( 3 - \(\dfrac{1}{3^n}\)) : 2

Lời giải:
a.
\(=\frac{\sqrt{5}+2}{(\sqrt{5}-2)(\sqrt{5}+2)}+\frac{4(\sqrt{5}-1)}{(\sqrt{5}-1)(\sqrt{5}+1)}=\frac{\sqrt{5}+2}{5-2^2}+\frac{4(\sqrt{5}-1)}{5-1}\)
$=\sqrt{5}+2+(\sqrt{5}-1)=2\sqrt{5}+1$
b.
$=\frac{4(\sqrt{3}+1)}{(\sqrt{3}-1)(\sqrt{3}+1)}+\frac{7(3+\sqrt{2})}{(3-\sqrt{2})(3+\sqrt{2})}-2\sqrt{3}$
$=\frac{4(\sqrt{3}+1)}{2}+\frac{7(3+\sqrt{2})}{1}-2\sqrt{3}$
$=2(\sqrt{3}+1)+7(3+\sqrt{2})-2\sqrt{3}$
$=23+7\sqrt{2}$
c.
$=(\frac{4(3+\sqrt{5})}{(3-\sqrt{5})(3+\sqrt{5})}-\frac{\sqrt{5}+2}{(\sqrt{5}-2)(\sqrt{5}+2)}).\frac{7(3+\sqrt{2})}{(3-\sqrt{2})(3+\sqrt{2})}$
$=[(3+\sqrt{5})-(\sqrt{5}+2)].(3+\sqrt{2})$
$=1(3+\sqrt{2})=3+\sqrt{2}$

a) =
=
b) =
=
=
. ( Với điều kiện b # 1)
c) \(\dfrac{a^{\dfrac{1}{3}}b^{-\dfrac{1}{3}-}a^{-\dfrac{1}{3}}b^{\dfrac{1}{3}}}{\sqrt[3]{a^2}-\sqrt[3]{b^2}}\)= =
=
( với điều kiện a#b).
d) \(\dfrac{a^{\dfrac{1}{3}}\sqrt{b}+b^{\dfrac{1}{3}}\sqrt{a}}{\sqrt[6]{a}+\sqrt[6]{b}}\) = =
=
=

ĐKXĐ: a > 0 và a khác 1
\(P=\dfrac{2\left(a^2+2\right)}{\left(1-a\right)\left(1+a+a^2\right)}-\dfrac{\left(1-\sqrt{a}\right)\left(1+a+a^2\right)}{\left(1-a\right)\left(1+a+a^2\right)}-\dfrac{\left(1+\sqrt{a}\right)\left(1+a+a^2\right)}{\left(1-a\right)\left(1+a+a^2\right)}\)\(=\dfrac{2a^2+4-\left(1+a+a^2\right).\left(1-\sqrt{a}+1+\sqrt{a}\right)}{1-a^3}\)
\(=\dfrac{2a^2+4-\left(1+a+a^2\right)}{1-a^3}\)
\(=\dfrac{a^2+a+3}{\left(1-a^3\right)}\)

Đk:\(a>2\)
\(\left(\dfrac{\sqrt{a-2}+2}{3}\right)\left(\dfrac{\sqrt{a-2}}{3+\sqrt{a-2}}+\dfrac{a+7}{11-a}\right):\left(\dfrac{3\sqrt{a-2}+1}{a-3\sqrt{a-2}-2}-\dfrac{1}{\sqrt{a-2}}\right)\)
Đặt \(b=\sqrt{a-2}\Leftrightarrow a=b^2+2\)
Biểu thức \(\Leftrightarrow\dfrac{b+2}{3}\left(\dfrac{b}{3+b}+\dfrac{b^2+2+7}{11-b^2-2}\right):\left(\dfrac{3b+1}{b^2-3b}-\dfrac{1}{b}\right)\)
\(=\dfrac{b+2}{3}\left[\dfrac{b}{3+b}-\dfrac{b^2+9}{b^2-9}\right]:\left[\dfrac{3b+1}{b\left(b-3\right)}-\dfrac{b-3}{b\left(b-3\right)}\right]\)
\(=\dfrac{b+2}{3}.\dfrac{b\left(b-3\right)-b^2-9}{\left(b-3\right)\left(3+b\right)}:\dfrac{3b+1-\left(b-3\right)}{b\left(b-3\right)}\)
\(=\dfrac{b+2}{3}.\dfrac{-3\left(b+3\right)}{\left(b-3\right)\left(3+b\right)}.\dfrac{b\left(b-3\right)}{2\left(b+2\right)}\)
\(=-\dfrac{b}{2}\)
\(=\dfrac{\sqrt{a-2}}{-2}\)

1,
\(A=\dfrac{4x^2}{\left(x-2\right)\left(x+2\right)}+\dfrac{x-2}{\left(x-2\right)\left(x+2\right)}-\dfrac{x+2}{\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{4x^2+x-2-\left(x+2\right)}{\left(x-2\right)\left(x+2\right)}=\dfrac{4x^2-4}{\left(x-2\right)\left(x+2\right)}\)
\(x=4\Rightarrow A=\dfrac{4.x^2-4}{\left(4-2\right)\left(4+2\right)}=...\)
2.
\(A=\dfrac{x\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}+\dfrac{3\left(x-1\right)}{\left(x-1\right)\left(x+1\right)}+\dfrac{3-5x}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{x\left(x+1\right)+3\left(x-1\right)+3-5x}{\left(x-1\right)\left(x+1\right)}=\dfrac{x^2-2x+1}{\left(x-1\right)\left(x+1\right)}\)
\(=\dfrac{\left(x-1\right)^2}{\left(x-1\right)\left(x+1\right)}=\dfrac{x-1}{x+1}\)
3.
Đề lỗi, thiếu dấu trước \(\dfrac{6+5x}{4-x^2}\)
4.
\(A=\dfrac{2x}{\left(x-5\right)\left(x+5\right)}-\dfrac{5\left(x+5\right)}{\left(x-5\right)\left(x+5\right)}-\dfrac{x-5}{\left(x-5\right)\left(x+5\right)}\)
\(=\dfrac{2x-5\left(x+5\right)-\left(x-5\right)}{\left(x-5\right)\left(x+5\right)}=\dfrac{-4x-20}{\left(x-5\right)\left(x+5\right)}\)
\(=\dfrac{-4\left(x+5\right)}{\left(x-5\right)\left(x+5\right)}=\dfrac{-4}{x-5}\)
\(x=\dfrac{4}{5}\Rightarrow A=\dfrac{-4}{\dfrac{4}{5}-5}=\dfrac{20}{21}\)
5.
\(M=\dfrac{x^2}{x\left(x+2\right)}+\dfrac{2x}{x\left(x+2\right)}+\dfrac{2\left(x+2\right)}{x\left(x+2\right)}\)
\(=\dfrac{x^2+2x+2\left(x+2\right)}{x\left(x+2\right)}=\dfrac{x^2+4x+4}{x\left(x+2\right)}\)
\(=\dfrac{\left(x+2\right)^2}{x\left(x+2\right)}=\dfrac{x+2}{x}\)
\(x=-\dfrac{3}{2}\Rightarrow M=\dfrac{-\dfrac{3}{2}+2}{-\dfrac{3}{2}}=-\dfrac{1}{3}\)
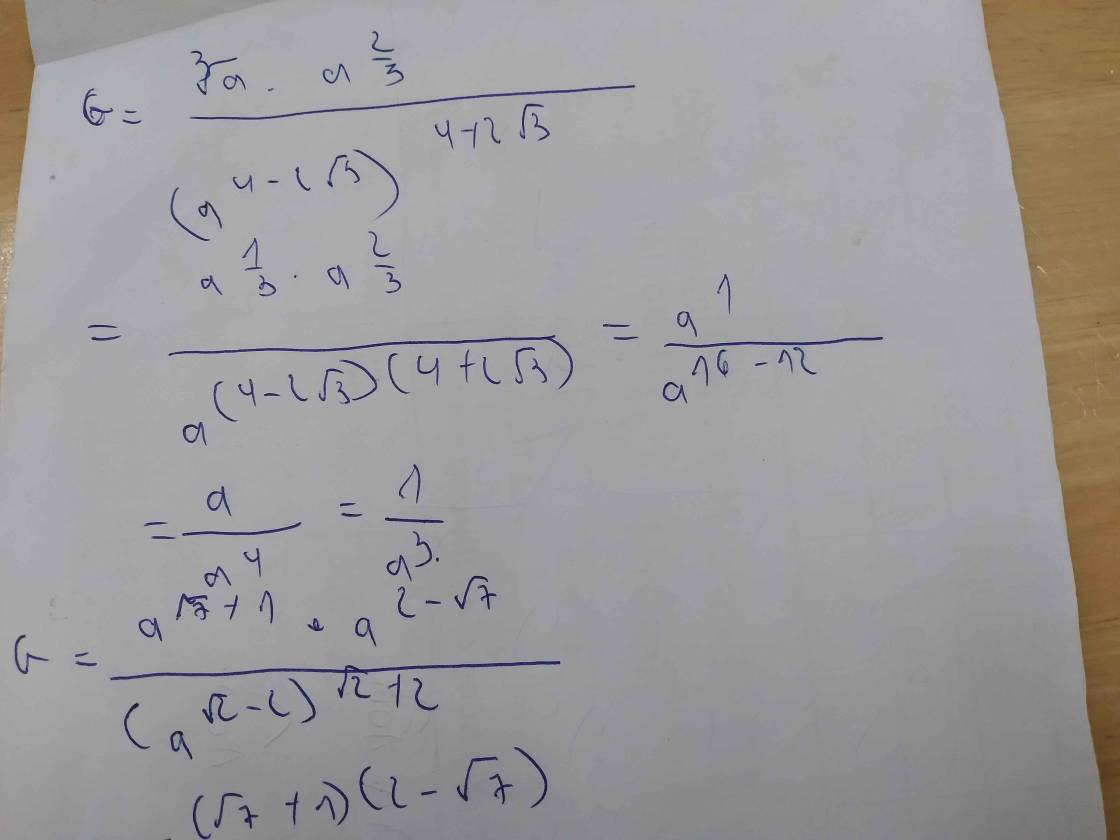

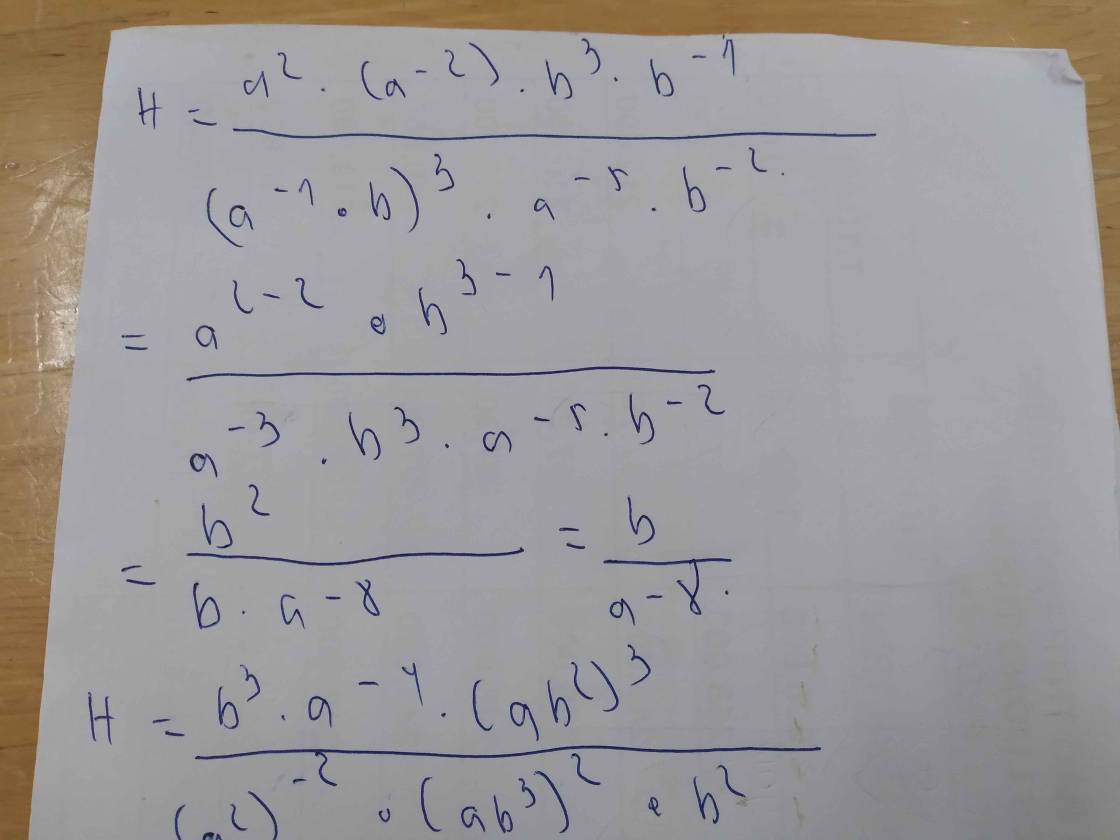
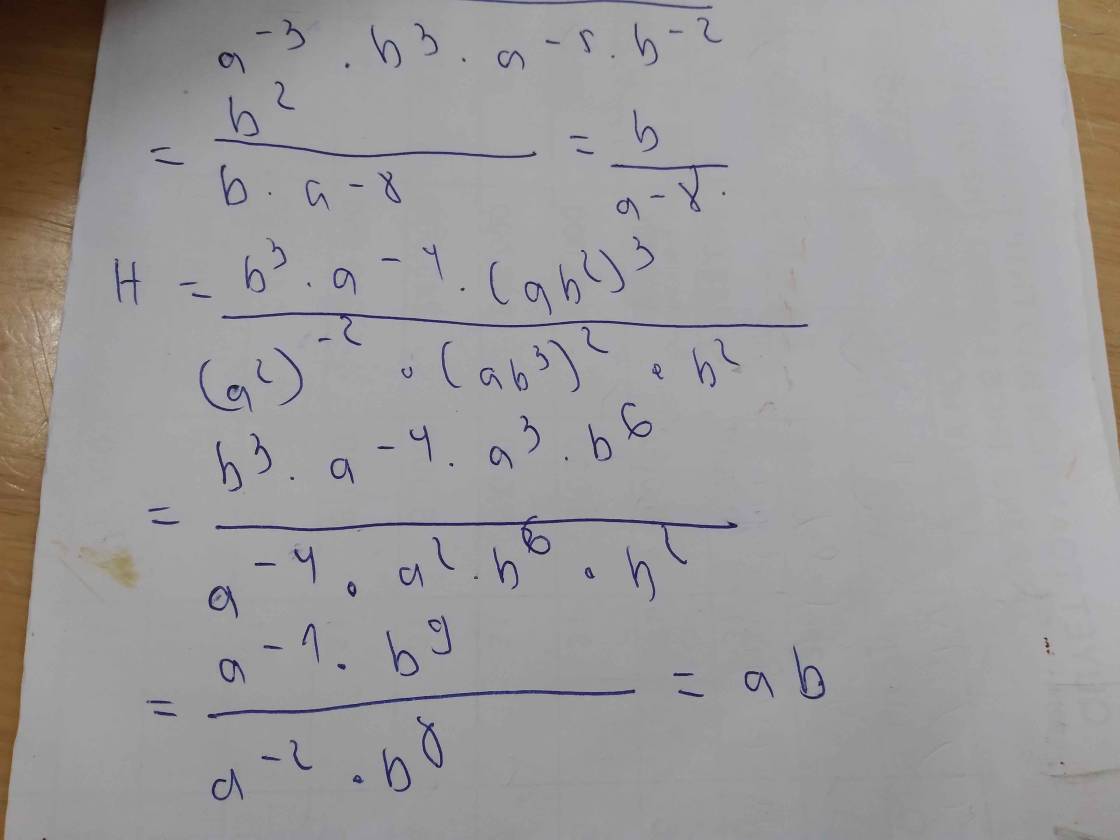
Lời giải:
Ta có \(A=\frac{a^{\frac{1}{3}}-a^{\frac{7}{3}}}{a^{\frac{1}{3}}-a^{\frac{4}{3}}}-\frac{a^{\frac{1}{3}}-a^{\frac{5}{3}}}{a^{\frac{2}{3}}+a^{\frac{1}{3}}}\)
\(=\frac{\sqrt[3]{a}-\sqrt[3]{a^7}}{\sqrt[3]{a}-\sqrt[3]{a^4}}-\frac{\sqrt[3]{a}-\sqrt[3]{a^5}}{\sqrt[3]{a^2}+\sqrt[3]{a}}\)
\(=\frac{\sqrt[3]{a}(1-a^2)}{\sqrt[3]{a}(1-a)}-\frac{\sqrt[3]{a}(1-\sqrt[3]{a^4})}{\sqrt[3]{a}(1+\sqrt[3]{a})}=\frac{1-a^2}{1-a}-\frac{1-\sqrt[3]{a^4}}{1+\sqrt[3]{a}}\)
\(=1+a-\frac{1-\sqrt[3]{a^4}}{1+\sqrt[3]{a}}\)
Đặt \(\sqrt[3]{a}=t\Rightarrow A=1+t^3-\frac{1-t^4}{1+t}=1+t^3-\frac{(1-t^2)(1+t^2)}{1+t}\)
\(=1+t^3-\frac{(1-t)(1+t)(1+t^2)}{1+t}=1+t^3-(1-t)(1+t^2)\)
\(=2t^3-t^2+t\)