Cho hàm số: \(y=-\dfrac{x^3}{3}+\left(a-1\right)x^2+\left(a+3\right)x-4\). Tìm a để hàm số đồng biến trên khoảng (0;3)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
12 tháng 1 2022
\(y'=x^2-2\left(m-1\right)x+3\left(m-1\right)\)
Hàm đồng biến trên khoảng đã cho khi với mọi \(x>1\) ta luôn có:
\(g\left(x\right)=x^2-2\left(m-1\right)x+3\left(m-1\right)\ge0\)
\(\Rightarrow\min\limits_{x>1}g\left(x\right)\ge0\)
Do \(a=1>0;-\dfrac{b}{2a}=m-1\)
TH1: \(m-1\ge1\Rightarrow m\ge2\)
\(\Rightarrow g\left(x\right)_{min}=f\left(m-1\right)=\left(m-1\right)^2-2\left(m-1\right)^2+3\left(m-1\right)\ge0\)
\(\Rightarrow\left(m-1\right)\left(4-m\right)\ge0\Rightarrow1\le m\le4\Rightarrow2\le m\le4\)
TH2: \(m-1< 1\Rightarrow m< 2\Rightarrow g\left(x\right)_{min}=g\left(1\right)=m\ge0\)
Vậy \(0\le m\le4\)
1 tháng 11 2023
y=sin x đồng biến trên \(\left(-\dfrac{\Omega}{2}+k2\Omega;\dfrac{\Omega}{2}+k2\Omega\right)\)
=>Hàm số y=sin x không thể đồng biến trên cả khoảng \(\left(0;\dfrac{5}{6}\Omega\right)\) được
=>Loại A
\(y=cosx\) đồng biến trên khoảng \(\left(-\Omega+k2\Omega;k2\Omega\right)\)
=>Hàm số y=cosx cũng không thể đồng biến trên khoảng \(\left(0;\dfrac{5}{6}\Omega\right)\)
=>Loại B
\(x\in\left(0;\dfrac{5}{6}\Omega\right)\)
=>\(x+\dfrac{\Omega}{3}\in\left(\dfrac{\Omega}{3};\dfrac{4}{3}\Omega\right)\)
=>\(y=sin\left(x+\dfrac{\Omega}{3}\right)\in\left[-\dfrac{\sqrt{3}}{2};\dfrac{\sqrt{3}}{2}\right]\)
=>Khi x tăng thì y chưa chắc tăng
=>Loại D
=>Chọn C
14 tháng 7 2021
Bài 1:
a) Để hàm số y=(k-2)x+k+3 là hàm số bậc nhất thì \(k\ne2\)
b) Để hàm số y=(k-2)x+k+3 đồng biến trên R thì k-2>0
hay k>2
Bài 2:
Thay \(x=-\dfrac{1}{2}\) và \(y=\dfrac{2}{3}\) vào (D), ta được:
\(\left(2m-3\right)\cdot\dfrac{-1}{2}-\dfrac{1}{2}=\dfrac{2}{3}\)
\(\Leftrightarrow\left(2m-3\right)\cdot\dfrac{-1}{2}=\dfrac{2}{3}+\dfrac{1}{2}=\dfrac{7}{6}\)
\(\Leftrightarrow2m-3=\dfrac{7}{6}:\dfrac{-1}{2}=\dfrac{-7}{6}\cdot\dfrac{2}{1}=-\dfrac{14}{6}=-\dfrac{7}{3}\)
\(\Leftrightarrow2m=\dfrac{-7}{3}+3=\dfrac{-7}{3}+\dfrac{9}{3}=\dfrac{2}{3}\)
hay \(m=\dfrac{1}{3}\)
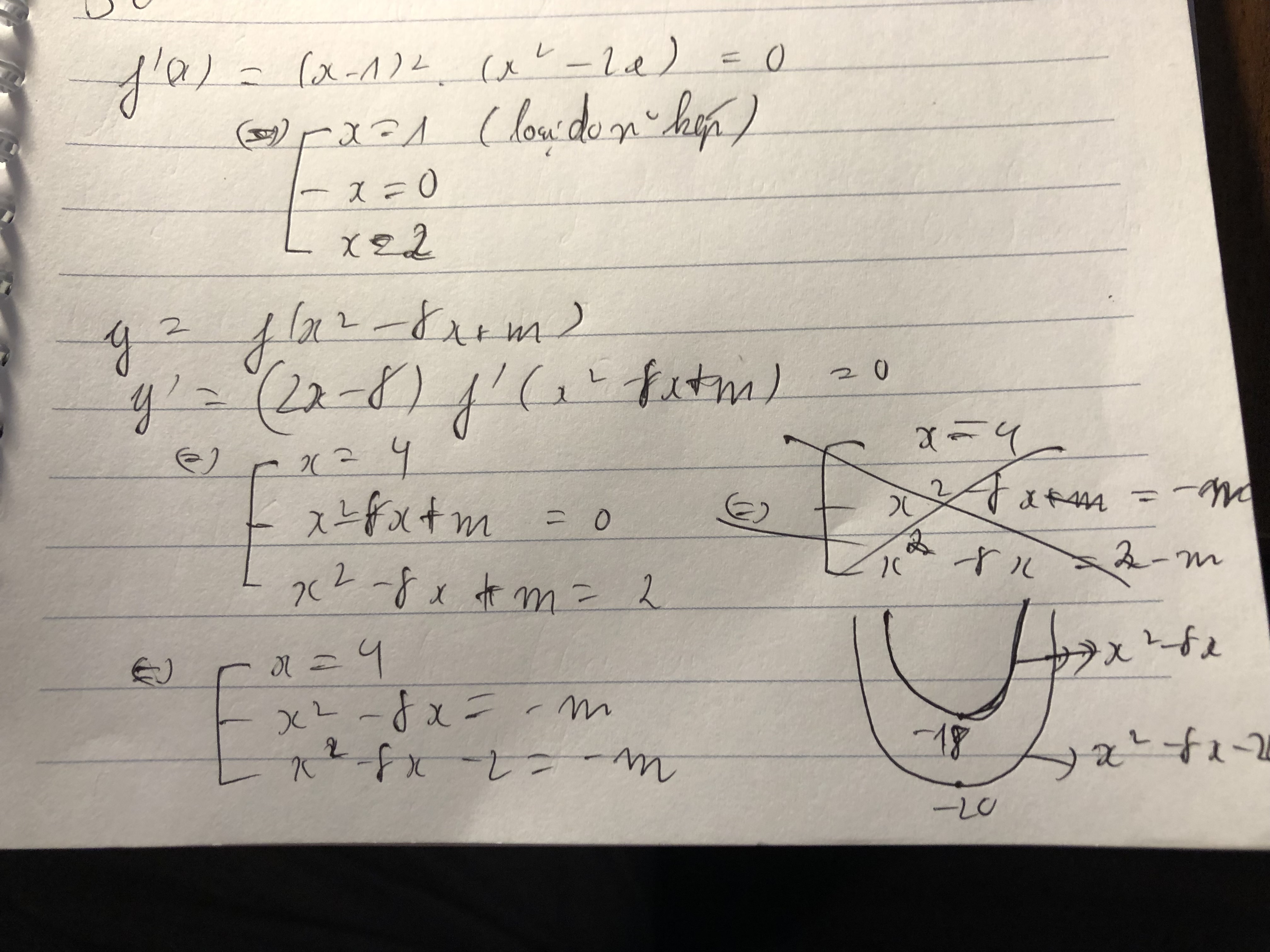
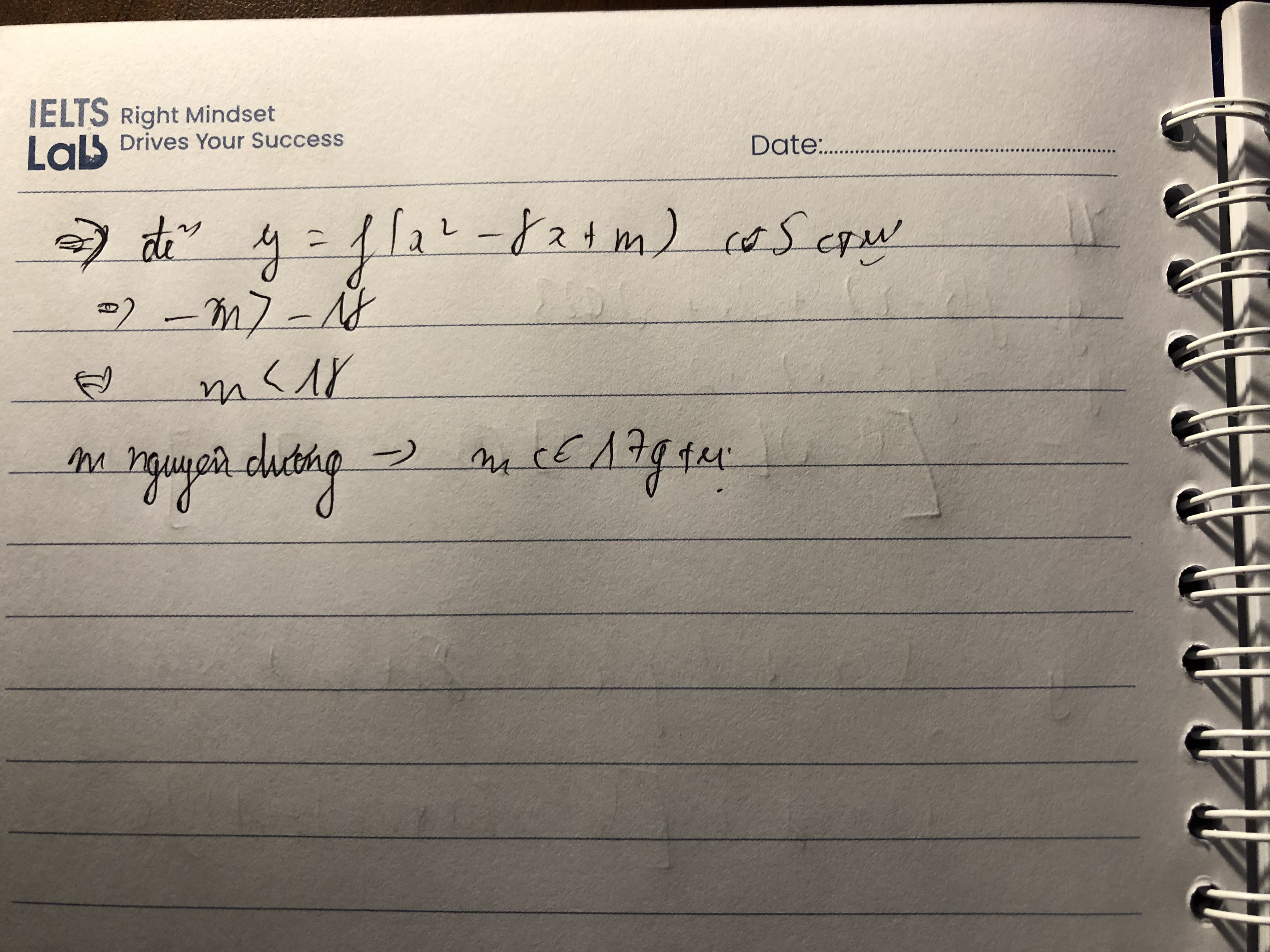
\(y'=-x^2+2\left(a-1\right)x+a+3\)
Hàm đồng biến trên khoảng đã cho khi với mọi \(x\in\left(0;3\right)\) ta có:
\(-x^2+2\left(a-1\right)x+a+3\ge0\)
\(\Leftrightarrow\left(2x+1\right)a\ge x^2+2x-3\)
\(\Rightarrow a\ge\dfrac{x^2+2x-3}{2x+1}\)
Xét hàm \(f\left(x\right)=\dfrac{x^2+2x-3}{2x+1}\) với \(x\in\left(0;3\right)\)
\(f'\left(x\right)=\dfrac{2\left(x^2+x+4\right)}{\left(2x+1\right)^2}>0\Rightarrow f\left(x\right)\) đồng biến
\(\Rightarrow f\left(x\right)< f\left(3\right)=\dfrac{12}{7}\Rightarrow a\ge\dfrac{12}{7}\)