Question

[Nhi Trần]

Giải giúp e vs ạ E cần gấp

Answer 1

[Ngô Thành Chung]

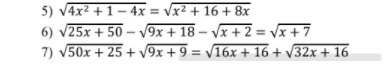
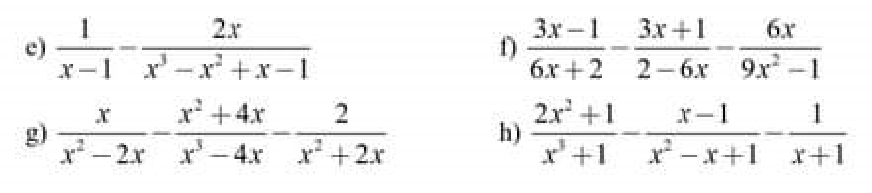
cos2x - (2m + 1)cosx + m + 1 = 0

⇔ 2cos²x - (2m + 1).cosx = 0

⇔ \(\left[{}\begin{matrix}cosx=0\left(1\right)\\2cosx=2m+1\left(2\right)\end{matrix}\right.\)

(1) ⇔ \(x=\dfrac{\pi}{2}+k\pi\) với k thuộc Z. Mà \(x\in\left(\dfrac{\pi}{2};2\pi\right)\)

⇒ x = \(\dfrac{3\pi}{2}\)

Như vậy đã có 1 nghiệm trên \(\left(\dfrac{\pi}{2};2\pi\right)\) đó là x = \(\dfrac{3\pi}{2}\). Bây giờ cần tìm m để (2) có 2 nghiệm phân biệt trên \(\left(\dfrac{\pi}{2};2\pi\right)\) và trong 2 nghiệm đó không có nghiệm x = \(\dfrac{3\pi}{2}\). Tức là x = \(\dfrac{3\pi}{2}\) không thỏa mãn (2), tức là

2m + 1 ≠ 0 ⇔ \(m\ne-\dfrac{1}{2}\)

(2) ⇔ \(2.\left(2cos^2\dfrac{x}{2}-1\right)=2m+1\)

⇔ \(4cos^2\dfrac{x}{2}=2m+3\)

Do x \(\in\left(\dfrac{\pi}{2};2\pi\right)\) nên \(\dfrac{x}{2}\in\left(\dfrac{\pi}{4};\pi\right)\) nên cos\(\dfrac{x}{2}\) ∈ \(\left(-1;\dfrac{\sqrt{2}}{2}\right)\)

Đặt cos\(\dfrac{x}{2}\) = t ⇒ t ∈ \(\left(-1;\dfrac{\sqrt{2}}{2}\right)\). Ta được phương trình : 4t² = 2m + 3

Cần tìm m để [phương trình được bôi đen] có 2 nghiệm t ∈ \(\left(-1;\dfrac{\sqrt{2}}{2}\right)\)

Dùng hàm số bậc 2 là ra. Nhớ kết hợp điều kiện \(m\ne-\dfrac{1}{2}\)