Tìm m để phương trình: \(mx^2-2\left(m+1\right)x+m+5=0\) có 2 nghiệm x1,x2 thỏa mãn x1<0<x2<2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
DH
2 tháng 3 2021
Ta có: \(\Delta=\left(m-4\right)^2-4m.2m=m^2-8m+16-8m^2=-7m^2-8m+16\)
Để phương trình có nghiệm thì \(\Delta>0\Rightarrow\dfrac{-4-8\sqrt{2}}{7}< x< \dfrac{-4+8\sqrt{2}}{7}\)
Áp dụng định lý Vi-et ta có:
\(x_1+x_2=\dfrac{\left(m-4\right)}{m};x_1.x_2=2\) (1)
Mặt khác ta lại có: \(2\left(x_1^2+x_2^2\right)-5x_1x_2=0\\ \Rightarrow2\left(x_1+x_2\right)^2-7x_1x_2=0\)(2)
Thay (1) vào (2) ta được
\(2\left(\dfrac{m-4}{m}\right)^2-7.2=0\)
\(\Rightarrow\left[{}\begin{matrix}m=\dfrac{4}{1-\sqrt{7}}\\m=\dfrac{4}{1+\sqrt{7}}\end{matrix}\right.\) (Loại)
Do đó không có giá trị m thỏa mãn
HP
12 tháng 8 2021
b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)
16 tháng 2 2021
a, Ta có : \(mx^3-x^2+2x-8m=0\)
\(\Leftrightarrow m\left(x^3-8\right)-\left(x^2-2x\right)=0\)
\(\Leftrightarrow m\left(x-2\right)\left(x^2+2x+4\right)-x\left(x-2\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(mx^2+2mx+4m-x\right)=0\)
\(\Leftrightarrow\left(x-2\right)\left(mx^2+x\left(2m-1\right)+4m\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-2=0\\mx^2+x\left(2m-1\right)+4m=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\left(TM\right)\\mx^2+x\left(2m-1\right)+4m=0\left(I\right)\end{matrix}\right.\)
- Để phương trình ban đầu có 3 nghiệm phân biệt lớn hơn 1
<=> Phương trình ( I ) có 2 nghiệm phân biệt lớn hơn 1 .
- Xét phương trình ( I ) có : \(\Delta=b^2-4ac=\left(2m-1\right)^2-4m.4m\)
\(=4m^2-4m+1-16m^2=-12m^2-4m+1\)
- Để phương trình ( I ) có 2 nghiệm phân biệt \(\Leftrightarrow\Delta>0\)
\(\Leftrightarrow-\dfrac{1}{2}< m< \dfrac{1}{6}\) ( * )
- Theo vi ét : \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{1-2m}{m}\\x_1x_2=4\end{matrix}\right.\)
- Để phương trình ( I ) có nghiệm lớn hơn 1 \(\Leftrightarrow\left\{{}\begin{matrix}x_1-1+x_2-1>0\\\left(x_1-1\right)\left(x_2-1\right)>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{1-4m}{m}>0\\5-\dfrac{1-2m}{m}>0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\dfrac{1-4m}{m}>0\\\dfrac{7m-1}{m}>0\end{matrix}\right.\)
- Lập bảng xét dấu ( đoạn này làm tắt tí nha :vv )
Từ bảng xét dấu ta được : \(\left\{{}\begin{matrix}\left[{}\begin{matrix}m< 0\\m>\dfrac{1}{7}\end{matrix}\right.\\0< m< \dfrac{1}{4}\end{matrix}\right.\)
- Kết hợp điều kiện ( * ) ta được :\(\dfrac{1}{7}< m< \dfrac{1}{6}\)
Vậy ...
16 tháng 2 2021
b, - Xét phương trình trên có : \(\Delta^,=b^{,2}-ac=\left(m-2\right)^2-\left(m-1\right)\left(m-3\right)\)
\(=m^2-4m+4-m^2+m+3m-3=1>0\)
Nên phương trình có 2 nghiệm phân biệt .
Theo vi ét : \(\left\{{}\begin{matrix}x_1+x_2=\dfrac{2\left(m-2\right)}{m-1}\\x_1x_2=\dfrac{m-3}{m-1}\end{matrix}\right.\)
- Để \(x_1+x_2+x_1x_2< 1\)
\(\Leftrightarrow\dfrac{2\left(m-2\right)+\left(m-3\right)-\left(m-1\right)}{m-1}< 0\)
\(\Leftrightarrow\dfrac{2m-6}{m-1}< 0\)
- Đặt \(\dfrac{2m-6}{m-1}=f\left(m\right)\)
Cho f(m) = 0 => m = 3
m-1 = 0 => m = 1
- Lập bảng xét dầu :
m.............................1..........................................3...................................
2m-6............-..........|......................-.....................0...................+.................
m-1..............-............0...................+.....................|....................+.................
f(m).............+...........||..................-........................0................+....................
- Từ bảng xét dầu ta được : Để \(f\left(m\right)< 0\)
\(\Leftrightarrow1< m< 3\)
Vậy ...
NV
Nguyễn Việt Lâm
Giáo viên
11 tháng 4 2022
\(ac=-2< 0\Rightarrow\) phương trình luôn có 2 nghiệm pb trái dấu
Mà \(x_1>x_2\Rightarrow\left\{{}\begin{matrix}x_2< 0\\x_1>0\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}\left|x_1\right|=x_1\\\left|x_2\right|=-x_2\end{matrix}\right.\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=m-1\\x_1x_2=-2\end{matrix}\right.\)
\(\left|2x_1\right|-\left|x_2\right|=2+x_1\)
\(\Leftrightarrow2x_1+x_2=2+x_1\)
\(\Leftrightarrow x_1+x_2=2\)
\(\Leftrightarrow m-1=2\)
\(\Rightarrow m=3\)
10 tháng 3 2021
Ta có: \(\Delta\) = m2 - 4(m - 1) = m2 - 4m + 4 = (m - 2)2 \(\ge\) 0
\(\Rightarrow\) x1 = \(\dfrac{m-\left(m-2\right)}{2}=1\); x2 = \(\dfrac{m+m-2}{2}=m-1\)
Ta có: |x1| + |x2| = 4
\(\Leftrightarrow\) 1 + |m - 1| = 4
\(\Leftrightarrow\) |m - 1| = 3
\(\Leftrightarrow\) \(\left[{}\begin{matrix}m-1=3\\m-1=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}m=4\\m=-2\end{matrix}\right.\)
Vậy ...
Chúc bn học tốt!
H
10 tháng 4 2023
a, Thay \(m=-3\) vào \(\left(1\right)\)
\(x^2-2.\left(m-1\right)x-m-3=0\\ \Leftrightarrow x^2-2.\left(-3-1\right)x+3-3=0\\ \Leftrightarrow x^2+8x=0\\ \Leftrightarrow x\left(x+8\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x=-8\end{matrix}\right.\)
Vậy với \(m=-3\) thì \(x=0;x=-8\)
b,
\(\Delta'=\left[-\left(m-1\right)\right]^2-1.\left(-m-3\right)\\ =m^2-2m+1+m+3\\ =m^2-m+4\)
phương trình có hai nghiệm phân biệt
\(\Delta'>0\\ m^2-m+4>0\\ \Rightarrow m^2-2.\dfrac{1}{2}m+\dfrac{1}{4}+\dfrac{7}{2}>0\\ \Leftrightarrow\left(m-\dfrac{1}{2}\right)^2+\dfrac{7}{2}>0\left(lđ\right)\)
\(\Rightarrow\forall m\)
Áp dụng hệ thức Vi ét :
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=-m-3\end{matrix}\right.\)
\(\left(x_1-x_2\right)^2=4m^2-5\left(x_1+x_2\right)\\ \Leftrightarrow x_1^2+2x_1.x_2+x^2_2-4x_1x_2=4m^2-5\left(x_1+x_2\right)\\ \Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2=4m^2-5\left(x_1+x_2\right)\\ \Leftrightarrow\left(2.\left(m-1\right)\right)^2-4.\left(-m-3\right)=4m^2-5.\left(-m-3\right)\\ \Leftrightarrow4m^2-8m+4+4m+12-4m^2-5m-15=0\\ \Leftrightarrow-9m+1=0\\ \Leftrightarrow m=\dfrac{1}{9}\)
Vậy \(m=\dfrac{1}{9}\)
DT
10 tháng 4 2023
a.
Thế m = -3 vào phương trình (1) ta được:
\(x^2-2\left(-3-1\right)x-\left(-3\right)-3=0\)
\(\Leftrightarrow\) \(x^2+8x=0\)
\(\Leftrightarrow x\left(x+8\right)=0\\ \Rightarrow x_1=0,x_2=-8\)
b.
Để phương trình (1) có hai nghiệm phân biệt thì:
\(\Delta>0\\ \Leftrightarrow\left[-2\left(m-1\right)\right]^2-4.1.\left(-m-3\right)>0\)
\(\Leftrightarrow4.\left(m^2-2m+1\right)+4m+12>0\)
\(\Leftrightarrow4m^2-8m+4+4m+12>0\)
\(\Leftrightarrow4m^2-4m+16>0\)
\(\Leftrightarrow\left(2m\right)^2-4m+1+15>0\)
\(\Leftrightarrow\left(2m-1\right)^2+15>0\)
Vì \(\left(2m-1\right)^2\) luôn lớn hơn hoặc bằng 0 với mọi m nên phương trình (1) có nghiệm với mọi m.
Theo viét:
\(\left\{{}\begin{matrix}x_1+x_2=2m-2\\x_1x_2=-m-3\end{matrix}\right.\) (I)
có:
\(\left(x_1-x_2\right)^2=4m^2-5x_1+x_2\)
<=> \(x_1^2-2x_1x_2+x_2^2-4m^2+5x_1-x_2=0\)
<=> \(x_1^2-2x_1x_2+x_2^2+2x_1x_2-2x_1x_2-4m^2+5x_1-x_2=0\)
<=> \(\left(x_1+x_2\right)^2-4x_1x_2-4m^2+5x_1-x_2=0\)
<=> \(\left(2m-2\right)^2-4.\left(-m-3\right)-4m^2+5x_1-x_2=0\)
<=> \(4m^2-8m+4+4m+12-4m^2+5x_1-x_2=0\)
<=> \(-4m+16+5x_1-x_2=0\)
<=> \(5x_1-x_2=4m-16\) (II)
Từ (I) và (II) ta có:
\(\left\{{}\begin{matrix}5x_1-x_2=4m-16\left(2\right)\\x_1+x_2=2m-2\left(3\right)\\x_1x_2=-m-3\left(4\right)\end{matrix}\right.\)
Từ (2) ta có:
\(x_1=\dfrac{4m-16+x_2}{5}=\dfrac{4}{5}m-3,2+\dfrac{1}{5}x_2\) (x)
Thế (x) vào (3) được:
\(\dfrac{4}{5}m-3,2+\dfrac{1}{5}x_2+x_2=2m-2\)
<=> \(\dfrac{4}{5}m-3,2+\dfrac{1}{5}x_2+x_2-2m+2=0\)
<=> \(-1,2m-1,2+1,2x_2=0\)
<=> \(x_2=1,2m+1,2\) (xx)
Thế (xx) vào (3) được:
\(x_1+1,2m+1,2=2m-2\)
<=> \(x_1+1,2m+1,2-2m+2=0\)
<=> \(x_1-0,8m+3,2=0\)
<=> \(x_1=-3,2+0,8m\) (xxx)
Thế (xx) và (xxx) vào (4) được:
\(\left(-3,2+0,8m\right)\left(1,2m+1,2\right)=-m-3\)
<=> \(-3,84m-3,84+0,96m^2+0,96m+m+3=0\)
<=> \(0,96m^2-1,88m-0,84=0\)
\(\Delta=\left(-1,88\right)^2-4.0,96.\left(-0,84\right)=6,76\)
\(m_1=\dfrac{1,88+\sqrt{6,76}}{2.0,96}=\dfrac{7}{3}\left(nhận\right)\)
\(m_2=\dfrac{1,88-\sqrt{6,76}}{2.0,96}=-\dfrac{3}{8}\left(nhận\right)\)
T.Lam
24 tháng 1 2022
b: \(\text{Δ}=\left(2m-2\right)^2-4\left(2m-5\right)\)
\(=4m^2-8m+4-8m+20\)
\(=4m^2-16m+24\)
\(=4\left(m^2-4m+6\right)>0\)
Do đó: Phương trình luôn có hai nghiệm phân biệt
Áp dụng hệ thức Vi-et, ta được:
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=2m-5\end{matrix}\right.\)
Theo đề, ta có: \(\left(\sqrt{x_1}-\sqrt{x_2}\right)^2=4\)
\(\Leftrightarrow x_1+x_2-2\sqrt{x_1x_2}=4\)
\(\Leftrightarrow2m-2-2\sqrt{2m-5}=4\)
\(\Leftrightarrow2\sqrt{2m-5}=2m-6\)
\(\Leftrightarrow\sqrt{2m-5}=m-3\)
\(\Leftrightarrow\left\{{}\begin{matrix}m>=3\\m^2-6m+9-2m+5=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m>=3\\m^2-8m+14=0\end{matrix}\right.\)
Đến đây thì dễ rồi, bạn chỉ cần giải pt bậc hai rồi đối chiếu với đk là xong
AD
6 tháng 6 2023
\(\Delta=\left(-m\right)^2-2.1.\left(m-1\right)\\ =m^2-2m+1\\ =\left(m-1\right)^2\)
Phương trình có hai nghiệm phân biệt :
\(\Leftrightarrow\Delta>0\\ \Rightarrow\left(m-1\right)^2>0\\ \Rightarrow m\ne1\)
Theo vi ét :
\(\Leftrightarrow\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=m-1\end{matrix}\right.\)
\(x^2_1+x^2_2=x_1+x_2\\ \Leftrightarrow x^2_1+x^2_2=m\\ \Leftrightarrow\left(x^2_1+2x_1x_2+x_2^2\right)-2x_1x_2=m\\ \Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2-m=0\\ \Leftrightarrow m^2-2\left(m-1\right)-m=0\\ \Leftrightarrow m^2-2m+2-m=0\\ \Leftrightarrow m^2-3m+2=0\\ \Leftrightarrow\left[{}\begin{matrix}m=1\left(loại\right)\\m=2\left(t/m\right)\end{matrix}\right.\)
Vậy \(m=2\)
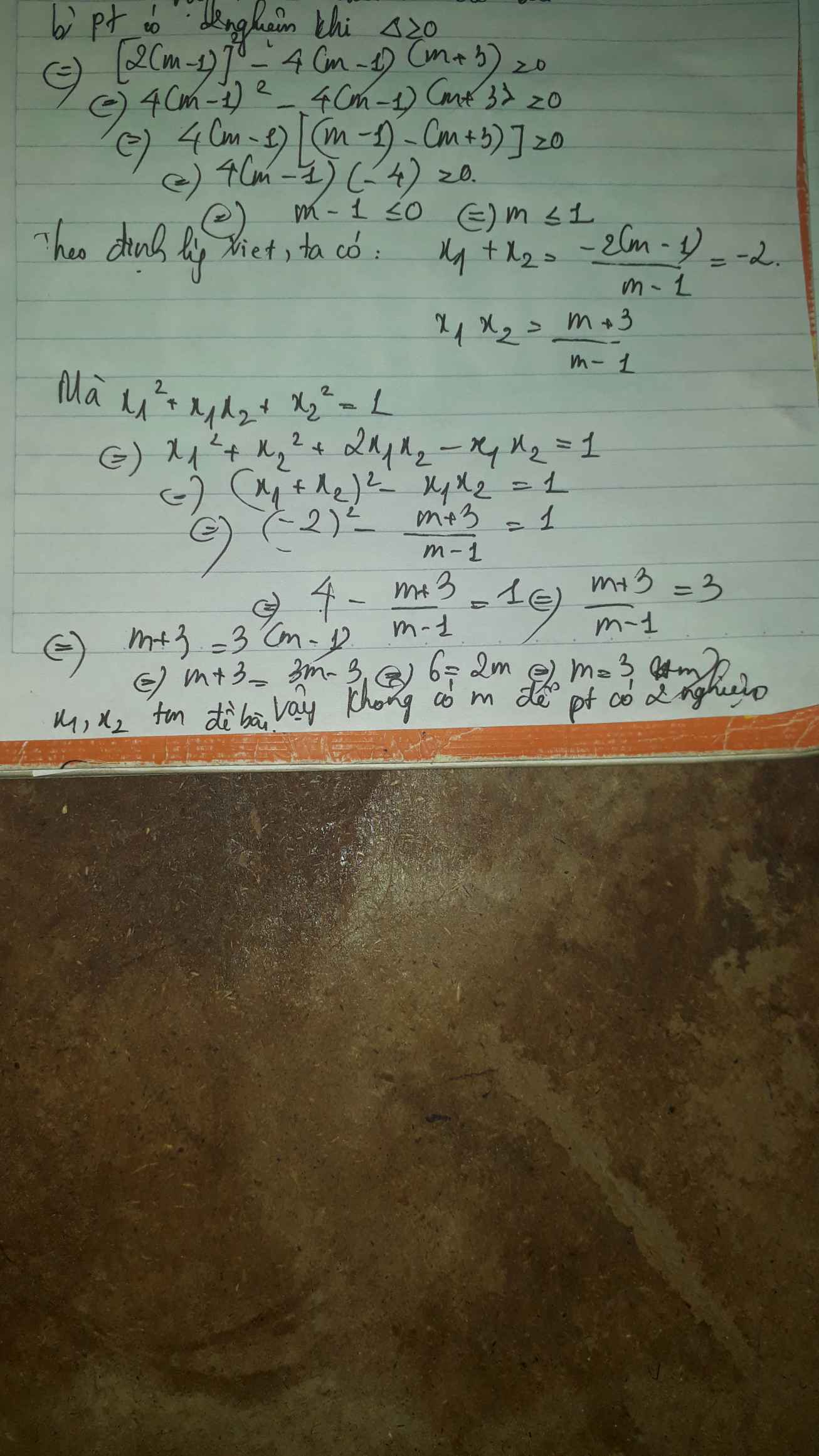
Lời giải:
Để pt có 2 nghiệm thì:
\(\left\{\begin{matrix} m\neq 0\\ \Delta'=(m+1)^2-m(m+5)=1-3m\geq 0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} m\neq 0\\ m\leq\frac{1}{3}\end{matrix}\right.(1)\)
Áp dụng định lý Viet:
\(\left\{\begin{matrix} x_1+x_2=\frac{2(m+1)}{m}\\ x_1x_2=\frac{m+5}{m}\end{matrix}\right.\)
Để $x_1< 0< x_2$
$\Leftrightarrow x_1x_2< 0$
$\Leftrightarrow \frac{m+5}{m}< 0$
$\Leftrightarrow -5< m< 0(2)$
$x_1< x_2< 2$
\(\Leftrightarrow \left\{\begin{matrix} (x_1-2)(x_2-2)>0\\ x_1+x_2<4\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x_1x_2-2(x_1+x_2)+4>0\\ x_1+x_2<4\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} \frac{m+1}{m}>0\\ \frac{1-m}{m}< 0\end{matrix}\right.\Leftrightarrow \left[\begin{matrix} m>1\\ m< -1\end{matrix}\right.(3)\)
Từ $(1);(2);(3)$ suy ra $-5< m< -1$