(1-2x)^2+(2-4x)*(1+2x)+(1+2x)^2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
22 tháng 2 2020
ĐKXĐ : \(x\ne\pm\frac{1}{2}\)
\(E=\left(\frac{\left(4x^2+2x\right)\left(1+4x^2\right)}{\left(1-4x^2\right)\left(1+4x^2\right)}-\frac{\left(4x^2-2x\right)\left(1-4x^2\right)}{\left(1-4x^2\right)\left(1+4x^2\right)}\right):\left(\frac{\left(1+2x\right)\left(1+2x\right)}{\left(1-2x\right)\left(1+2x\right)}-\frac{\left(1-2x\right)\left(1-2x\right)}{\left(1+2x\right)\left(1-2x\right)}\right)\)
\(E=\left(\frac{16x^4+8x^3+4x^2+2x+16x^4-8x^3-4x^2+2x}{1-16x^4}\right):\left(\frac{1+2x+x^2-1+2x-x^2}{1-4x^2}\right)\)
\(E=\frac{32x^4+4x}{1-16x^4}:\frac{4x}{1-4x^2}\)
\(E=\frac{4x\left(8x^3+1\right)}{\left(1-4x^2\right)\left(1+4x^2\right)}.\frac{1-4x^2}{4x}\)
\(E=\frac{8x^3+1}{1+4x^2}\)
Study well
LT
22 tháng 2 2020
E=\(\left(\frac{4x^2+2x}{1-4x^2}-\frac{4x^2-2x}{1+4x^2}\right):\left(\frac{1+2x}{1-2x}-\frac{1-2x}{1+2x}\right)\)
E=\(\left(\frac{\left(4x^2+2x\right)\left(1+4x^2\right)-\left(4x^2-2x\right)\left(1-4x^2\right)}{\left(1-4x^2\right)\left(1+4x^2\right)}\right):\)\(\left(\frac{\left(1+2x\right)^2-\left(1-2x\right)^2}{\left(1-2x\right)\left(1+2x\right)}\right)\)
E=\(\frac{4x^2+16x^4+2x+8x^3-\left(4x^2-16x^4-2x+8x^3\right)}{\left(1-4x^2\right)\left(1+4x^2\right)}:\)\(\left(\frac{\left(1+4x+4x^2\right)-\left(1-4x+4x^2\right)}{\left(1-2x\right)\left(1+2x\right)}\right)\)
E=\(\frac{4x^2+16x^4+2x+8x^3-4x^2+16x^4+2x-8x^3}{\left(1-4x^2\right)\left(1+4x^2\right)}:\)\(\left(\frac{1+4x+4x^2-1+4x-4x^2}{\left(1-2x\right)\left(1+2x\right)}\right)\)
E=\(\frac{16x^4+2x+16x^4+2x}{\left(1-4x^2\right)\left(1+4x^2\right)}:\)\(\left(\frac{8x}{\left(1-2x\right)\left(1+2x\right)}\right)\)
E=\(\frac{32x^4+8x}{\left(1-4x^2\right)\left(1+4x^2\right)}.\frac{1-4x^2}{8x}\)
E=\(\frac{8x\left(4x^3+1\right)}{\left(1-4x^2\right)\left(1+4x^2\right)}.\frac{1-4x^2}{8x}\)
E=\(\frac{4x^3+1}{1+4x^2}\)
16 tháng 6 2023
`@` `\text {Ans}`
`\downarrow`
`a)`
`3x(4x-1) - 2x(6x-3) = 30`
`=> 12x^2 - 3x - 12x^2 + 6x = 30`
`=> 3x = 30`
`=> x = 30 \div 3`
`=> x=10`
Vậy, `x=10`
`b)`
`2x(3-2x) + 2x(2x-1) = 15`
`=> 6x- 4x^2 + 4x^2 - 2x = 15`
`=> 4x = 15`
`=> x = 15/4`
Vậy, `x=15/4`
`c)`
`(5x-2)(4x-1) + (10x+3)(2x-1) = 1`
`=> 5x(4x-1) - 2(4x-1) + 10x(2x-1) + 3(2x-1)=1`
`=> 20x^2-5x - 8x + 2 + 20x^2 - 10x +6x - 3 =1`
`=> 40x^2 -17x - 1 = 1`
`d)`
`(x+2)(x+2)-(x-3)(x+1)=9`
`=> x^2 + 2x + 2x + 4 - x^2 - x + 3x + 3=9`
`=> 6x + 7 =9`
`=> 6x = 2`
`=> x=2/6 =1/3`
Vậy, `x=1/3`
`e)`
`(4x+1)(6x-3) = 7 + (3x-2)(8x+9)`
`=> 24x^2 - 12x + 6x - 3 = 7 + (3x-2)(8x+9)`
`=> 24x^2 - 12x + 6x - 3 = 7 + 24x^2 +11x - 18`
`=> 24x^2 - 6x - 3 = 24x^2 + 18x -11`
`=> 24x^2 - 6x - 3 - 24x^2 + 18x + 11 = 0`
`=> 12x +8 = 0`
`=> 12x = -8`
`=> x= -8/12 = -2/3`
Vậy, `x=-2/3`
`g)`
`(10x+2)(4x- 1)- (8x -3)(5x+2) =14`
`=> 40x^2 - 10x + 8x - 2 - 40x^2 - 16x + 15x + 6 = 14`
`=> -3x + 4 =14`
`=> -3x = 10`
`=> x= - 10/3`
Vậy, `x=-10/3`
22 tháng 2 2020
E=\(\frac{\left(4x^2+2x\right)\left(1+4x^2\right)-\left(4x^2-2x\right)\left(1-4x^2\right)}{\left(1-4x^2\right)\left(1+4x^2\right)}:\frac{\left(1+2x\right)^2-\left(1-2x\right)^2}{1-4x^2}\)
E=\(\frac{4x^2+16x^4+2x+8x^3-4x^2+16x^2+2x-8x^3}{\left(1-4x^2\right)\left(1+4x^2\right)}.\frac{1-4x^2}{1+4x+4x^2-1+4x-4x^2}\)
E=\(\frac{32x^4+4x}{8x\left(1+4x^2\right)}=\frac{8x^3+1}{2\left(1+4x^2\right)}\)
H9
HT.Phong (9A5)
CTVHS
31 tháng 7 2023
p) \(\left(9-x\right)\left(x^2+2x-3\right)\)
\(=9\left(x^2+2x-3\right)-x\left(x^2+2x-3\right)\)
\(=9x^2+18x-27-x^3-2x^2+3x\)
\(=-x^3+7x^2+21x-27\)
n) \(\left(-x+3\right)\left(x^2+x+1\right)\)
\(=-x\left(x^2+x+1\right)+3\left(x^2+x+1\right)\)
\(=-x^3-x^2-x+3x^2+3x+3\)
\(=-x^2+2x^2+2x+3\)
o) \(\left(-6x+\dfrac{1}{2}\right)\left(x^2-4x+2\right)\)
\(=-6x\left(x^2-4x+2\right)+\dfrac{1}{2}\left(x^2-4x+2\right)\)
\(=-6x^3+24x^2-12x+\dfrac{1}{2}x^2-2x+1\)
\(=-6x^3+\dfrac{49}{2}x^2-14x+1\)
q) \(\left(6x+1\right)\left(x^2-2x-3\right)\)
\(=6x\left(x^2-2x-3\right)+\left(x^2-2x-3\right)\)
\(=6x^3-12x^2-18x+x^2-2x-3\)
\(=6x^3-11x^2-20x-3\)
r) \(\left(2x+1\right)\left(-x^2-3x+1\right)\)
\(=2x\left(-x^2-3x+1\right)+\left(-x^2-3x+1\right)\)
\(=-2x^3-6x^2+2x-x^2-3x+1\)
\(=-2x^3-7x^2-x+1\)
u) \(\left(2x-3\right)\left(-x^2+x+6\right)\)
\(=2x\left(-x^2+x+6\right)-3\left(-x^2+x+6\right)\)
\(=-2x^3+2x^2+12x+3x^2-3x-18\)
\(=-2x^3+5x^2+9x-18\)
s) \(\left(-4x+5\right)\left(x^2+3x-2\right)\)
\(=-4x\left(x^2+3x-2\right)+5\left(x^2+3x-2\right)\)
\(=-4x^3-12x^2+8x+5x^2+15x-10\)
\(=-4x^3-7x^2+23x-10\)
v) \(\left(-\dfrac{1}{2}x+3\right)\left(2x+6-4x^3\right)\)
\(=-\dfrac{1}{2}x\left(2x+6-4x^3\right)+3\left(2x+6-4x^3\right)\)
\(=-x^2-3+2x^4+6x+18-12x^3\)
\(=2x^4-12x^3-x^2+6x+15\)
30 tháng 7 2023
p: (-x+9)(x^2+2x-3)
=-x^3-2x^2+3x+9x^2+18x-27
=-x^3+7x^2+21x-27
n: (-x+3)(x^2+x+1)
=-x^3-x^2-x+3x^2+3x+3
=-x^3+2x^2+2x+3
o: (-6x+1/2)(x^2-4x+2)
=-6x^3+24x^2-12x+1/2x^2-2x+1
=-64x^3+49/2x^2-14x+1
q: (6x+1)(x^2-2x-3)
=6x^3-12x^2-18x+x^2-2x-3
=6x^3-11x^2-20x-3
r: (2x+1)(-x^2-3x+1)
=-2x^3-6x^2+2x-x^2-3x+1
=-2x^3-7x^2-x+1
u: =-2x^3+2x^2+12x+3x^2-3x-18
=-2x^3+5x^2+9x-18
s: =-4x^3-12x^2+8x+5x^2+15x-10
=-4x^3-7x^2+23x-10
26 tháng 6 2021
a,sửa đề : \(\left(\frac{1}{x^2+4x+4}-\frac{1}{x^2-4x+4}\right):\left(\frac{1}{x+2}+\frac{1}{x^2-4}\right)\)
\(=\left(\frac{1}{\left(x+2\right)^2}-\frac{1}{\left(x-2\right)^2}\right):\left(\frac{x-2+1}{\left(x+2\right)\left(x-2\right)}\right)\)
\(=\left(\frac{x^2-4x+4-x^2-4x-4}{\left(x+2\right)^2\left(x-2\right)^2}\right):\left(\frac{x-1}{\left(x+2\right)\left(x-2\right)}\right)\)
\(=\frac{-8x\left(x+2\right)\left(x-2\right)}{\left(x+2\right)^2\left(x-2\right)^2\left(x-1\right)}=\frac{-8x}{\left(x-1\right)\left(x^2-4\right)}\)
26 tháng 6 2021
b, \(\left(\frac{2x}{2x-y}-\frac{4x^2}{4x^2+4xy+y^2}\right):\left(\frac{2x}{4x^2-y^2}+\frac{1}{y-2x}\right)\)
\(=\left(\frac{2x}{2x-y}-\frac{4x^2}{\left(2x+y\right)^2}\right):\left(\frac{2x}{\left(2x-y\right)\left(2x+y\right)}-\frac{1}{2x-y}\right)\)
\(=\left(\frac{2x\left(2x+y\right)^2-4x^2\left(2x-y\right)}{\left(2x-y\right)\left(2x+y\right)^2}\right):\left(\frac{2x-\left(2x+y\right)}{\left(2x-y\right)\left(2x+y\right)}\right)\)
\(=\left(\frac{8x^3+8x^2y+2xy^2-8x^3+4x^2y}{\left(2x-y\right)\left(2x+y\right)^2}\right):\left(\frac{-y}{\left(2x-y\right)\left(2x+y\right)}\right)\)
\(=-\left(\frac{12x^2y+xy^2}{2x+y}\right)=\frac{-12x^2y-xy^2}{2x+y}\)
29 tháng 7 2021
\(\dfrac{11x}{2x-3}+\dfrac{x-18}{2x-3}\left(ĐKXĐ:x\ne\dfrac{3}{2}\right)\\ =\dfrac{11x+x-18}{2x-3}\\ =\dfrac{12x-18}{2x-3}\\ =\dfrac{6\left(2x-3\right)}{2x-3}\\ =6\)
\(\dfrac{2x+12}{4x^2-9}+\dfrac{2x+5}{4x-6}\left(ĐKXĐ:x\ne\dfrac{3}{2};x\ne\dfrac{-3}{2}\right)\\ =\dfrac{2x+12}{\left(2x-3\right)\left(2x+3\right)}+\dfrac{2x+5}{2\left(2x-3\right)}\\ =\dfrac{4x+24}{2\left(2x-3\right)\left(2x+3\right)}+\dfrac{\left(2x+5\right)\left(2x+3\right)}{2\left(2x-3\right)\left(2x+3\right)}\\ =\dfrac{4x+24+4x^2+6x+10x+15}{2\left(2x-3\right)\left(2x+3\right)}\\ =\dfrac{4x^2+20x+39}{2\left(2x-3\right)\left(2x+3\right)}\)
\(\dfrac{x}{2x+1}+\dfrac{-1}{4x^2-1}+\dfrac{2-x}{2x-1}\left(ĐKXĐ:x\ne\dfrac{1}{2};x\ne\dfrac{-1}{2}\right)\\ =\dfrac{x\left(2x-1\right)-1+\left(2-x\right)\left(2x+1\right)}{\left(2x+1\right)\left(2x-1\right)}\\ =\dfrac{2x^2-x-1+4x+2-2x^2-x}{\left(2x-1\right)\left(2x+1\right)}\\ =\dfrac{2x+1}{\left(2x+1\right)\left(2x-1\right)}\\ =\dfrac{1}{2x-1}\)
HA
1
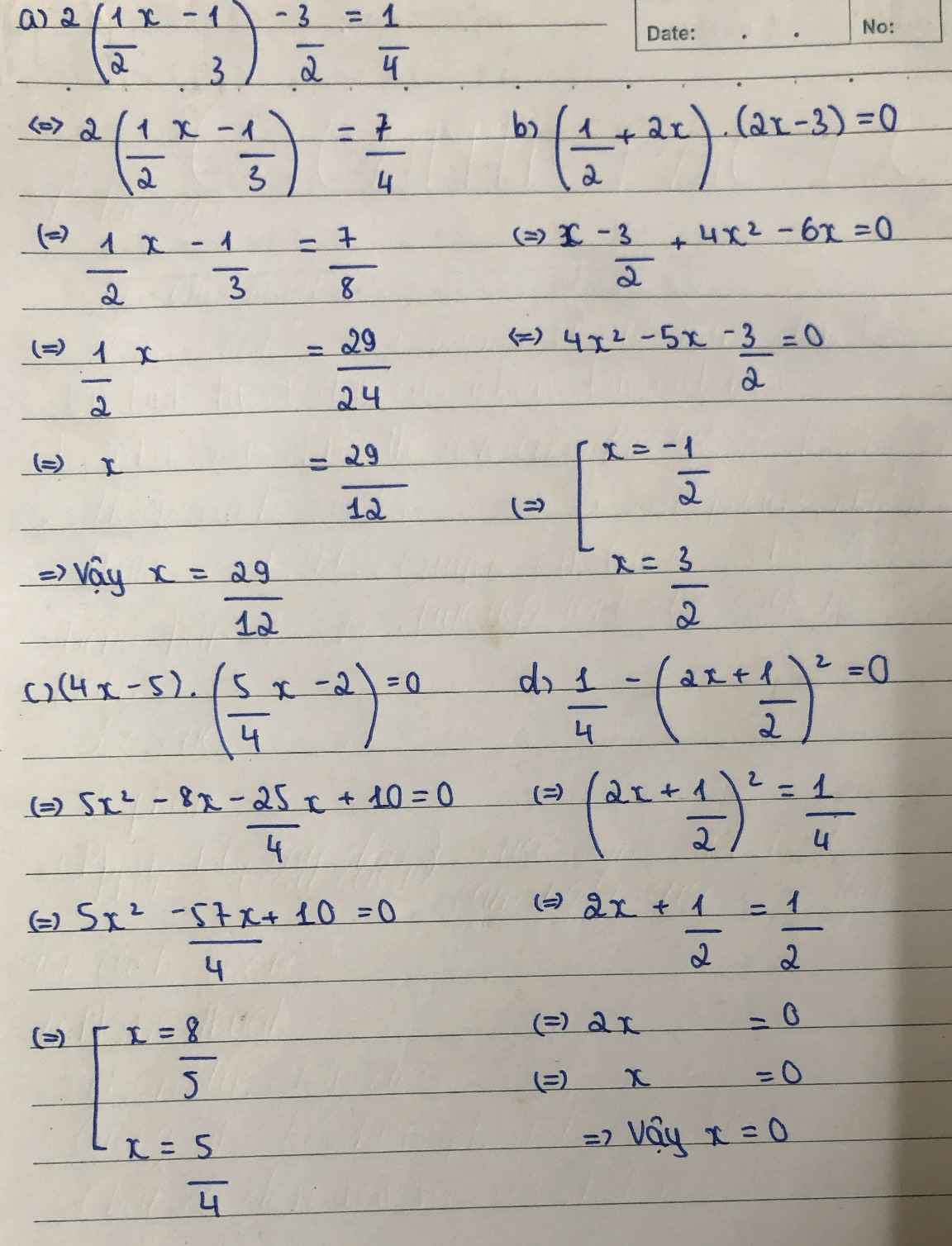
(1-2x)^2+(2-4x)*(1+2x)+(1+2x)^2
= 2^2