\(N=\left(2x+1\right)^2-2\left(4x^2-1\right)+\left(2x-1\right)^2\) . Tính N với \(x=2017^{8^9}\)
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
8 tháng 9 2023
Bạn xem lại đề nhé.
a) \(A=x^2+5y^2+2xy-4x-8y+2015\)
\(A=x^2-4x+4-2y\left(x-2\right)+y^2+2011+4y^2\)
\(A=\left(x-2\right)^2-2y\left(x-2\right)+y^2+2011+4y^2\)
\(A=\left(x-2-y\right)^2+4y^2+2011\)
Vì \(\left(x-y-2\right)^2\ge0;4y^2\ge0\)
\(\Rightarrow A_{min}=2011\)
Dấu bằng xảy ra : \(\Leftrightarrow\left\{{}\begin{matrix}x-y-2=0\\4y^2=0\end{matrix}\right.\Leftrightarrow}\left\{{}\begin{matrix}x=2\\y=0\end{matrix}\right.\)
H9
HT.Phong (9A5)
CTVHS
11 tháng 7 2023
\(M=\left(7-2x\right)\left(4x^2+14x+49\right)-\left(64-8x^3\right)\)
\(M=\left(7-2x\right)\left[\left(2x\right)^2+2x\cdot7+7^2\right]-\left(64-8x^3\right)\)
\(M=\left[7^3-\left(2x\right)^3\right]-\left(64-8x^3\right)\)
\(M=343-8x^3-64+8x^3\)
\(M=279\)
Vậy M có giá trị 279 với mọi x
\(P=\left(2x-1\right)\left(4x^2-2x+1\right)-\left(1-2x\right)\left(1+2x+4x^2\right)\)
\(P=8x^3-4x^2+2x-4x^2+2x-1-1+8x^3\)
\(P=16x^3-8x^2+4x-2\)
Thay \(x=10\) vào P ta có:
\(P=16\cdot10^3-8\cdot10^2+4\cdot10-2=15238\)
Vậy P có giá trị 15238 tại x=10
10 tháng 7 2023
a: M=343-8x^3-64+8x^3=279
b: P=8x^3-4x^2+2x-4x^2+2x-1-1+8x^3
=16x^3-8x^2+4x-2
=16*10^3-8*10^2+4*10-2=15238
29 tháng 10 2017
Áp suất là đơn vị của áp lực trên bề mặt của một diện tích bị ép 
29 tháng 10 2017
a) ( x2 - 1)2 - x( x2 - 1) - 2x2
Đặt : x2 - 1 = a , ta có :
a2 - ax - 2x2
= a2 + ax - 2ax - 2x2
= a( a + x) -2x( a + x)
= ( a + x)( a - 2x)
Thay , x2 - 1 = a , ta có :
( x2 - 1 + x)( x2 - 1 - 2x)
b) ( x2 + 4x + 8)2 + 3x( x2 + 4x + 8) + 2x2
Đặt : x2 + 4x + 8 = b, ta có :
b2 + 3bx + 2x2
= b2 + bx + 2bx + 2x2
= b( b + x) + 2x( b + x)
= ( b + x)( b + 2x)
Thay x2 + 4x + 8 = b, ta có :
( x2 + 4x + 8 + x)( x2 + 4x + 8 + 2x)
= ( x2 + 5x + 8)( x2 + 6x + 8)
Chúc bn hok tốt
2 tháng 6 2022
a: \(\left[\dfrac{1}{2}x^2\left(2x-1\right)^m-\dfrac{1}{2}x^{m+2}\right]:\dfrac{1}{2}x^2=0\)
\(\Leftrightarrow\left(2x-1\right)^m-x^m=0\)
\(\Leftrightarrow\left(2x-1\right)^m=x^m\)
=>2x-1=x
=>x=1
b: \(\left(2x-3\right)^8=\left(2x-3\right)^6\)
\(\Leftrightarrow\left(2x-3\right)^6\cdot\left(2x-4\right)\left(2x-2\right)=0\)
hay \(x\in\left\{\dfrac{3}{2};2;1\right\}\)
c: \(\Leftrightarrow4x^2-4x+1+y^2-\dfrac{2}{3}y+\dfrac{1}{9}+\dfrac{6}{9}=0\)
\(\Leftrightarrow\left(2x-1\right)^2+\left(y-\dfrac{1}{3}\right)^2+\dfrac{6}{9}=0\)(vô lý)
CW
18 tháng 7 2016
a) \(3x\left(2x+1\right)=5\left(2x+1\right)\)
\(3x=5\)
\(x=\frac{5}{3}\)
b) \(\left(3x-8\right)^2=\left(2x-7\right)^2\)
\(3x-8=2x-7\)
\(x=1\)
c) \(\left(4x^2-3x-18\right)^2-\left(4x^2+3x\right)^2=0\)
\(\left(4x^2-3x-18\right)^2=\left(4x^2+3x\right)^2\)
\(4x^2-3x-18=4x^2+3x\)
\(6x=-18\)
\(x=-3\)
d) Sai đề
e) ko bt
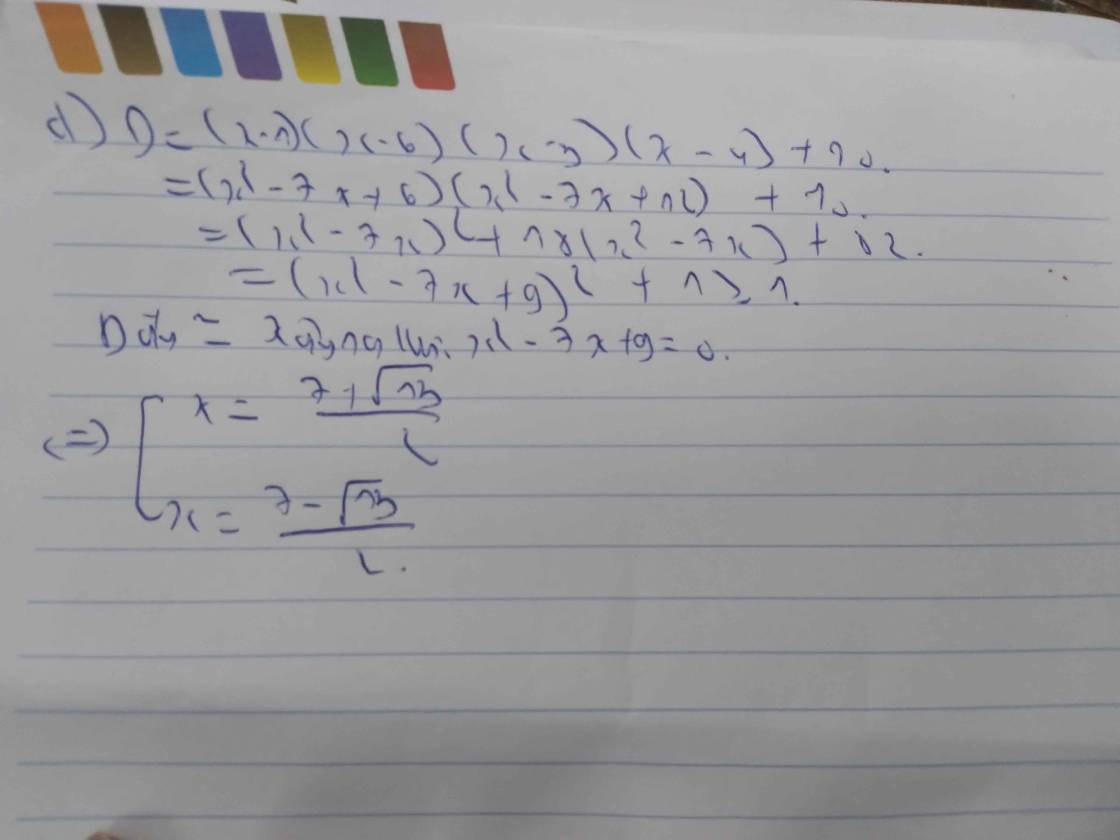
Ta có:
\(N=\left(2x+1\right)^2-2\left(4x^2-1\right)+\left(2x-1\right)^2\)
\(N=\left(2x+1\right)^2-2\left(2x-1\right)\left(2x+1\right)+\left(2x-1\right)^2\)
\(N=\left(2x+1-2x+1\right)^2=2^2=4\)
Vậy với mọi giá trị của x thì biểu thức \(N=4\)
Chúc bạn học tốt!!!
Ta có:
\(N=\left(2x+1\right)^2-2\left(4x^2-1\right)+\left(2x-1\right)^2\)
\(N=\left(2x+1\right)^2-2\left(2x-1\right).\left(2x+1\right)+\left(2x-1\right)^2\)
\(N=\left(2x+1-2x+1\right)^2=2^2=4\)
Vậy vs mọi giá trị của \(x\) thì \(N=4\)