Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
\(\left(4A\right)\\ a,\\ \Leftrightarrow\left[\left(x-2\right)\left(2x+3\right)\right]\left[\left(x-2\right)\left(2x+3\right)\right]=0\\ \Leftrightarrow\left(-x-5\right)\left(3x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}-x-5=0\\3x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-5\\x=\dfrac{-1}{3}\end{matrix}\right.\\ b,\\ \Leftrightarrow\left[3\left(2x+1\right)\right]^2-\left[2\left(x+1\right)\right]^2=0\\ \Leftrightarrow\left[3\left(2x+1\right)-2\left(x+1\right)\right]\left[3\left(2x+1\right)+2\left(x+1\right)\right]=0\\ \Leftrightarrow\left(4x+1\right)\left(8x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}4x+1=0\\8x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-1}{4}\\x=\dfrac{-5}{8}\end{matrix}\right.\\ c,\\ \Leftrightarrow\left[\left(x+1\right)+1\right]^2=0\\ \Leftrightarrow\left(x+1\right)+1=0\\ \Leftrightarrow x+2=0\Rightarrow x=-2\\ d,\\ \Leftrightarrow\left(x-1\right)\left(x-3\right)\left(x+3\right)+\left(x+3\right)=0\\ \Leftrightarrow\left(x+3\right)\left[\left(x-1\right)\left(x+3\right)+1\right]=0\\ \Leftrightarrow\left(x+3\right)\left(x^2-4x+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+3=0\\\left(x+2\right)^2=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-3\\x=-2\end{matrix}\right.\)
\(\left(4B\right)\\ a,\\ \Leftrightarrow49-14x+x^2-4\left(x+25\right)^2=0\\ \Leftrightarrow49-14x+x^2-4x^2-40x-100=0\\ \Leftrightarrow3x^2-54x-51=0\\ \Leftrightarrow-3\left(x^2+18x+17\right)=0\\ \Leftrightarrow\left(x+1\right)\left(x+17\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+1=0\\x+17=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-17\end{matrix}\right.\\ b,\\ \Leftrightarrow4x^2\left(x^2-2x+1\right)-\left(4x^2+4x+1\right)=0\\ \Leftrightarrow x^2-6x=0\\ \Leftrightarrow\left[{}\begin{matrix}x=0\\x-6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=6\end{matrix}\right.\)
\(c,\\ \Leftrightarrow\left(x+1\right)\left(x^2-x+1\right)=\left(x+1\right)\left(2-x\right)=0\\ \Leftrightarrow\left(x+1\right)\left[\left(x^2-x+1\right)-\left(2-x\right)\right]=0\\ \Leftrightarrow\left(x+1\right)\left(x^1-1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x+1=0\\x-1=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=1\\x=-1\end{matrix}\right.\\ d,\\ \Leftrightarrow\left(x-5\right)\left(x+1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x-5=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=-1\end{matrix}\right.\)
`A=4(3^2+1)(3^4+1)...(3^64+1)`
`=>2A=(3^2-1)(3^2+1)(3^4+1)...(3^64+1)`
- Ta có:
`(3^2-1)(3^2+1)=3^4-1`
`(3^4-1)(3^4+1)=3^16-1`
`....`
`(3^64-1)(3^64+1)=3^128-1`
Suy ra `2A=3^128-1=B`
`=>A<B`
a) 2/1 = 8/4
2/8=1/4
8/2=4/1
1/2= 4/8
b) (-2)/(-4)= 3/6
(-2)/3= (-4)/6
6/3= (-4)/(-2)
6/(-4)= 3/(-2)
a) 2/1 = 8/4
2/8=1/4
8/2=4/1
1/2= 4/8
b) (-2)/(-4)= 3/6
(-2)/3= (-4)/6
6/3= (-4)/(-2)
6/(-4)= 3/(-2)
a) \(=\left(x-2\right)^2\)
b) \(=\left(2x+1\right)^2\)
c) \(=\left(4x-3y\right)\left(4x+3y\right)\)
d) \(=\left(4-x-3\right)\left(4+x+3\right)=\left(1-x\right)\left(x+7\right)\)
e) \(=\left(2x-3x+1\right)\left(2x+3x-1\right)=\left(1-x\right)\left(5x-1\right)\)
f) \(=\left(x-y\right)\left(x^2+xy+y^2\right)\)
g) \(=\left(x+3\right)\left(x^2-3x+9\right)\)
h) \(=\left(x+2\right)^3\)
i) \(=\left(1-x\right)^3\)
a: \(x^2-4x+4=\left(x-2\right)^2\)
b: \(4x^2+4x+1=\left(2x+1\right)^2\)
g: \(x^3+27=\left(x+3\right)\left(x^2-3x+9\right)\)
\(8x^3+12x^2+6x+1=\left(2x+1\right)^3\)
\(=\left(2\cdot24.5+1\right)^3=50^3=125000\)
Ta có:
f(x) = x4 – x2 + 6x – 9 = x4 – (x2 – 6x +9) = – (x-3)2
= (x2 –x + 3).(x2 + x - 3)
+ Tam thức x2 – x + 3 có Δ = -11 < 0, a = 1 > 0 nên x2 – x + 3 > 0 với ∀ x ∈ R.
+ Tam thức x2 + x – 3 có hai nghiệm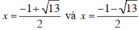 
Ta có bảng xét dấu sau:
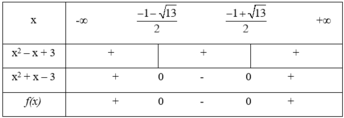
Kết luận:
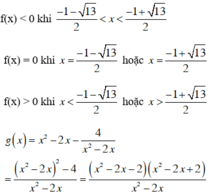
Tam thức x2 - 2x + 2 có Δ = -4 < 0, hệ số a = 1 > 0 nên x2 - 2x + 2 > 0 với ∀ x ∈ R
Tam thức x2 - 2x - 2 có hai nghiệm là x1 = 1 - √3; x2 = 1 + √3.
Tam thức x2 - 2x có hai nghiệm là x1 = 0; x2 = 2
Ta có bảng xét dấu :
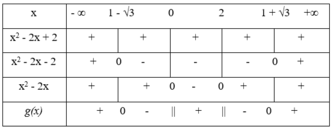
Kết luận : g(x) < 0 khi x ∈ (1 - √3; 0) ∪ (2; 1 + √3)
g(x) = 0 khi x = 1- √3 hoặc x = 1 + √3
g(x) > 0 khi x ∈ (-∞; 1 - √3) ∪ (0; 2) ∪ (1 + √3; +∞)
g(x) không xác định khi x = 0 và x = 2.
\(B=x^3-3x^2+3x\)
\(=x^3-3x^21+3x1^2-1^3+1\)
\(=\left(x-1\right)^3+1\)
thay x=11 vào P ta đc:
\(B=\left(11-1\right)^3+1=1001\)
Vậy B=1001
192=(20−1)2=202−2.20.1+12=400−40+1=361
a)
19^2=(20−1)^2=20^2−2.20.1+1^2=400−40+1=361
28^2=(30−2)^2=30^2−2.30.2+2^2=900−120+4=784
81^2=(80+1)^2=80^2+2.80.1+1^2=6400+160+1=6561
91^2=(90+1)^2=90^2+2.90.1+1^2=8100+180+1=8281