Tìm GTLN ( hoặc GTNN ) của biểu thức sau :
\(D=\dfrac{x^2}{x-2}.\left(\dfrac{x^2+4}{x}-4\right)+3\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: \(A=\dfrac{x-2-2x-4+x}{\left(x-2\right)\left(x+2\right)}\cdot\dfrac{-\left(x-2\right)\left(x+1\right)}{6\left(x+2\right)}\)
\(=\dfrac{-6}{\left(x+2\right)}\cdot\dfrac{-\left(x+1\right)}{6\left(x+2\right)}=\dfrac{\left(x+1\right)}{\left(x+2\right)^2}\)
b: A>0
=>x+1>0
=>x>-1
c: x^2+3x+2=0
=>(x+1)(x+2)=0
=>x=-2(loại) hoặc x=-1(loại)
Do đó: Khi x^2+3x+2=0 thì A ko có giá trị

\(y=x+\dfrac{1}{x}-5\ge2\sqrt{\dfrac{x}{x}}-5=-3\)
\(y_{min}=-3\) khi \(x=1\)
\(y=4x^2+\dfrac{1}{2x}+\dfrac{1}{2x}-4\ge3\sqrt[3]{\dfrac{4x^2}{2x.2x}}-4=-1\)
\(y_{min}=-1\) khi \(x=\dfrac{1}{2}\)
\(y=x+\dfrac{4}{x}\Rightarrow y'=1-\dfrac{4}{x^2}=0\Rightarrow x=-2\)
\(y\left(-2\right)=-4\Rightarrow\max\limits_{x>0}y=-4\) khi \(x=-2\)

B1: ĐXXĐ: \(x\ne\pm2;x\ne-1\)
\(=\left(\dfrac{x-2}{\left(x+2\right)\left(x-2\right)}-\dfrac{2\left(x+2\right)}{\left(x+2\right)\left(x-2\right)}+\dfrac{x}{\left(x+2\right)\left(x-2\right)}\right):\dfrac{-6\left(x+2\right)}{\left(x-2\right)\left(x+1\right)}\)
\(=\left(\dfrac{x-2-2x-2+x}{\left(x+2\right)\left(x-2\right)}\right):\dfrac{-6\left(x+2\right)}{\left(x-2\right)\left(x+1\right)}\)
\(=\dfrac{-4}{\left(x+2\right)\left(x-2\right)}:\dfrac{-6\left(x+2\right)}{\left(x-2\right)\left(x+1\right)}\)
\(=\dfrac{-4}{\left(x+2\right)\left(x-2\right)}.\dfrac{\left(x-2\right)\left(x+1\right)}{-6\left(x+2\right)}=\dfrac{2\left(x+1\right)}{3\left(x+2\right)^2}\)
b, \(A=\dfrac{2\left(x+1\right)}{3\left(x+2\right)^2}>0\)
\(\Leftrightarrow2x+2>0\) (vì \(3\left(x+2\right)^2\ge0\forall x\))
\(\Leftrightarrow x>-1\).
-Vậy \(x\in\left\{x\in Rlx>-1;x\ne2\right\}\) thì \(A>0\).

a: ĐKXĐ: x<>1; x<>2; x<>3
\(K=\left(\dfrac{x^2}{\left(x-2\right)\left(x-3\right)}+\dfrac{x^2}{\left(x-1\right)\left(x-2\right)}\right)\cdot\dfrac{\left(x-1\right)\left(x-3\right)}{x^4+2x^2+1-x^2}\)
\(=\dfrac{x^3-x^2+x^3-3x^2}{\left(x-2\right)\left(x-3\right)\left(x-1\right)}\cdot\dfrac{\left(x-1\right)\left(x-3\right)}{\left(x^2+1+x\right)\left(x^2+1-x\right)}\)
\(=\dfrac{2x^3-4x^2}{\left(x-2\right)}\cdot\dfrac{1}{\left(x^2+x+1\right)\left(x^2-x+1\right)}\)
\(=\dfrac{2x^2\left(x-2\right)}{\left(x-2\right)\left(x^4+x^2+1\right)}=\dfrac{2x^2}{x^4+x^2+1}\)
b:
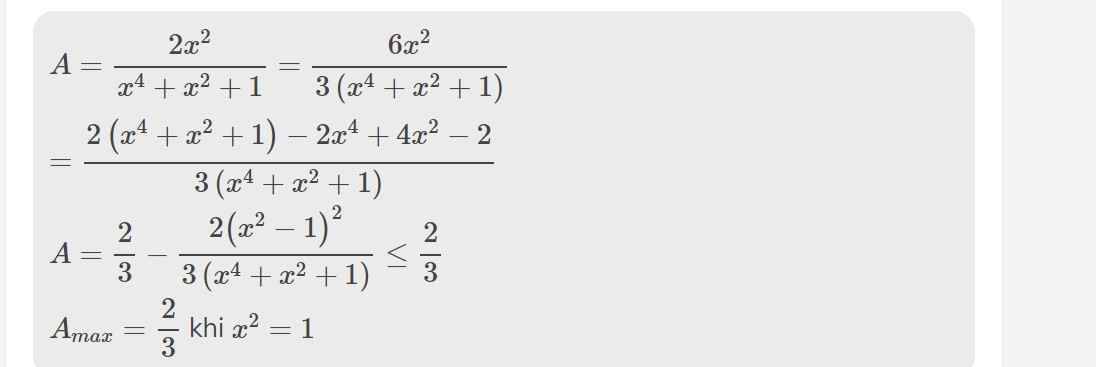

\(D=\left|x+\dfrac{1}{2}\right|+\left|x+\dfrac{1}{3}\right|+\left|x+\dfrac{1}{4}\right|\)
\(=\left|x+\dfrac{1}{2}\right|+\left|x+\dfrac{1}{3}\right|+\left|-\left(x+\dfrac{1}{4}\right)\right|\)
\(=\left|x+\dfrac{1}{2}\right|+\left|x+\dfrac{1}{3}\right|+\left|-x-\dfrac{1}{4}\right|\)
\(\ge x+\dfrac{1}{2}+0-x-\dfrac{1}{4}=\dfrac{1}{4}\)
Đẳng thức xảy ra khi \(x=-\dfrac{1}{3}\)
Vậy với \(x=-\dfrac{1}{3}\) thì \(D_{Min}=\dfrac{1}{4}\)
Ta có : | x + 1/2 | > hoặc = 0
| x + 1/3 | > hoặc = 0
| x + 1/4 | > hoặc = 0
=> D = | x + 1/2 | + | x + 1/3 | + | x + 1/4 | > hoặc = 0
Dấu " = " xảy ra khi D = 0
Vậy GTNN của biểu thức D là 0

Bạn tham khảo lời giải tại đây:
Tìm GTLN của biểu thức: \(A=\left(\dfrac{x^2}{x^2-3x 2} \dfrac{x^2}{x^2-5x 6}\right):\dfrac{x^4 x^2 1}{x^2-4x 3}\) - Hoc24
\(D=\dfrac{x^2}{x-2}\left(\dfrac{x^2+4-4x}{x}\right)+3\)
\(D=\dfrac{x^2}{x-2}\dfrac{\left(x-2\right)^2}{x}+3\)
\(D=x\left(x-2\right)+3\)
\(D=x^2-2x+1+2\)
\(D=\left(x-1\right)^2+2\ge2\)
Dấu"=" xảy ra \(\Leftrightarrow\left(x-1\right)^2=0\)
\(\Leftrightarrow x=1\)
Vậy MinD là 2 \(\Leftrightarrow x=1\)