Ai giúp em câu 13 với ạ :>
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




Cu không phản ứng với H2SO4 loãng.
\(Fe + H_2SO_4 \to FeSO_4 + H_2\\ n_{Fe} = n_{H_2} = \dfrac{2,24}{22,4} = 0,1(mol)\\ \%m_{Fe} =\dfrac{0,1.56}{10}.100\% = 56\%\)
Đáp án B

Câu 13:
ΔABC vuông tại A
=>\(AB^2+AC^2=BC^2\)
=>\(BC^2=6^2+8^2=100\)
=>\(BC=10\left(cm\right)\)
Xét ΔABC vuông tại A có AH là đường cao
nên \(AH\cdot BC=AB\cdot AC\)
=>\(AH\cdot10=6\cdot8=48\)
=>AH=48/10=4,8(cm)
Xét ΔABC vuông tại A có AH là đường cao
nên \(\left\{{}\begin{matrix}AB^2=BH\cdot BC\\AC^2=CH\cdot BC\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}BH=\dfrac{6^2}{10}=3,6\left(cm\right)\\CH=\dfrac{8^2}{10}=6,4\left(cm\right)\end{matrix}\right.\)
Xét ΔHAB vuông tại H có HM là đường cao
nên \(BM\cdot BA=BH^2\)
=>\(BM\cdot6=3,6^2\)
=>BM=2,16(cm)
Xét ΔHAC vuông tại H có HN là đường cao
nên \(AN\cdot AC=AH^2\)
=>\(AN\cdot8=4,8^2\)
=>AN=2,88(cm)
ΔABN vuông tại A
=>\(AB^2+AN^2=BN^2\)
=>\(BN^2=2.88^2+6^2=44,2944\)
=>\(BN=\sqrt{44,2944}=\dfrac{6\sqrt{769}}{25}\left(cm\right)\)
Xét tứ giác AMHN có \(\widehat{AMH}=\widehat{ANH}=\widehat{MAN}=90^0\)
nên AMHN là hình chữ nhật
=>AH=MN=4,8(cm)
Xét ΔMBN có \(cosBMN=\dfrac{MB^2+MN^2-NB^2}{2\cdot MB\cdot MN}\)
\(=\dfrac{4,8^2+2,16^2-\dfrac{27684}{625}}{2\cdot4,8\cdot2,16}=\dfrac{-10368}{625}:\dfrac{2592}{125}=-\dfrac{4}{5}\)
=>\(sinBMN=\sqrt{1-\left(-\dfrac{4}{5}\right)^2}=\dfrac{3}{5}\)
Xét ΔBMN có \(\dfrac{NB}{sinBMN}=2R\)
=>\(2R=\dfrac{6\sqrt{769}}{25}:\dfrac{3}{5}=\dfrac{6\sqrt{769}}{25}\cdot\dfrac{5}{3}=\dfrac{2}{5}\sqrt{769}\)
=>\(R=\dfrac{\sqrt{769}}{5}\)
=>Chọn A

Câu 7:
a, \(Fe+H_2SO_4\rightarrow FeSO_4+H_2\)
\(CuO+H_2SO_4\rightarrow CuSO_4+H_2O\)
b, \(n_{H_2}=\dfrac{2,24}{22,4}=0,1\left(mol\right)\)
Theo PT: \(n_{Fe}=n_{H_2}=0,1\left(mol\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\%m_{Fe}=\dfrac{0,1.56}{10}.100\%=56\%\\\%m_{CuO}=44\%\end{matrix}\right.\)
c, \(n_{CuO}=\dfrac{10-0,1.56}{80}=0,055\left(mol\right)\)
Theo PT: \(n_{H_2SO_4}=n_{Fe}+n_{CuO}=0,155\left(mol\right)\)
\(\Rightarrow C\%_{H_2SO_4}=\dfrac{0,155.98}{100}.100\%=15,19\%\)
d, Theo PT: \(\left\{{}\begin{matrix}n_{FeSO_4}=n_{Fe}=0,1\left(mol\right)\\n_{CuSO_4}=n_{CuO}=0,055\left(mol\right)\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}m_{FeSO_4}=0,1.152=15,2\left(g\right)\\m_{CuSO_4}=0,055.160=8,8\left(g\right)\end{matrix}\right.\)
Câu 8:
a, \(CuCO_3+2HCl\rightarrow CuCl_2+CO_2+H_2O\)
b, \(n_{CO_2}=\dfrac{3,36}{22,4}=0,15\left(mol\right)\)
Theo PT: \(n_{CuCO_3}=n_{CO_2}=0,15\left(mol\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\%m_{CuCO_3}=\dfrac{0,15.124}{20}.100\%=93\%\\\%m_{CuCl_2}=7\%\end{matrix}\right.\)
c, \(n_{HCl}=2n_{CO_2}=0,3\left(mol\right)\)
\(\Rightarrow C_{M_{HCl}}=\dfrac{0,3}{0,2}=1,5\left(M\right)\)

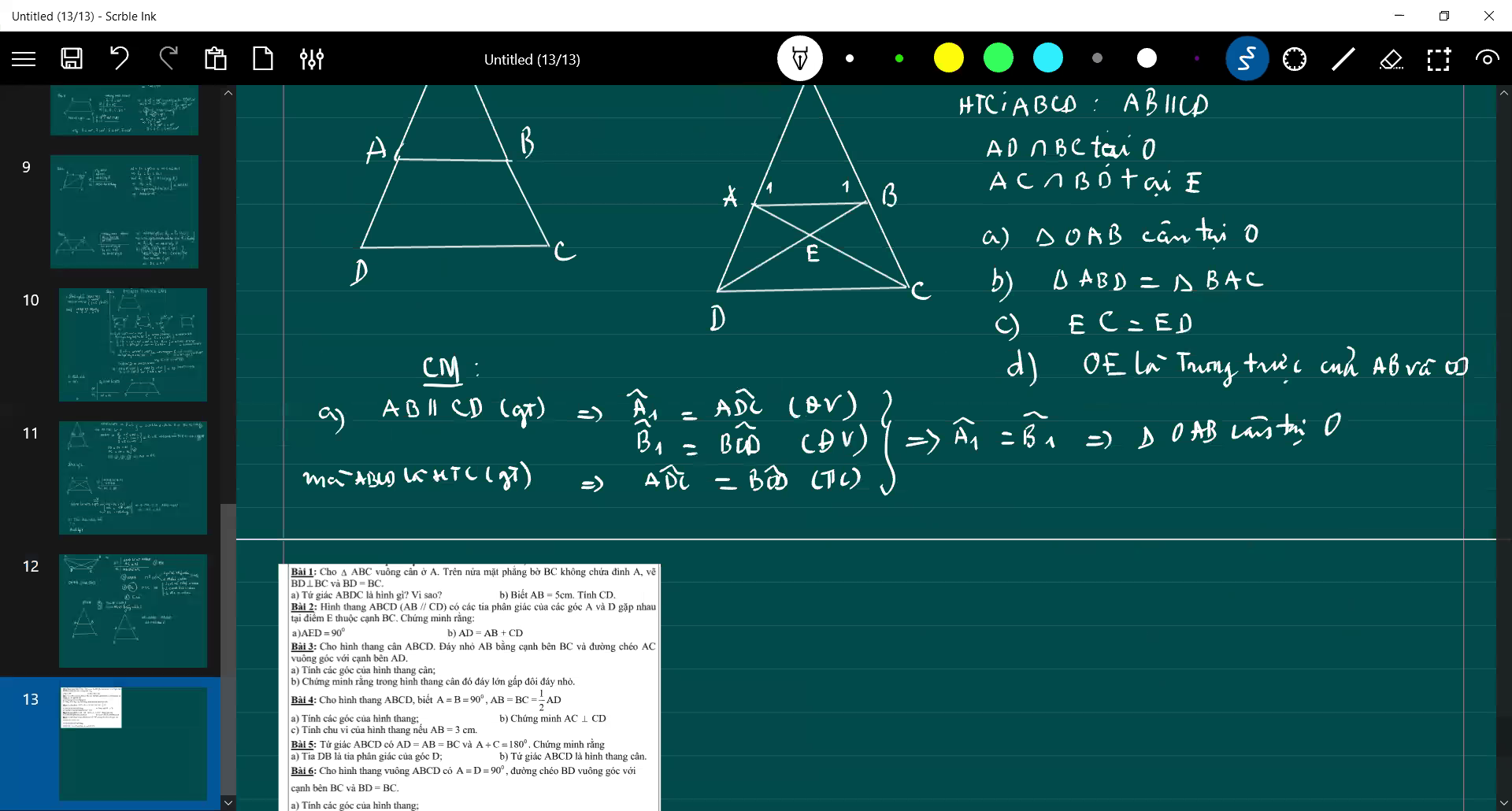
b: Xét ΔABD và ΔBAC có
BA chung
BD=AC
AD=BC
Do đó: ΔABD=ΔBAC
c: ta có: EA+EC=AC
EB+ED=BD
mà AC=BD
và EA=EB
nên EC=ED

a: \(f\left(x\right)=x^4\left(-7-2\right)+2x^3+x^2\left(3-6\right)+x\left(-5+8\right)+6-7\)
\(=-9x^4+2x^3-3x^2+3x-1\)
\(g\left(x\right)=3x^4-2x^3+4x^2-7x+4\)
\(h\left(x\right)=-9x^4-2x^3+5x^2+6x-5\)
b: Bậc của f(x) là 4
Hệ số tự do của f(x) là -1
Hệ số cao nhất là -9
Bậc của g(x) là 4
Hệ số tự do là 4
Hệ số cao nhất là 3
Bậc của h(x) là 4
Hệ số tự do là -5
Hệ số cao nhất là -9

5x + 13 ⋮ 2x + 1
=> 5x + 2,5 + 11,5 ⋮ 2x + 1
=> 2,5(2x + 1) + 11,5 ⋮ 2x + 1
=> 11,5 ⋮ 2x + 1
=> 23 ⋮ 2x + 1
=> ...
=>5x+13chia hết cho 2x+1
ta có : 2(5x+13) chia hết 2x+1
5(2x+1) chia hết 2x+1
=>10x+26 chia hết 2x+1
10x +5 chia hết 2x+1
=>[(10x+26)-(10x+5)]chia hết 2x+1
=>21chia hết 2x+1 hay 2x +1 thược Ư(21) =(1 ;3;7;21;-1;-3;-7;-21)
ta có bảng:
| 2x+1 | 1 | 3 | 7 | -1 | -3 | -7 | 21 | -21 |
| x | 0 | 1 | 3 | -1 | -2 | -4 | 10 | -11 |
| nhận xét | chọn | chọn | chọn | chọn | chọn | chọn | chọn | chọn |
vậy x thuộc {0;1;3;-1;-2;-4;10;-11}



 câu 13, 14, 15 ạ giúp em với
câu 13, 14, 15 ạ giúp em với

...