
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(f\left(x\right)=\left(m^2-1\right)x^2-8mx+9-m^2\ge0\)
\(TH1:m^2-1=0\Rightarrow m=\pm1\)
\(m=1\Rightarrow bpt\Leftrightarrow-8x+8\ge0\Leftrightarrow x\le1\left(loại\right)\)
\(m=-1\Rightarrow bpt\Leftrightarrow8x+8\ge0\Leftrightarrow x\ge-1\left(tm\right)\)
\(TH2:m^2-1>0\Leftrightarrow\left[{}\begin{matrix}m>1\\m< -1\end{matrix}\right.\)
\(\Delta=\left(8m\right)^2-4\left(9-m^2\right)\left(m^2-1\right)< 0\Leftrightarrow4x^4+24x^2+36=\left(2x^2\right)^2+2.2x^2.6+6^2=\left(2x^2+6\right)^2>0\left(\forall m\right)\)
\(\Rightarrow x1< x2\le0\Leftrightarrow\)\(\left\{{}\begin{matrix}x1x2\ge0\\x1+x2< 0\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}9-m^2\ge0\\8m< 0\end{matrix}\right.\)
\(\Leftrightarrow-3\le m< 0\Rightarrow-3\le m< -1\)
\(TH3:m^2-1< 0\Leftrightarrow-1< m< 1\)
\(f\left(x\right)\ge0\Leftrightarrow\Delta\le0\Leftrightarrow\left(2x^2+6\right)^2\le0\left(vô-lí\right)\)
\(\Rightarrow-3\le m\le-1\)

\(4x^2-12x+6\left(x-3\right)=0\\ 4x\left(x-3\right)+6\left(x-3\right)=0\\ \left(x-3\right)\left(4x+6\right)=0\\ \left[{}\begin{matrix}x-3=0\\4x+6=0\end{matrix}\right.\left[{}\begin{matrix}x=3\\x=-\dfrac{3}{2}\end{matrix}\right.\)

Ta có: chân thấp là tính trạng lặn, chân cao là tính trạng trội
Quy ước: A- cao, a- thấp
Bò mẹ chân thấp : aa
Để đẻ được bò con chân cao thì bò bố phải cho giao tử A
Vậy bò bố có KG : AA hoặc Aa
P: AA cao x aa thấp
G: A a
F1: 100% Aa cao
P: Aa cao x aa thấp
G: 1A:1a a
F1: 1Aa: 1aa
1 cao : 1 thấp

1 Nga enjoys collecting coins in her free time
2 There are 54 ethnic groups in VN
3 Sitting in front of a computer all day can cause health problems
4 Some ethnic minoties in the mountains still keep their traditional ways of life

Ta có :/x-3/=/x+1-4/\(\le\)/x+1/+/4/ (bđt về GTTĐ)
nên M\(\le4\)
dấu = xảy ra \(\Leftrightarrow\left(x+1\right).\left(-4\right)\ge0\)
\(\Leftrightarrow x+1\le0\)
\(\Leftrightarrow x\le-1\)
vậy.....
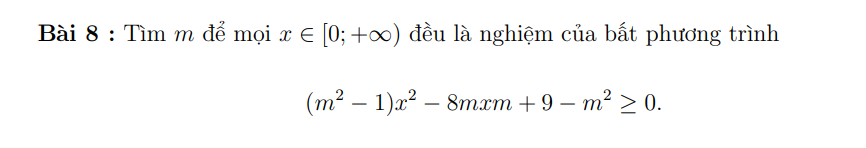

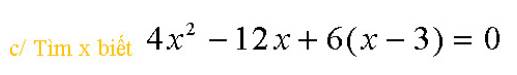

Điều kiện xác định của hàm số đã cho là:
\(1-sinx>0\Leftrightarrow sinx< 1\).
Ta có hàm \(sinx\le1\)nên điều kiện xác định tương đương với \(sinx\ne1\)
\(\Leftrightarrow x\ne\frac{\pi}{2}+k2\pi,\left(k\inℤ\right)\).
Tập xác định: \(D=ℝ\backslash\left\{\frac{\pi}{2}+k2\pi,k\inℤ\right\}\).